Probabilistic Graphical Models 1: Representation-Week1-reasoning-patterns

Posted 2020-08-29 karis

tags:

篇首语：本文由小常识网(cha138.com)小编为大家整理，主要介绍了Probabilistic Graphical Models 1: Representation-Week1-reasoning-patterns相关的知识，希望对你有一定的参考价值。

课程链接：https://www.coursera.org/learn/probabilistic-graphical-models/lecture/KMjHs/reasoning-patterns

原CPD如下：

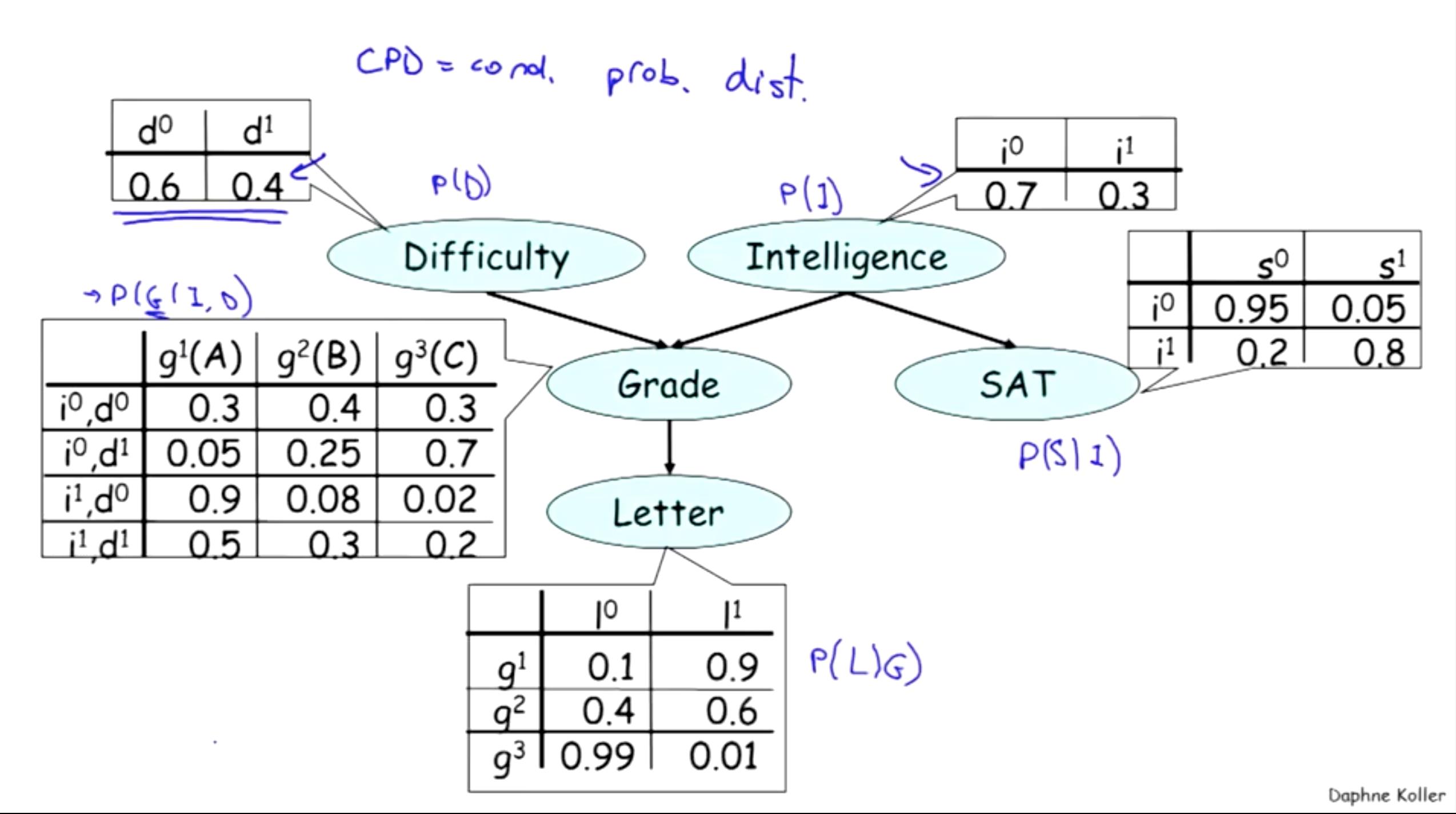
计算：

p(d1|g3) = p(d1, g3) / p(g3)

p(d1, g3) = p(g3, d1) = p(g3|d1, i0) * p(d1, i0) + p(g3|d1, i1) * p(d1, i1)

　　　　　　　　　　　 = 0.7*0.4*0.7 + 0.2*0.4*0.3 约等于0.22

p(g3) = p(g3|d1, i0) * p(d1, i0) + p(g3|d1, i1) * p(d1, i1) +

　　　　 p(g3|d0, i0) * p(d0, i0) + p(g3|d0, i1) * p(d0, i1)

　　　=0.7*0.4*0.7 + 0.2*0.4*0.3 +

　　　　0.3*0.6*0.7 + 0.02*0.68*0.3

　　约等于0.35

p(d1|g3) =0.22／0.35 约等于0.6286

p(i1|g3) = p(i1, g3) / p(g3)

p(i1, g3) = p(g3| i1, d0) * p(i1, d0) + p(g3| i1, d1) * p(i1, d1)

　　　　 = 0.02 * 0.3 * 0.6 + 0.2 * 0.3 * 0.4

　　　　 = 0.0276

p(i1|g3) = 0.0276/0.35 = 0.079

p(i1 | g3, d1) = p(i1, g3, d1) / p(g3, d1)

p(i1, g3, d1)= p(g3| i1, d1) * p(i1, d1) = 0.2 * 0.4 * 0.3

p(i1 | g3, d1) =0.2 * 0.4 * 0.3/0.22 = 0.1091

以上是关于Probabilistic Graphical Models 1: Representation-Week1-reasoning-patterns的主要内容，如果未能解决你的问题，请参考以下文章