HDU 5794：A Simple Chess（Lucas + DP）

Posted 2020-08-05 Shadowdsp

题目链接：http://acm.split.hdu.edu.cn/showproblem.php?pid=5794

题意：让一个棋子从（1,1）走到（n，m），要求像马一样走日字型并只能往右下角走。里面还有r个障碍点不能经过或者到达，问有多少种走法可以走到（n，m）。

思路：画个图可以发现走的点像一个斜着的杨辉三角。所以可以得到一个从点 i 走到点 j 的路径数是一个组合数。 

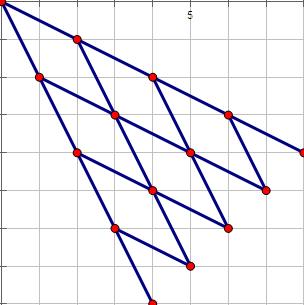大概就是长这样，杨辉三角的每个点的数如下。

1

1       1

1      2      1

1       3      3      1

1      4       6      4      1

1       5      10      10      5      1

1      6      15      20      15      6      1

1      7      21      35      35      21      7      1

 

找到规律：路径数为C（在这一步的位置，走过的步数）。走过的步数是当前的点 i 坐标（x，y），（x+y）/3就是步数了。当前的位置是min（x，y）-步数。这里的步数就相当于三角的层数。

首先对全部障碍从小到大进行排序，对于每个障碍 i，求出从（1，1）走到其的路径总数，减去之前的障碍（0 <= j < i）可以走到现在的障碍的路径总数（dp[i] -= dp[j] * 从点 j 走到点 i 的路径数）。组合数的计算要用到Lucas定理进行计算。

  1 #include <cstdio>
  2 #include <cstring>
  3 #include <algorithm>
  4 #include <string>
  5 #include <cmath>
  6 #include <iostream>
  7 #include <stack>
  8 using namespace std;
  9 #define MOD 110119
 10 typedef long long LL;
 11 struct node
 12 {
 13     LL x, y;
 14 }p[115];
 15 LL dp[115];
 16 LL f[MOD+10];
 17 /*
 18 dp[i]一开始表示从(0, 0)走到第i个点的路径数
 19 后面要减去如果前面有障碍,那么会有一部分路径是不能走的
 20 减去的路径数为分别为第j个点(0<=j<i)走到第i个点的路径数*dp[j]
 21 */
 22 
 23 bool cmp(const node &a, const node &b)
 24 {
 25     if(a.x == b.x) return a.y < b.y;
 26     return a.x < b.x;
 27 }
 28 
 29 void biao() //打出阶乘表
 30 {
 31     f[0] = f[1] = 1;
 32     for(int i = 2; i <= MOD; i++) {
 33         f[i] = f[i-1] * i % MOD;
 34     }
 35 }
 36 
 37 LL quick_pow(LL a, LL b)
 38 {
 39     a %= MOD, b %= MOD;
 40     LL ans = 1;
 41     while(b) {
 42         if(b & 1) ans = ans * a % MOD;
 43         a = a * a % MOD;
 44         b >>= 1;
 45     }
 46     return ans;
 47 }
 48 
 49 LL C(LL n, LL m)
 50 {
 51     if(m > n) return 0;
 52     if(m < 0) return 0;
 53     LL ans = 1;
 54     ans = ans * f[n] % MOD * quick_pow(f[m] * f[n-m] % MOD, MOD - 2) % MOD;
 55     return ans;
 56 }
 57 
 58 LL Lucas(LL n, LL m)
 59 {
 60     if(m == 0) return 1;
 61     return C(n % MOD, m % MOD) % MOD * Lucas(n / MOD, m / MOD) % MOD;
 62 }
 63 
 64 int main()
 65 {
 66     LL n, m, r;
 67     int cas = 0;
 68     biao();
 69     while(~scanf("%I64d%I64d%I64d", &n, &m, &r)) {
 70         memset(dp, 0, sizeof(dp));
 71         bool flag = 0;
 72         for(int i = 0; i < r; i++) {
 73             scanf("%I64d%I64d", &p[i].x, &p[i].y);
 74             if(p[i].x == n && p[i].y == m) flag = 1;
 75             p[i].x--, p[i].y--;
 76         }
 77         sort(p, p + r, cmp);
 78         p[r].x = n - 1, p[r].y = m - 1; //把目标点加入
 79         printf("Case #%d: ", ++cas);
 80         if(flag || (p[r].x + p[r].y) % 3 != 0) { //如果障碍在目标点上或者不能走到目标点
 81             puts("0"); continue;
 82         }
 83         for(int i = 0; i <= r; i++) {
 84             if((p[i].x + p[i].y) % 3 == 0) { //如果这个障碍是可以走到的
 85                 LL a = (p[i].x + p[i].y) / 3; //第几层
 86                 LL b = min(p[i].x, p[i].y) - a; //位置
 87                 dp[i] = Lucas(a, b); //类似于杨辉三角的组合数
 88                 for(int j = 0; j < i; j++) {
 89                     if(p[j].y >= p[i].y || p[j].x >= p[i].x) continue; //题目要求只能往右下角走
 90                     LL xx = (p[i].x - p[j].x);
 91                     LL yy = (p[i].y - p[j].y);
 92                     if((xx + yy) % 3 == 0) { //要能够从j点走到i点
 93                         LL aa = (xx + yy) / 3;
 94                         LL bb = min(xx, yy) - aa; //减去可以从j点走到i点的路径数
 95                         dp[i] -= (Lucas(aa, bb) * dp[j]) % MOD;
 96                         dp[i] = (dp[i] + MOD) % MOD;
 97                     }
 98                 }
 99             }
100         }
101         printf("%I64d\\n", dp[r]);
102     }
103     return 0;
104 }