62. Unique Paths

Posted 2020-11-10 lyinfo

62. Unique Paths

A robot is located at the top-left corner of a m x n grid (marked ‘Start‘ in the diagram below).

The robot can only move either down or right at any point in time. The robot is trying to reach the bottom-right corner of the grid (marked ‘Finish‘ in the diagram below).

How many possible unique paths are there?

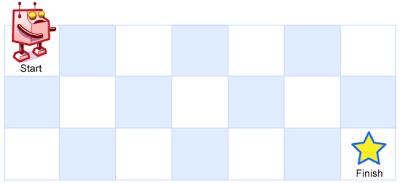
Above is a 7 x 3 grid. How many possible unique paths are there?

Note: m and n will be at most 100.

Example 1:

Input: m = 3, n = 2
Output: 3
Explanation:
From the top-left corner, there are a total of 3 ways to reach the bottom-right corner:
1. Right -> Right -> Down
2. Right -> Down -> Right
3. Down -> Right -> Right

Example 2:

Input: m = 7, n = 3
Output: 28

　　题目要求到达终点的可能路径，在已知长宽的情况下，到达途中Finish，一共要走(m - 1) + ( n - 1) = m + n - 2，其中向右m - 1步，向下n - 1步，很典型的排列组合问题，即在m + n - 2步中选 m - 1个位置向右走，剩下的自然是向下走，得到公式：

　　　　N = C（m + n - 2, m - 1）

　　编码完成组合公式的计算：

    public int uniquePaths(int m, int n) {
        return choose(m + n - 2, m > n ? n - 1 : m - 1);
    }

    public static int choose(int m, int n) {
        int member = 1;
        int temp = n;
        while (temp-- > 0) {
            member *= m--;
        }
        int denominator = 1;
        int start = 1;
        temp = n;
        while (temp-- > 0) {
            denominator *= start++;
        }
        return member / denominator;
    }

　　提交后发现，在case : m = 10 , n = 10时，未通过。想了半天确定解题思路没问题，于是一步步debug，终于发现乘法计算中，超出了int能表示的最大整数！