Useful Decomposition

Posted 2020-11-09 行远山

time limit per test

1 second

memory limit per test

256 megabytes

input

standard input

output

standard output

Ramesses knows a lot about problems involving trees (undirected connected graphs without cycles)!

He created a new useful tree decomposition, but he does not know how to construct it, so he asked you for help!

The decomposition is the splitting the edges of the tree in some simple paths in such a way that each two paths have at least one common vertex. Each edge of the tree should be in exactly one path.

Help Remesses, find such a decomposition of the tree or derermine that there is no such decomposition.

Input

The first line contains a single integer $\mathrm{nn (2\le n\le 1052\le n\le 105)\; the\; number\; of\; nodes\; in\; the\; tree.}$

Each of the next $\mathrm{n?1n???1 lines\; contains\; two\; integers aiai and bibi (1\le ai,bi\le n1\le ai,bi\le n, ai\ne biai\ne bi) —\; the\; edges\; of\; the\; tree.\; It\; is\; guaranteed\; that\; the\; given\; edges\; form\; a\; tree.}$

Output

If there are no decompositions, print the only line containing "No".

Otherwise in the first line print "Yes", and in the second line print the number of paths in the decomposition $\mathrm{mm.}$

Each of the next $\mathrm{mm lines\; should\; contain\; two\; integers uiui, vivi (1\le ui,vi\le n1\le ui,vi\le n, ui\ne viui\ne vi)\; denoting\; that\; one\; of\; the\; paths\; in\; the\; decomposition\; is\; the\; simple\; path\; between\; nodes uiui and vivi.}$

Each pair of paths in the decomposition should have at least one common vertex, and each edge of the tree should be presented in exactly one path. You can print the paths and the ends of each path in arbitrary order.

If there are multiple decompositions, print any.

Examples

input

Copy

4
1 2
2 3
3 4

output

Copy

Yes
1
1 4

input

Copy

6
1 2
2 3
3 4
2 5
3 6

output

Copy

No

input

Copy

5
1 2
1 3
1 4
1 5

output

Copy

Yes
4
1 2
1 3
1 4
1 5

Note

The tree from the first example is shown on the picture below: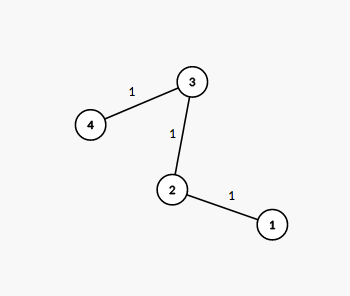The number next to each edge corresponds to the path number in the decomposition. It is easy to see that this decomposition suits the required conditions.

The tree from the second example is shown on the picture below: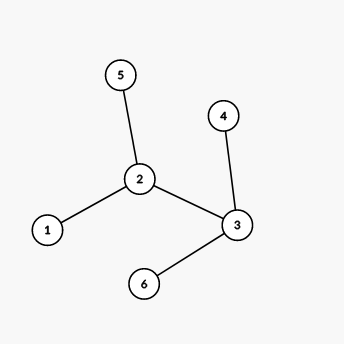We can show that there are no valid decompositions of this tree.

The tree from the third example is shown on the picture below: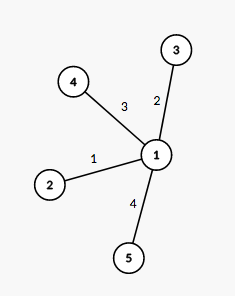The number next to each edge corresponds to the path number in the decomposition. It is easy to see that this decomposition suits the required conditions.

 

题意是给你一颗树，问你能不能分解成若干条路，每条路之间要有公共点，且每条路不能共用一条边，路必须是简单路（不往回走能走通）。

我们可以发现，如果除了根节点外，其他节点有两个及以上子节点，那么必然无法分解。

那么除了根节点，其余节点最多只有一个子节点。

我们选取一个根节点，此根节点必然是节点数最多的，然后将其与没有子节点的节点作为一条路，就可以达到题目要求。

AC代码：

#include <bits/stdc++.h>
#define maxn 100005
using namespace std;
typedef long long ll;
int sizes[maxn];
int main()
{
    int n,i;
    scanf("%d",&n);
    for(i=1;i<=n-1;i++)
    {
        int u,v;
        scanf("%d%d",&u,&v);
        sizes[u]++;
        sizes[v]++;
    }
    int flag=0;
    int sum=-1,num;
    for(i=1;i<=n;i++)
    {
        if(sizes[i]>=sum)
        {
            num=i;
            sum=sizes[i];
        }
    }
    for(i=1;i<=n;i++)
    {
        if(i!=num)
        {
            if(sizes[i]>=3) flag=1;
        }
    }
    if(flag) printf("No\n");
    else
    {
        printf("Yes\n");
        int cnts=0;
        for(i=1;i<=n;i++)
        {
            if(i!=num)

            {if(sizes[i]==1)
            {   cnts++;
            }}
        }
        printf("%d\n",cnts);
        for(i=1;i<=n;i++)
        {
            if(i!=num)
            {
            if(sizes[i]==1)
            {
                printf("%d %d\n",num,i);
            }
            }
        }
    }
    return 0;
}

　　

以上是关于Useful Decomposition的主要内容，如果未能解决你的问题，请参考以下文章

CF981C Useful Decomposition 树 dfs 二十三 *

CF981C Useful Decomposition树/思维

[TIA PORTAL][CONVERT] Convert Char Array to DInt...DInt to Char Array..Useful and easy function(代码片段

seasonal_decomposition 的 resid 函数删除 Python 中的列名

sklearn.decomposition.DicitonaryLearning.fit 中的 y 参数有啥作用？

《Single Image Depth Prediction with Wavelet Decomposition》论文笔记