Educational Codeforces Round 37 E 补图求连通块 bfs+链表优化 F 线段树套路

Posted 2020-10-23 掉血菜鸡煮熟中

Educational Codeforces Round 37

E. Connected Components?

题意：给出的是补图，求原图连通块个数及每个连通块的大小。

tags：原题。。

1】每次选取一个未分配的点，从这个点 bfs。但因为原图太大，我们只能在补图的基础上对原图 bfs。

假设当前点是 u ，如果补图上有边 u -> v ，就把点 v 标记。之后在所有未分配的点中，找没有被标记的点，加入连通块。 注意还要把点 v 的标记取消，因为之后可能还有点会搜到 v 。

2】因为每次要在未分配的点中跑一遍，所以我们要加个链表优化。已经分配的点就从链表中删除。

因为每个点和每条边都只会走一次，复杂度是 O(n+m) 。

//  Edu  37 E
#include<bits/stdc++.h>
using namespace std;
#pragma comment(linker, "/STACK:102400000,102400000")
#define rep(i,a,b) for (int i=a; i<=b; ++i)
#define per(i,b,a) for (int i=b; i>=a; --i)
#define mes(a,b)  memset(a,b,sizeof(a))
#define INF 0x3f3f3f3f
#define MP make_pair
#define PB push_back
#define fi  first
#define se  second
typedef long long ll;
const int N = 200005;

int n, m, pre[N], nex[N], cnt, ans[N];
bool vis1[N], mark[N];
queue< int > q;
vector< int > G[N];
void Delete(int u) {
    nex[pre[u]] = nex[u];  pre[nex[u]] = pre[u];
    ++ans[cnt], mark[u]=true;
}
void bfs(int u)
{
    ++cnt;
    q.push(u);
    Delete(u);
    while(!q.empty())
    {
        u = q.front();  q.pop();
        for(auto to : G[u])
            if(!mark[to]) vis1[to]=true;
        for(int i=nex[0]; i; i=nex[i])
            if(!vis1[i]) q.push(i), Delete(i);
        for(auto to : G[u])
            if(!mark[to]) vis1[to]=false;
    }
}
int main()
{
    scanf("%d%d", &n, &m);
    rep(i,1,n) pre[i]=i-1, nex[i]=i+1;
    pre[1]=0, nex[n]=0, nex[0]=1;
    int u, v;
    rep(i,1,m)
    {
        scanf("%d%d", &u, &v);
        G[u].PB(v);  G[v].PB(u);
    }
    u = 0;
    while(nex[u]) {
        mark[nex[u]] = true;
        bfs(nex[u]);
        u = 0;
    }
    sort(ans+1, ans+1+cnt);
    printf("%d\n", cnt);
    rep(i,1,cnt) printf("%d ", ans[i]);

    return 0;
}

View Code

 

F. SUM and REPLACE

题意：定义 D(n) 为正整数 n 的正因子个数。两种操作：

1. REPLACE l r — for every 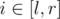replace a_i with D(a_i);
2. SUM l r — calculate .

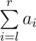tags：一眼线段树，区间更新，区间求和。。一开始想 lazy 标记写，结果发现每次必须更新下去，lazy 没有用。。

其实就是一个老套路，D(n) 下降速度很快，如果 n<=2 ，D(n) = n 。所以我们只要记录一下区间最大值，如果 max <= 2 ，就跳出。

//  Edu37 F
#include<bits/stdc++.h>
using namespace std;
#pragma comment(linker, "/STACK:102400000,102400000")
#define rep(i,a,b) for (int i=a; i<=b; ++i)
#define per(i,b,a) for (int i=b; i>=a; --i)
#define mes(a,b)  memset(a,b,sizeof(a))
#define INF 0x3f3f3f3f
#define MP make_pair
#define PB push_back
#define fi  first
#define se  second
#define  mid  (L+R>>1)
typedef long long ll;
const int N = 1000005, M = 300005;

int n, m, cnt[N];
ll  sum[N<<2], mx[N<<2];
void pushup(int ro) {
    sum[ro] = sum[ro<<1]+sum[ro<<1|1];
    mx[ro] = max(mx[ro<<1], mx[ro<<1|1]);
}
void build(int ro, int L, int R)
{
    if(L==R) {
        scanf("%lld", &mx[ro]);
        sum[ro]=mx[ro];
        return ;
    }
    build(ro<<1, L, mid);
    build(ro<<1|1, mid+1, R);
    pushup(ro);
}
void update(int ro, int L, int R, int l, int r)
{
    if(mx[ro]<=2) return ;
    if(l<=L && R<=r && L==R) {
        sum[ro]=mx[ro]=cnt[sum[ro]];
        return ;
    }
    if(l<=mid) update(ro<<1, L, mid, l, r);
    if(mid<r) update(ro<<1|1, mid+1, R, l, r);
    pushup(ro);
}
ll  query(int ro, int L, int R, int l, int r)
{
    if(l<=L && R<=r) return sum[ro];
    ll  ans = 0;
    if(l<=mid) ans += query(ro<<1, L, mid, l, r);
    if(mid<r) ans += query(ro<<1|1, mid+1, R, l, r);
    return ans;
}
int main()
{
    rep(i,1,N-1)
        for(int j=i; j<N; j+=i)
            ++cnt[j];
    scanf("%d%d", &n, &m);
    build(1, 1, n);
    int t, l, r;
    while(m--)
    {
        scanf("%d%d%d", &t, &l, &r);
        if(t==1) update(1, 1, n, l, r);
        else printf("%lld\n", query(1, 1, n, l, r));
    }

    return 0;
}

View Code