Now imagine you‘d like to buy m kilos of apples. You‘ve asked n supermarkets and got the prices. Find the minimum cost for those apples.
You can assume that there are enough apples in all supermarkets.
The first line contains two positive integers n and m (1 ≤ n ≤ 5 000, 1 ≤ m ≤ 100), denoting that there are n supermarkets and you want to buy m kilos of apples.
The following n lines describe the information of the supermarkets. Each line contains two positive integers a, b (1 ≤ a, b ≤ 100), denoting that in this supermarket, you are supposed to pay a yuan for b kilos of apples.
The only line, denoting the minimum cost for m kilos of apples. Please make sure that the absolute or relative error between your answer and the correct answer won‘t exceed 10 - 6.
Formally, let your answer be x, and the jury‘s answer be y. Your answer is considered correct if 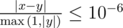 .
.
3 5
1 2
3 4
1 3
1.66666667
2 1
99 100
98 99
0.98989899
In the first sample, you are supposed to buy 5 kilos of apples in supermarket 3. The cost is 5 / 3 yuan.
In the second sample, you are supposed to buy 1 kilo of apples in supermarket 2. The cost is 98 / 99 yuan.
找最便宜的超市买东西即可。


#include <bits/stdc++.h> using namespace std; int main() { int n; double m; scanf("%d%lf",&n,&m); double minx = 0x3f3f3f3f; for(int i = 0; i < n; i++) { double a,b; scanf("%lf%lf",&a,&b); minx = min(minx,a/b); } printf("%lf\n",m*minx); return 0; }
We consider a positive integer perfect, if and only if the sum of its digits is exactly 10. Given a positive integer k, your task is to find the k-th smallest perfect positive integer.
A single line with a positive integer k (1 ≤ k ≤ 10 000).
A single number, denoting the k-th smallest perfect integer.
1
19
2
28
The first perfect integer is 19 and the second one is 28.
暴力打表


#include <bits/stdc++.h> using namespace std; bool calc(int x) { int sum = 0; while(x) { sum+=(x%10); x/=10; } return sum==10; } int main() { vector<int> ans; for(int i = 19; i < 20000000; i++) { if(calc(i)) ans.push_back(i); } //printf("%d\n",ans.size()); int k; scanf("%d",&k); printf("%d\n",ans[k-1]); return 0; }
Suppose that you are in a campus and have to go for classes day by day. As you may see, when you hurry to a classroom, you surprisingly find that many seats there are already occupied. Today you and your friends went for class, and found out that some of the seats were occupied.
The classroom contains n rows of seats and there are m seats in each row. Then the classroom can be represented as an n × m matrix. The character ‘.‘ represents an empty seat, while ‘*‘ means that the seat is occupied. You need to find k consecutive empty seats in the same row or column and arrange those seats for you and your friends. Your task is to find the number of ways to arrange the seats. Two ways are considered different if sets of places that students occupy differs.
The first line contains three positive integers n, m, k (1 ≤ n, m, k ≤ 2 000), where n, m represent the sizes of the classroom and k is the number of consecutive seats you need to find.
Each of the next n lines contains m characters ‘.‘ or ‘*‘. They form a matrix representing the classroom, ‘.‘ denotes an empty seat, and ‘*‘ denotes an occupied seat.
A single number, denoting the number of ways to find k empty seats in the same row or column.
2 3 2
**.
...
3
1 2 2
..
1
3 3 4
.*.
*.*
.*.
0
In the first sample, there are three ways to arrange those seats. You can take the following seats for your arrangement.
- (1, 3), (2, 3)
- (2, 2), (2, 3)
- (2, 1), (2, 2)
k连坐
做的时候把我做傻了,一步三坑。以至于我算重复了。
1 ,行数只有一行
2 ,k = 1
搞得我换了两种写法。第二种好看一点。


#include <bits/stdc++.h> using namespace std; const int maxn = 2005; char maps[maxn][maxn]; int main() { //freopen("in.txt","r",stdin); int n,m,K; scanf("%d%d%d",&n,&m,&K); int ans = 0; for(int i = 0; i < n; i++) scanf("%s",maps[i]); if(K == 1) { for(int i = 0; i < n; i++) { for(int j = 0; j < m; j++) { if(maps[i][j]==‘.‘) ans++; } } printf("%d\n",ans); return 0; } for(int i = 0; i < n; i++) { for(int j = 0; j < m; j++) { if(maps[i][j]==‘.‘) { int cnt = 0; int k; for(k = j; k < m; k++) { if(maps[i][k]==‘.‘) cnt++; else break; } if(cnt>=K) { ans = ans + cnt-K+1; } j = k; } } } if(n!=1) { for(int j = 0; j < m; j++) { for(int i = 0; i < n; i++) { if(maps[i][j]==‘.‘) { int cnt = 0; int k; for(k = i; k < n; k++) { if(maps[k][j]==‘.‘) cnt++; else break; } if(cnt>=K) ans=ans + cnt - K +1; i = k; } } } } printf("%d\n",ans); return 0; }


#include <bits/stdc++.h> using namespace std; const int maxn = 2005; char maps[maxn][maxn]; int main() { //freopen("in.txt","r",stdin); int n,m,K; scanf("%d%d%d",&n,&m,&K); for(int i = 0; i < n; i++) scanf("%s",maps[i]); if(K == 1) { int ans = 0; for(int i = 0; i < n; i++) { for(int j = 0; j < m; j++) { if(maps[i][j]==‘.‘) ans++; } } printf("%d\n",ans); return 0; } int ans = 0; int cnt = 0; int i,j; for(i = 0; i < n; i++) { cnt = 0; for(j = 0; j < m; j++) { if(maps[i][j]==‘*‘||maps[i][j+1]==‘\0‘) { if(maps[i][j]==‘.‘) cnt++; ans += cnt>=K ? cnt - K + 1:0; cnt = 0; continue; } cnt ++; } } if(n!=1) { for(i = 0; i < m; i++) { cnt = 0; for(j = 0; j < n; j++) { if(maps[j][i]==‘*‘||maps[j+1][i]==‘\0‘) { if(maps[j][i]==‘.‘) cnt++; ans += cnt>=K ? cnt - K + 1:0; cnt = 0; continue; } cnt++; } } } printf("%d\n",ans); return 0; }