第45课 递归的思想与应用（下）

Posted 2020-10-06 浅墨浓香

1. 函数调用栈的回顾

 

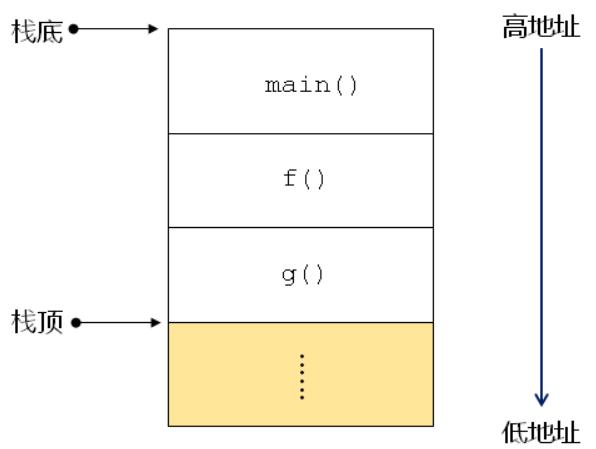
（1）用于保存函数中的实参、局部变量、临时变量等。

（2）从起始地址开始往一个方向增长（如：高地址→低地址）

（3）有一个专用“指针”标识当前已使用内存的“顶部”

（4）当函数调用结束时，栈会恢复到被调用前的状态。可以利用这个时机进行一些的回溯算法的设计。

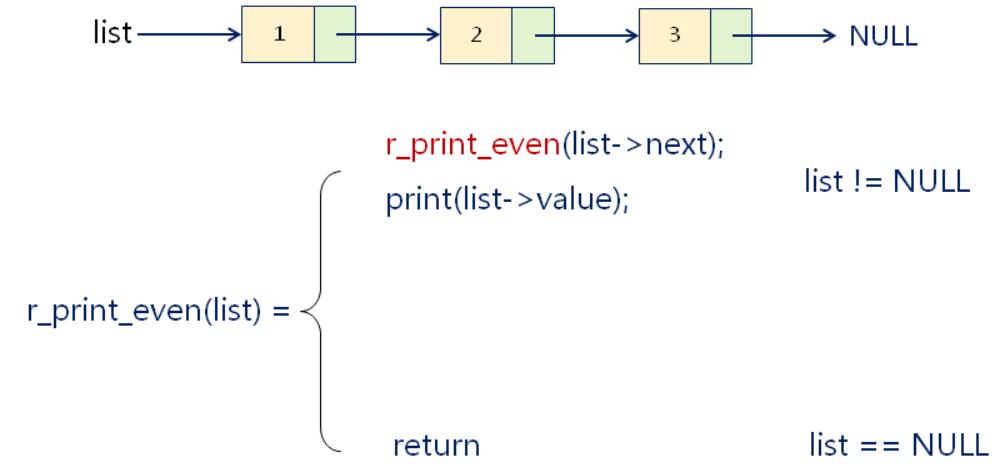
【实例分析】函数调用栈分析：逆序打印单链表中的偶数结点（void r_print_even(Node* list)），见本课后面的源码

2. 回溯求解八皇后问题

（1）回溯算法：实际上一个类似枚举的搜索尝试过程，主要是在搜索尝试过程中寻找问题的解，当发现已不满足求解条件时，就“回溯”返回，尝试别的路径。八皇后问题就是回溯算法的典型，第一步按照顺序放一个皇后，然后第二步符合要求放第2个皇后，如果没有位置符合要求，那么就要改变第一个皇后的位置，再重新放第2个皇后的位置，直到找到符合条件的位置，以此方法放其他皇后…

（2）八皇后问题

 

　　①在一个8×8的国际象棋棋盘上，有8个皇后，每个皇后占一格；

　　②要求皇后间不会出现相互“攻击”的现象（不能有两个皇后处在同一行、 同一列或同一对角线上）

（3）关键数据结构定义

　　①棋盘：二维数组（8 × 8）：0表示位置为空，1表示皇后。

　　②位置：struct Pos; 其中的x字段表示列坐标，y表示行坐标。

　　③方向：

　　　　水平：向左(-1,0)，向右(1,0)

　　　　垂直：向上(0,1)，向下(0,-1)

　　　　对角线：左上(-1,1)，左下(-1,-1)，右上(1,1)，右下(1,-1)

（3）算法思路：

　　①从第一行开始，为皇后找到安全位置，然后跳到下一行，通过递归进行深度搜索。

　　②如果在第n行出现死胡同，当该行为第一行，棋局失败，否则后退到上一行，进行回溯，并从该行的下一列位置继续查找安全位置。

　　③如果在第8行上找到了安全位置，则棋局成功。

【编程实验】八皇后问题的递归解法

#include <iostream>
#include "LinkList.h"

using namespace std;
using namespace DTLib;

struct Node
{
    int value;
    Node* next;
};

//创建无表头结点的单链表（己填充数据）：v结点的值，len链表长度
Node* create_list(int v, int len)
{
    Node* ret = NULL;
    Node* slider = NULL;

    for(int i=0; i<len; i++){
        Node* node = new Node();

        node->value = v++;
        node->next = NULL;

        //每创建好一个节点，slider指向这个节点
        if( slider == NULL ){
            slider = node;
            ret = node;
        }else{
            slider->next = node;
            slider = node;
        }
    }

    return ret;
}

void destroy_list(Node* list)
{
    while(list){
        Node* del = list;
        list = list->next;

        delete del;
    }
}

//打印链表的内容
void print_list(Node* list)
{
    while(list){
        cout << list->value << "->";
        list = list->next;
    }

    cout << "NULL" << endl;
}

//逆序打印单链表中的偶数结点
void r_print_even(Node* list)
{
    if(list != NULL){

        //深度搜索，直到最后一个节点
        r_print_even(list->next);

        //回溯算法设计：上一行递归结束，开始回溯并判断节点的值是否为偶数
        if(list->value % 2 == 0){
            cout << list->value << " ";
        }
    }
}

template<int N>
class QueenSolution : public Object
{
protected:

    struct Pos : public Object
    {
        int x;
        int y;
        Pos(int px = 0, int py = 0):x(px),y(py){}
    };

    int m_chessboard[N][N]; //棋盘: 原点0，0在左下角，x轴正方向向右，y轴正方向向上
    Pos m_direction[3];     //三个方向向量
    LinkList<Pos> m_solution;
    int m_count;

    void init()
    {
        m_count = 0;

        //初始化棋盘
        for(int i=0; i<N; i++){
            for (int j=0; j<N; j++){
                m_chessboard[i][j] = 0;
            }
        }

        //初始化方向矢量
        //左下角
        m_direction[0].x =-1;
        m_direction[0].y =-1;
        //向下
        m_direction[1].x =0;
        m_direction[1].y =-1;
        //右下角
        m_direction[2].x =1;
        m_direction[2].y =-1;
    }

    //打印棋盘内容
    void print()
    {
        typename LinkList<Pos>::iterator iter = m_solution.begin();

        while(iter != m_solution.end()){
            cout << "(" << (*iter).x <<"," <<(*iter).y <<") ";
            iter++;
        }

        cout << endl;

        for(int y=0; y<N; y++){
            for (int x=0; x<N; x++){
                switch (m_chessboard[x][y]) {
                    case 0:cout << " ."; break;
                    case 1:cout << " #"; break;
                }
            }
            cout << endl;
        }

        cout << endl;
    }

    bool isInner(int x, int y)
    {
        return (0<=x) && (x<N) && (0<=y) && (y<N);
    }

    //检测在棋盘的（x，y）位置是否可以放置皇后
    bool check(int x, int y, int d)
    {
        bool ret = true;

        while(ret && isInner(x, y))
        {
            ret = ret && (m_chessboard[x][y] == 0);
            x += m_direction[d].x;
            y += m_direction[d].y;
        }

        return ret;
    }

    //在第row行尝试放置皇后
    void place(int row)
    {
        int y = row;
        if(row < N){
            for(int x=0; x<N; x++){
                if(check(x, y, 0) && check(x, y, 1) && check(x, y, 2)){
                    m_chessboard[x][y] = 1; //放置皇后
                    m_solution.insert(Pos(x,y)); //将该位置加入链表

                    place(row + 1); //在下一行放置皇后。

                    //当place(row+1)函数返回时，表示第row+1行无法找到放置皇后的地方（或找到解决方案后正常返回）
                    m_chessboard[x][y] = 0; //回溯,将当前位置的皇后移除。
                    m_solution.remove(m_solution.length() - 1);
                }
            }
        }else{
            //由于采用深度优先搜索，所以放置到第SIZE行时，表示己经找到解决方案
            m_count++;  //每找到一行方案，m_count加1。
            print();    //打印解决方案
        }
    }

public:
    QueenSolution(){init();}

    void solve()
    {
        place(0); //从第0行开始放置
        cout << "total : " << m_count << endl;
    }

};

int main()
{
    //测试：逆序打印单链表中的偶数结点
    Node* list = create_list(1, 10);
    print_list(list);


    r_print_even(list);

    destroy_list(list);

    cout << endl;

    //测试：八皇后解决方案
    QueenSolution<8> qs;
    qs.solve();

    return 0;
}
/*测试结果：
1->2->3->4->5->6->7->8->9->10->NULL
10 8 6 4 2
(0,0) (4,1) (7,2) (5,3) (2,4) (6,5) (1,6) (3,7) //显示时，使用的坐标系（原点在左上角，x向右为正，y向下为正）
 # . . . . . . .
 . . . . # . . .
 . . . . . . . #
 . . . . . # . .
 . . # . . . . .
 . . . . . . # .
 . # . . . . . .
 . . . # . . . .

(0,0) (5,1) (7,2) (2,3) (6,4) (3,5) (1,6) (4,7)
 # . . . . . . .
 . . . . . # . .
 . . . . . . . #
 . . # . . . . .
 . . . . . . # .
 . . . # . . . .
 . # . . . . . .
 . . . . # . . .

 //省略其余解决方案...

total : 92
*/

3. 经典面试题

（1）三羊献瑞

 

（2）解题思路

　　①给“祥瑞生辉三羊献气”编号0、1、2、3、4、5、6、7。

　　②利用深度优先递归+回溯方法穷举

【编程实验】三羊献瑞

//main.cpp

#include <iostream>
using namespace std;

//问题：求“三羊献瑞”所代表的4位数字
//     祥  瑞  生  辉
// +   三  羊  献  瑞
//----------------------
// 三  羊  生  瑞  气

//注意：相同汉字代表相同数字，不同汉字代表的不同数字

//给“祥瑞生辉三羊献气”等汉字编号，放入a数组中。
//即“祥”代表a[0]，“瑞”为a[1]，“生”为a[2]...等。
int a[8] = {0};
int visited[10] = {0}; //0~9只能用1次，己访问1，未访问0

//深度优先递归 + 回溯算法。
//参数curr：表示当前正在尝试放置a[curr]元素。
void threeSheep(int curr)
{
    //递归出口
    if(curr == 8){    //当curr为8时，表示a数组中的数字全部填满
        int x, y, z;
        x = a[0]*1000 + a[1]*100 + a[2]*10 + a[3];
        y = a[4]*1000 + a[5]*100 + a[6]*10 + a[1];
        z = a[4]*10000 + a[5]*1000 + a[2]*100 + a[1]*10 + a[7];
        
        //判断找到的8个数字是否满足上述“算式”，若满足则递归结束
        if((x + y) == z){
            cout << a[4] << a[5] << a[6] << a[1] << endl;        
        }
        
        return;
    }
    
    //将0~9数字一个一个放入a[curr]中试试
    for(int i=0; i<=9; i++){
        if(curr==0 && i==0) //a[0]不能等于0
            continue;
        
        if(curr==4 && i!=1) //a[4]代表“三”字，必须为1
            continue;
        
        if(!visited[i]){ //i数字是否己被放入a数组中。
            visited[i] = 1; //标记i数字己被使用
            a[curr] = i;    //尝试将i放入a[curr]
            
            threeSheep(curr + 1); //放置a[curr+1]这个数字
            
            visited[i] = 0; //回溯
        }
    }
}

int main()
{    
    threeSheep(0); //从a[0]开始放置数字
    return 0;
}
/*输出结果：
e:\\Study\\test>g++ main.cpp
e:\\Study\\test>a.exe
1085
*/

4. 小结

（1）程序运行后的栈存储区专供函数调用使用

（2）栈存储区用于保存实参、局部变量和临时变量等。

（3）利用栈存储区能够方便的实现回溯算法

（4）八皇后问题是栈回溯的经典应用。