最短编辑距离算法实现

Posted 2020-10-05 hapjin

一，算法介绍

在CS124课程的第一周提到 求解两个字符串相似度的算法---Minimum Edit Distance（最短编辑距离）算法。该算法在NLP（自然语言处理）中也会用到。

如何定义相似度呢？任给两个字符串X 和Y，使用以下三种操作将 字符串X 变到 字符串Y  ：①插入(Insert)操作；②删除操作（delete）；③替换操作(substitute)

比如 字符串X="intention" ，  字符串Y="execution"。从字符串X 转换成 字符串Y 如下图所示：

 

定义：插入操作的代价为1，删除操作的代价为1，替换操作的代价为2（称为： Levenshtein distance）。那么，"intention"  变成  "execution" 执行了三次替换，一次删除，一次插入。因此，总代价为8

而这个代价又称为编辑距离， 用之来 衡量 两个字符串的相似程度。显然，若两个字符串越相似，则从一个字符串变到另一个字符串所需要的 “操作” 步骤 就越少。

 

二，动态规则求解最短编辑距离

为什么能用动态规划来求解呢？ⓐ该问题可以分解成若干个子问题；ⓑ子问题之间具有重叠性（可“查表”），具体可参考一些动态规划的示例1，示例2.

假设字符串X的长度为n，字符串Y的长度为m，用d[n][m] 表示 字符串X 转换成 字符串Y 的最短编辑距离

定义 d[i][j] 表示 字符串X的子串X[1...i]   转换成 字符串Y 的子串 Y[1...j] 的最短编辑距离（这里的 下标从1开始，不从0开始），有如下动态规划公式：

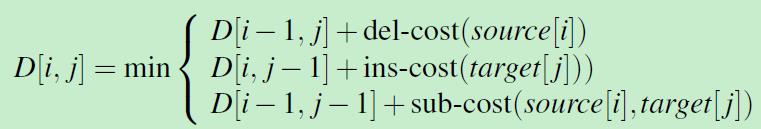
要想从 长度为 i 的源字符串X 转换成 长度为 j 的目标字符串Y，有三种方式：

①先将 源字符串X 的前 i-1 个字符 X[1...i-1] 转换成 目标字符串Y[1...j]， 然后再 删除字符串X 的第 i 个字符source[i]

②先将 源字符串X[1...j] 转换成 目标字符串Y[1...j-1] ，然后再 插入字符串Y的第 j 个字符 target[j] 

③先将 源字符串X[1...i-1] 转换成 目标字符串Y[1...j-1]，然后 源字符串中的 第 i 个字符X[i] 替换为 目标字符串的第 j 个字符 Y[j]

 

为什么 只有上述三种方式呢？

因为我们是将 源问题 的求解，分解成若干个子问题的求解，子问题的规模比原问题要小1。源问题 X[1...i]  转换成 Y[1...j]  。比如，子问题是：先将X[1...i-1] 转换成 Y[1...j] ，...

结合前面定义的 操作代价（删除和插入操作代价为1，替换操作为2），就是下面这个公式：

解释一下为什么 if source[i]=target[j]时，替换的 代价为0呢？if source[i]=target[j] 表明 字符串X 的第 i 个字符串 和 字符串Y的第 j 个字符是相同的

要想将 X[1...i] 转换成 Y[1...j] ，对于第三种转换方式：先将 源字符串X[1...i-1] 转换成 目标字符串Y[1...j-1] ，既然：字符串X 的第 i 个字符串 和 字符串Y的第 j 个字符是相同的，那就相当于“自己替换自己”，或者说是 不需要替换操作了嘛。这也是下面代码实现逻辑：

 

                if (source.charAt(i-1) == target.charAt(j-1)) {
                    dp[i][j] = dp[i - 1][j - 1];

 

 

三，代码实现

伪代码描述如下：

 

JAVA实现：

 1 public class MinimumEditDistance {
 2 
 3     public static void main(String[] args) {
 4         MinimumEditDistance med = new MinimumEditDistance();
 5         String source = "execution";
 6         String target = "intention";
 7         int result = med.similarDegree(source, target);
 8         System.out.println(result);
 9     }
10 
11     public int similarDegree(String source, String target) {
12         if(source == null || target == null)
13             throw new IllegalArgumentException("illegal input String");
14 
15         int sourceLen = source.length();
16         int targetLen = target.length();
17 
18         int[][] dp = new int[sourceLen + 1][targetLen +1];
19         //init
20         dp[0][0] = 0;
21         for(int i = 1; i <= sourceLen; i++)
22             dp[i][0] = i;
23         for(int i = 1; i <= targetLen; i++)
24             dp[0][i] = i;
25 
26         for(int i = 1; i <= sourceLen; i++) {
27             for(int j = 1; j <= targetLen; j++) {
28                 if (source.charAt(i-1) == target.charAt(j-1)) {
29                     dp[i][j] = dp[i - 1][j - 1];
30                 }else{
31                     int insert = dp[i][j - 1] + 1;//source[0,i] to target[0,j-1] then insert target[j]
32                     int delete = dp[i - 1][j] + 1;//source[0,i-1] to target[0,j] then delete source[i]
33                     int substitute = dp[i - 1][j - 1] + 2;//source[0,i-1] to target[0,j-1] then substitute(source[i] by target[j])
34 
35                     int min = min(insert, delete, substitute);
36                     dp[i][j] = min;
37                 }
38             }
39         }
40         return dp[sourceLen][targetLen];
41     }
42 
43     private int min(int insert, int delete, int substitute) {
44         int tmp = insert < delete ? insert:delete;
45         int min = tmp < substitute ? tmp:substitute;
46         return min;
47     }
48 }

 

参考：Stanford CS124课程

原文：http://www.cnblogs.com/hapjin/p/7467035.html