bzoj 1101 [POI2007]Zap - 莫比乌斯反演

Posted 2020-09-29 阿波罗2003

Description

　　FGD正在破解一段密码，他需要回答很多类似的问题：对于给定的整数a,b和d，有多少正整数对x,y，满足x<=a ，y<=b，并且gcd(x,y)=d。作为FGD的同学，FGD希望得到你的帮助。

Input

　　第一行包含一个正整数n，表示一共有n组询问。（1<=n<= 50000）接下来n行，每行表示一个询问，每行三个 正整数，分别为a,b,d。（1<=d<=a,b<=50000）

Output

　　对于每组询问，输出到输出文件zap.out一个正整数，表示满足条件的整数对数。

Sample Input

2
4 5 2
6 4 3

Sample Output

3
2
//对于第一组询问，满足条件的整数对有(2,2)，（2,4），（4，2）。对于第二组询问，满足条件的整数对有（
6,3），（3,3）。

---

　　题目大意 (这道题够短了吧，不需要大意了)

　　这道题的目标是求。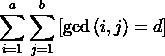

　　根据gcd的性质，等价于求。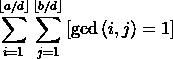

　　然后看看上一道题的“套路”，发现它的解法2可以支持多组数据。然后思考，发现莫比乌斯函数和欧拉函数有类似的性质:。赶紧抓过来化简式子。

　　

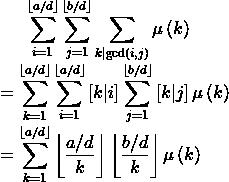
　　然后又得到了这个优美的式子。然后发现莫比乌斯函数的系数的取值并不多，所以可以分段求值，然后就可以把这道题水掉了。

　　(说实话，我并不觉得这算莫比乌斯反演，只是用到了莫比乌斯函数的性质罢了。当个入门题练练手吧)

Code

  1 /**
  2  * bzoj
  3  * Problem#1101
  4  * Accepted
  5  * Time:8956ms
  6  * Memory:1576k
  7  */
  8 #include <iostream>
  9 #include <cstdio>
 10 #include <ctime>
 11 #include <cmath>
 12 #include <cctype>
 13 #include <cstring>
 14 #include <cstdlib>
 15 #include <fstream>
 16 #include <sstream>
 17 #include <algorithm>
 18 #include <map>
 19 #include <set>
 20 #include <stack>
 21 #include <queue>
 22 #include <vector>
 23 #include <list>
 24 #ifndef WIN32
 25 #define Auto "%lld"
 26 #else
 27 #define Auto "%I64d"
 28 #endif
 29 using namespace std;
 30 typedef bool boolean;
 31 const signed int inf = (signed)((1u << 31) - 1);
 32 const signed long long llf = (signed long long)((1ull << 61) - 1);
 33 const double eps = 1e-6;
 34 const int binary_limit = 128;
 35 #define smin(a, b) a = min(a, b)
 36 #define smax(a, b) a = max(a, b)
 37 #define max3(a, b, c) max(a, max(b, c))
 38 #define min3(a, b, c) min(a, min(b, c))
 39 template<typename T>
 40 inline boolean readInteger(T& u){
 41     char x;
 42     int aFlag = 1;
 43     while(!isdigit((x = getchar())) && x != \'-\' && x != -1);
 44     if(x == -1) {
 45         ungetc(x, stdin);    
 46         return false;
 47     }
 48     if(x == \'-\'){
 49         x = getchar();
 50         aFlag = -1;
 51     }
 52     for(u = x - \'0\'; isdigit((x = getchar())); u = (u << 1) + (u << 3) + x - \'0\');
 53     ungetc(x, stdin);
 54     u *= aFlag;
 55     return true;
 56 }
 57 
 58 const int limit = 5e4;
 59 
 60 int n;
 61 int a, b, d;
 62 int num = 0;
 63 int prime[10000];
 64 int miu[limit + 1];
 65 boolean vis[limit + 1];
 66 
 67 inline void Euler() {
 68     memset(vis, false, sizeof(vis));
 69     miu[0] = 0, miu[1] = 1;
 70     for(int i = 2; i <= limit; i++) {
 71         if(!vis[i])    miu[i] = -1, prime[num++] = i;
 72         for(int j = 0; j < num && prime[j] * 1LL * i <= limit; j++) {
 73             int c = prime[j] * i;
 74             vis[c] = true;
 75             if((i % prime[j]) == 0) {
 76                 miu[c] = 0;
 77                 break;
 78             } else {
 79                 miu[c] = -1 * miu[i];
 80             }
 81         }
 82         miu[i] += miu[i - 1];
 83     }
 84 }
 85 
 86 inline void init() {
 87     readInteger(n);
 88 }
 89 
 90 long long res;
 91 
 92 inline void solve() {
 93     while(n--) {
 94         readInteger(a);
 95         readInteger(b);
 96         readInteger(d);
 97         a /= d, b /= d;
 98         if(a > b)    swap(a, b);
 99         res = 0;
100         for(int i = 1, j; i <= a; i = j + 1) {
101             j = min(a / (a / i), b / (b / i));
102             res += (a / j) * 1LL * (b / j) * (miu[j] - miu[i - 1]);
103         }
104         printf(Auto"\\n", res);
105     }
106 }
107 
108 int main() {
109     Euler();
110     init();
111     solve();
112     return 0;
113 }