Codeforces 724 G Xor-matic Number of the Graph 线性基+DFS

Posted 2020-09-08 Oncle_Ha

G. Xor-matic Number of the Graph

http://codeforces.com/problemset/problem/724/G

题意：给你一张无向图。定义一个无序三元组(u,v,s)表示u到v的(不一定为简单路径)路径上xor值为s。求出这张无向图所有不重复三元组的s之和。1≤n≤10^5,1≤m≤2*10^5。

想法：

如果做过【Wc2011 xor】这道题目(题解)，那么问题变得简单起来了。

①假设我们钦定一个(u,v),设任意一条u->v的路径xor值为X,该连通图所有小环xor值构成的序列为{Ai}。

那么(u,v)的所有路径的xor值可以由X xor {Ai}的子集xor值得到。于是一个(u,v)的 s 之和变成了求X xor{Ai}的子集可以得到多少个不同的数，这些不同的数的和是多少？

如果能知道{Ai}的子集xor值的值域，那么好办了。于是用线性基得到值域{T}。求和的话，按位考虑定义S(i)为{T}中第i为1的个数，为0的个数取个补集就好了。

对于一个(u,v)：

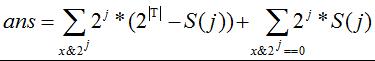
②考虑所有的无序点对(u,v)的答案。上面说过任意一条u->v的路径都可以，不如就钦定是DFS遍历得到DFS树的树上路径。

树上两点路径xor值的求法很简单：设dis(i)表示第i个到根节点路径xor值。

dis(a,b)=dis(a) xor dis(lca(a,b)) xor dis(b) xor dis(lca(a,b))=dia(a) xor dis(b)。

根据上面求ans 的式子，ans只与X的第j位是什么有关，所以设cnt(i)表示两点路径xor值第i位为1的个数。cnt(i)可以利用上面dis(a,b)=dia(a) xor dis(b)求。

对于所有(u,v):

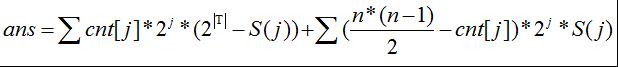
于是解决了。

#include<cstdio>
#include<vector>
#define ll long long
const int len(100000),MP(1000000007);
struct Node{int nd;ll co;};
std::vector<Node>Edge[len+10];
int n,m,u,v,top,ans,much,vis[len+10];
ll sum,S[61],cnt[61],now[61];//cnt(i) 统计 i-th =0的个数 now(i):dx^dy i-th==1的个数 
ll st[len+10],dis[len+10],All,t;
struct Base_Linear
{
    ll p[61];int size;
    void ins(ll x)
    {
        for(int j=60;j>=0;j--)
        if((x>>j)&1)
        {
            if(p[j])x^=p[j];
            else {p[j]=x;size++;break;}
        }
    }
}BL;
template <class T>void read(T &x)
{
    x=0;int f=0;char ch=getchar();
    while(ch<\'0\'||ch>\'9\'){f=(ch==\'-\');ch=getchar();}
    while(ch>=\'0\'&&ch<=\'9\'){x=x*10+ch-\'0\';ch=getchar();}
    x=f?-x:x;
}
ll power(int a,int b)
{
    ll t=1,y=a;
    for(;b;b>>=1)
    {
        if(b&1)t=(t*y)%MP;
        y=(y*y)%MP;
    }
    return t;
}
void add(int a,int b,ll c){Edge[a].push_back((Node){b,c});}
void plus(ll x)
{
    for(int j=0;j<=60;j++)
    if(((x>>j)&1)==0)cnt[j]++;
}
void Dfs(int x)
{
    much++; vis[x]=1; plus(dis[x]); st[++top]=dis[x];
    for(int v=0,sz=Edge[x].size();v<sz;v++)
    {
        Node y=Edge[x][v];
        if(vis[y.nd])BL.ins(dis[x]^dis[y.nd]^y.co);
        else 
        {
            dis[y.nd]=dis[x]^y.co;
            Dfs(y.nd);
        }
    }
}
void Back()
{
    for(int j=0;j<=60;j++)cnt[j]=now[j]=S[j]=0;
    for(int j=0;j<=60;j++)BL.p[j]=0; BL.size=0;
    much=0; All=0;
}
void Total()
{
    for(;top;top--)
    {
        for(int j=0;st[top];j++,st[top]>>=1)
        if(st[top]&1)now[j]=(now[j]+cnt[j])%MP;
    }
    for(int j=0;j<=60;j++)All|=BL.p[j];
    for(int j=0;All;j++,All>>=1)
    if(All&1)S[j]=power(2,BL.size-1);
    All=power(2,BL.size); ll C=1;
    for(int j=0;j<=60;j++,C<<=1,C%=MP)
    {
        sum=(ll)much*(much-1)/2;
        ll t1=now[j]*(All-S[j])%MP;
        ll t2=((sum-now[j])*S[j])%MP;
        ans=(ans+C*t1+C*t2)%MP;
    }
}
int main()
{
    read(n),read(m);
    for(int i=1;i<=m;i++)
    {
        read(u),read(v),read(t);
        add(u,v,t),add(v,u,t);
    }
    for(int i=1;i<=n;i++)
    if(!vis[i])//图可能不连通 
    {
        Back();
        Dfs(i);
        Total();
    }
    ans+=ans<0?MP:0;
    printf("%d",ans);
    return 0;
}

View Code