二分图判断（交叉染色）

Posted 2020-06-18 我不吃饼干呀

二分图又称二部图。

二分图是无向图。

设G=(V,E)是一个无向图。如顶点集V可分割为两个互不相交的子集，并且图中每条边依附的两个顶点都分属两个不同的子集。

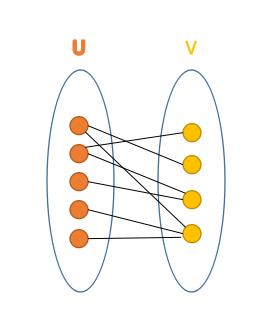
例如这就是一个二分图。 大概就是把顶点分成两堆，每堆内部没有边。

无向图G为二分图的充分必要条件是，G至少有两个顶点, 且其所有回路的长度均为偶数。

最大独立点集：在二分图中，求最少的点集，使得任意两个点之间没有直接边连接。

最小点覆盖：在二分图中，求最少的点集，使得每一条边至少都有端点在这个点集中。

 

二分图判断：二分图染色。

给一个无向图。要给图上每个顶点染色，并且使任意相邻的顶点染色不同。并且最多用两种颜色。

如果可以进行二分图染色，证明是一个二分图。

如果可以染色，就是如上图，把每一个颜色放入一个堆，就构成了二分图。

 

模板：

#include <iostream>
#include <cstdio>
#include <cstring>
#include <vector>
using namespace std;

const int MAX_V = 205;

vector<int> G[MAX_V];	// 图
int V;					// 顶点数
int color[MAX_V];		// 顶点i的颜色 1 或 -1

// 把顶点染成1或-1
bool dfs(int v, int c)
{
	color[v] = c; // 把顶点v染成颜色c
	for (int i = 0; i < G[v].size(); ++i) {
		if (color[G[v][i]] == c) return false;
		if (color[G[v][i]] == 0 && !dfs(G[v][i], -c)) return false;
	}
	return true;
}

void solve()
{
	for (int i = 0; i < V; ++i) {
		if (color[i] == 0) {
			if (!dfs(i, 1)) {
				puts("No");
				return ;
			}
		}
	}
	puts("Yes");
}

int main()
{
    int E;
    while (scanf("%d%d", &V, &E) == 2) {
		int a, b;
		for (int i = 0; i < V; ++i) G[i].clear();			
		memset(color, 0, sizeof color);
		for (int i = 0; i < E; ++i) {
			scanf("%d%d", &a, &b);
			G[a].push_back(b);
			G[b].push_back(a);
		}
		solve();
    }
    return 0;
}

 

例：hdu4751

题意：一堆人，每个人单方面认识一些人，希望把这些人分成两堆，使得每一堆的人都相互认识。

题解：把所有不互相认识的人建边，如果是二分图，则可以。

#include <iostream>
#include <cstdio>
#include <cstring>
#include <vector>
using namespace std;

const int MAX_V = 105;
bool knows[MAX_V][MAX_V];

vector<int> G[MAX_V];	
int V;	
int color[MAX_V];


bool dfs(int v, int c)
{
	color[v] = c;
	for (unsigned i = 0; i < G[v].size(); ++i) {
		if (color[G[v][i]] == c) return false;
		if (color[G[v][i]] == 0 && !dfs(G[v][i], -c)) return false;
	}
	return true;
}

void solve()
{
	for (int i = 1; i <= V; ++i) {
		if (color[i] == 0) {
			if (!dfs(i, 1)) {
				puts("NO");
				return ;
			}
		}
	}
	puts("YES");
}

int main()
{
    while (~scanf("%d", &V)) {

        memset(knows, false, sizeof knows);
        for (int i = 0; i <= V; ++i) G[i].clear();
		memset(color, 0, sizeof color);

        int a;
        for (int i = 1; i <= V; ++i) {
            while (~scanf("%d", &a) && a)
                knows[i][a] = true;
        }

        for (int i = 1; i <= V; ++i) {
            for (int j = i + 1; j <= V; ++j) {
                if (!knows[i][j] || !knows[j][i]) {
                    G[i].push_back(j);
                    G[j].push_back(i);
                }
            }
        }

        solve();
    }
    return 0;
}