codeforces 622F. The Sum of the k-th Powers 拉格朗日插值法

Posted 2020-06-16

题目链接

求sigma(i : 1 to n)i^k。 

 

为了做这个题这两天真是补了不少数论， 之前连乘法逆元都不知道...

关于拉格朗日插值法， 我是看的这里http://www.guokr.com/post/456777/， 还挺有趣...

根据题目给出的例子我们可以发现， k次方的通项公式的最高次是k+1次， 根据拉格朗日插值法， 构建一个k+1次的方程需要k+2项。

然后公式是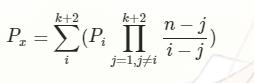  ， 对于这个题， p[i]就是i^k+（i-1)^k+(i-2)^k+.....+1^k, 这部分可以预处理出来。 自己不会搞公式 ， 从http://www.cnblogs.com/qscqesze/p/5207132.html这里盗的（雾

我们发现上面就是(n-1)*(n-2)......*(n-k-2)/(n-i), 上面的那部分预处理出来， 除(n-i)相当于乘(n-i)的乘法逆元。

下面那部分就是(i-1)*(i-2)*...(i-i+1)     *   (i-i-1)*(i-i-2)*......(i-k-2), 相当于两个阶乘相乘， 阶乘预处理出来， 然后注意一下后面的正负号就可以了。

#include <iostream>
#include <vector>
#include <cstdio>
#include <cstring>
#include <algorithm>
#include <cmath>
#include <map>
#include <set>
#include <string>
#include <queue>
#include <stack>
#include <bitset>
using namespace std;
#define pb(x) push_back(x)
#define ll long long
#define mk(x, y) make_pair(x, y)
#define lson l, m, rt<<1
#define mem(a) memset(a, 0, sizeof(a))
#define rson m+1, r, rt<<1|1
#define mem1(a) memset(a, -1, sizeof(a))
#define mem2(a) memset(a, 0x3f, sizeof(a))
#define rep(i, n, a) for(int i = a; i<n; i++)
#define fi first
#define se second
typedef pair<int, int> pll;
const double PI = acos(-1.0);
const double eps = 1e-8;
const ll mod = 1e9+7;
const int inf = 1061109567;
const int dir[][2] = { {-1, 0}, {1, 0}, {0, -1}, {0, 1} };
const int maxn = 1e6+5;
ll f[maxn], fac[maxn];
ll pow(ll a, ll b) {
    ll ret = 1;
    while(b) {
        if(b&1)
            ret = (ret*a)%mod;
        a = (a*a)%mod;
        b>>=1;
    }
    return ret;
}
int main()
{
    ll n, k;
    cin>>n>>k;
    for(int i = 1; i<=k+2; i++) {
        f[i] = (f[i-1]+pow(i*1LL, k))%mod;
    }
    fac[0] = 1;
    for(int i = 1; i<maxn; i++) {
        fac[i] = (fac[i-1]*i)%mod;
    }
    if(n<=k+2) {
        cout<<f[n]<<endl;
        return 0;
    }
    ll cur = 1, ans = 0;
    for(int i = 1; i<=k+2; i++) {
        cur = (cur*(n-i))%mod;
    }
    for(int i = 1; i<=k+2; i++) {
        ll tmp = pow(n-i, mod-2)%mod;
        ll tmp1 = pow(fac[i-1]%mod*fac[k+2-i]%mod, mod-2)%mod;
        int sign = (k+2-i)%2?-1:1;
        ans = (ans + sign*tmp*tmp1%mod*f[i]%mod*cur%mod)%mod;
    }
    ans = (ans+mod)%mod;
    cout<<ans<<endl;
    return 0;
}