hdu-4810 Wall Painting(组合数学)
Posted LittlePointer
tags:
篇首语:本文由小常识网(cha138.com)小编为大家整理,主要介绍了hdu-4810 Wall Painting(组合数学)相关的知识,希望对你有一定的参考价值。
题目链接:
Wall Painting
Time Limit: 10000/5000 MS (Java/Others) Memory Limit: 32768/32768 K (Java/Others)
Total Submission(s): 2681 Accepted Submission(s): 857
Problem Description
Ms.Fang loves painting very much. She paints GFW(Great Funny Wall) every day. Every day before painting, she produces a wonderful color of pigments by mixing water and some bags of pigments. On the K-th day, she will select K specific bags of pigments and mix them to get a color of pigments which she will use that day. When she mixes a bag of pigments with color A and a bag of pigments with color B, she will get pigments with color A xor B.
When she mixes two bags of pigments with the same color, she will get color zero for some strange reasons. Now, her husband Mr.Fang has no idea about which K bags of pigments Ms.Fang will select on the K-th day. He wonders the sum of the colors Ms.Fang will get with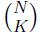 different plans.
different plans.
For example, assume n = 3, K = 2 and three bags of pigments with color 2, 1, 2. She can get color 3, 3, 0 with 3 different plans. In this instance, the answer Mr.Fang wants to get on the second day is 3 + 3 + 0 = 6.
Mr.Fang is so busy that he doesn’t want to spend too much time on it. Can you help him?
You should tell Mr.Fang the answer from the first day to the n-th day.
When she mixes two bags of pigments with the same color, she will get color zero for some strange reasons. Now, her husband Mr.Fang has no idea about which K bags of pigments Ms.Fang will select on the K-th day. He wonders the sum of the colors Ms.Fang will get with
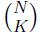 different plans.
different plans.For example, assume n = 3, K = 2 and three bags of pigments with color 2, 1, 2. She can get color 3, 3, 0 with 3 different plans. In this instance, the answer Mr.Fang wants to get on the second day is 3 + 3 + 0 = 6.
Mr.Fang is so busy that he doesn’t want to spend too much time on it. Can you help him?
You should tell Mr.Fang the answer from the first day to the n-th day.
Input
There are several test cases, please process till EOF.
For each test case, the first line contains a single integer N(1 <= N <= 103).The second line contains N integers. The i-th integer represents the color of the pigments in the i-th bag.
For each test case, the first line contains a single integer N(1 <= N <= 103).The second line contains N integers. The i-th integer represents the color of the pigments in the i-th bag.
Output
For each test case, output N integers in a line representing the answers(mod 106 +3) from the first day to the n-th day.
Sample Input
4
1 2 10 1
Sample Output
14 36 30 8
题意:
取k个数的异或和是多少,k从1到n;
思路:
按位计算对ans[k]的贡献,计数第i为上有多少个1和多少个0,然后算取法就好了;
AC代码:
#include <bits/stdc++.h>
using namespace std;
typedef long long LL;
const int maxn=1e3+10;
const LL mod=1e6+3;
LL ans[maxn],c[maxn][maxn];
int n,a[maxn];
inline void Init()
{
memset(c,0,sizeof(c));
c[0][0]=1;
for(int i=1;i<=maxn-2;i++)
{
c[i][0]=c[i][i]=1;
for(int j=1;j<i;j++)
c[i][j]=(c[i-1][j-1]+c[i-1][j])%mod;
}
}
int main()
{
Init();
while(scanf("%d",&n)!=EOF)
{
for(int i=1;i<=n;i++)scanf("%d",&a[i]),ans[i]=0;
LL p=1;
for(int i=0;i<=31;i++)
{
int x=0,y=0;
for(int j=1;j<=n;j++)
{
if((a[j]>>i)&1)x++;
else y++;
}
for(int j=1;j<=n;j++)
{
for(int k=1;k<=j;k+=2)
{
ans[j]=(ans[j]+c[x][k]*c[y][j-k]%mod*p)%mod;
}
}
p=2*p%mod;
}
for(int i=1;i<n;i++)printf("%lld ",ans[i]);
printf("%lld\n",ans[n]);
}
return 0;
}
以上是关于hdu-4810 Wall Painting(组合数学)的主要内容,如果未能解决你的问题,请参考以下文章