[LeetCode] 882. Reachable Nodes In Subdivided Graph 细分图中的可到达结点

Posted 2022-02-06 grandyang

Starting with an?undirected?graph (the "original graph") with nodes from?0?to?N-1, subdivisions are made to some of the edges.

The graph is given as follows:?edges[k]?is a list of integer pairs?(i, j, n)?such that?(i, j)?is an edge of the original graph,

and?n?is the total number of?new?nodes on that edge.?

Then, the edge?(i, j)?is deleted from the original graph,?n?new nodes?(x_1, x_2, ..., x_n)?are added to the original graph,

and?n+1?new?edges?(i, x_1), (x_1, x_2), (x_2, x_3), ..., (x_n-1, x_n), (x_n, j)?are added to the original?graph.

Now, you start at node?0?from the original graph, and in each move, you travel along one?edge.?

Return how many nodes you can reach in at most?Mmoves.

Example 1:

Input: `edges` = [[0,1,10],[0,2,1],[1,2,2]], M = 6, N = 3
Output: 13
Explanation:
The nodes that are reachable in the final graph after M = 6 moves are indicated below.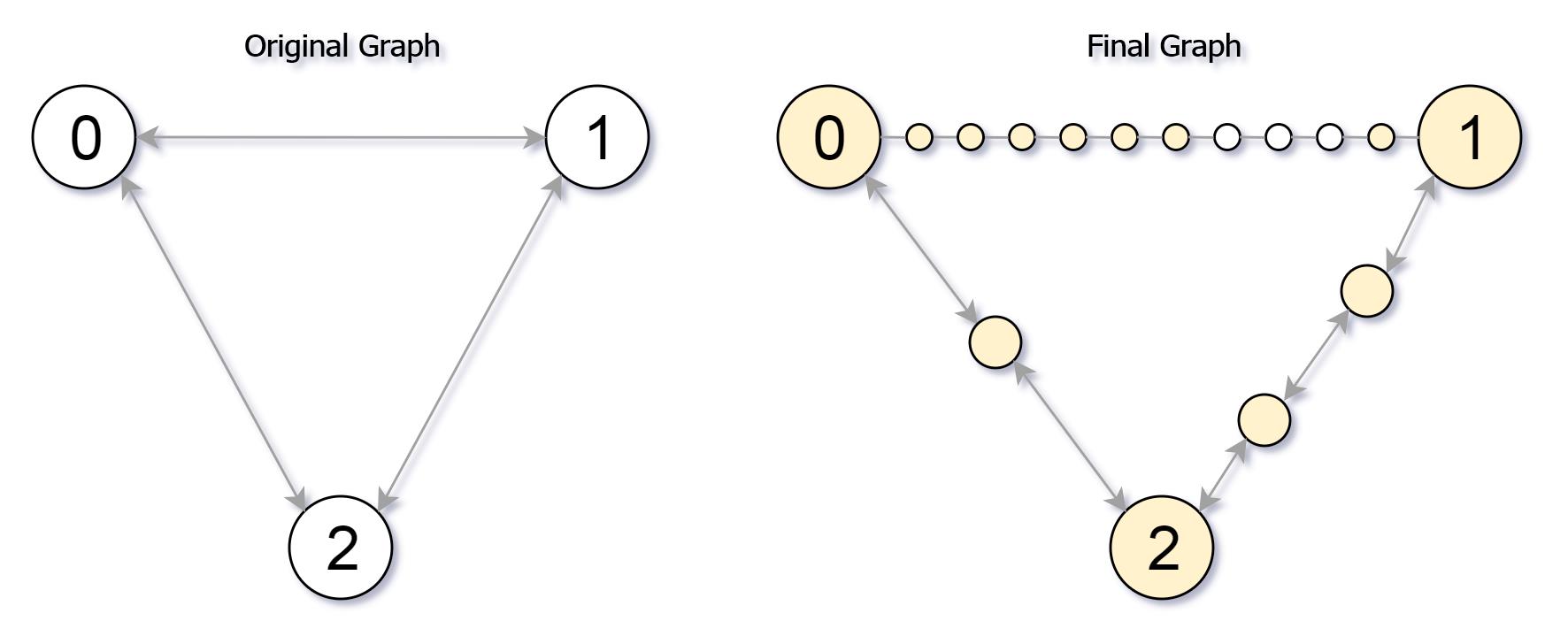

Example 2:

Input: `edges` = [[0,1,4],[1,2,6],[0,2,8],[1,3,1]], M = 10, N = 4
Output: 23

Note:

1. 0 <= edges.length <= 10000
2. 0 <= edges[i][0] <?edges[i][1] < N
3. There does not exist any?i != j?for which?edges[i][0] == edges[j][0]?and?edges[i][1] == edges[j][1].
4. The original graph?has no parallel edges.
5. 0 <= edges[i][2] <= 10000
6. 0 <= M <= 10^9
7. 1 <= N <= 3000
8. A reachable node is a node that can be travelled to?using at most?M moves starting from?node 0.

Github 同步地址:

https://github.com/grandyang/leetcode/issues/882

参考资料：

https://leetcode.com/problems/reachable-nodes-in-subdivided-graph/

LeetCode All in One 题目讲解汇总(持续更新中...)