CF 题目集锦 PART 2 #260 div 1 C

Posted 2022-12-09 阿蒋

【#260 div 1 C. Civilization】

【原题】

C. Civilization time limit per test 1 second memory limit per test 256 megabytes input standard input output standard output

Andrew plays a game called "Civilization". Dima helps him.

The game has n cities and m bidirectional roads. The cities are numbered from 1 to n. Between any pair of cities there either is a single (unique) path, or there is no path at all. A path is such a sequence of distinct cities v1, v2, ..., vk, that there is a road between any contiguous cities vi andvi + 1 (1 ≤ i < k). The length of the described path equals to (k - 1). We assume that two cities lie in the same region if and only if, there is a path connecting these two cities.

During the game events of two types take place:

1. Andrew asks Dima about the length of the longest path in the region where city x lies.
2. Andrew asks Dima to merge the region where city x lies with the region where city y lies. If the cities lie in the same region, then no merging is needed. Otherwise, you need to merge the regions as follows: choose a city from the first region, a city from the second region and connect them by a road so as to minimize the length of the longest path in the resulting region. If there are multiple ways to do so, you are allowed to choose any of them.

Dima finds it hard to execute Andrew's queries, so he asks you to help him. Help Dima.

Input

The first line contains three integers n, m, q (1 ≤ n ≤ 3·105; 0 ≤ m < n; 1 ≤ q ≤ 3·105) — the number of cities, the number of the roads we already have and the number of queries, correspondingly.

Each of the following m lines contains two integers, ai and bi (ai ≠ bi; 1 ≤ ai, bi ≤ n). These numbers represent the road between cities ai andbi. There can be at most one road between two cities.

Each of the following q lines contains one of the two events in the following format:

• 1 xi. It is the request Andrew gives to Dima to find the length of the maximum path in the region that contains city xi (1 ≤ xi ≤ n).
• 2 xi yi. It is the request Andrew gives to Dima to merge the region that contains city xi and the region that contains city yi (1 ≤ xi, yi ≤ n). Note, that xi can be equal to yi.

Output

For each event of the first type print the answer on a separate line.

Sample test(s) input

6 0 6
2 1 2
2 3 4
2 5 6
2 3 2
2 5 3
1 1

output

4

【题意】开始有N个彼此独立的点。每次有两个操作：查询x所在的树的直径；合并x和y所在的树使新的树直径最小。

【分析】拿到手以为是神题，然后愉快地被SKYDEC大爷喷了。

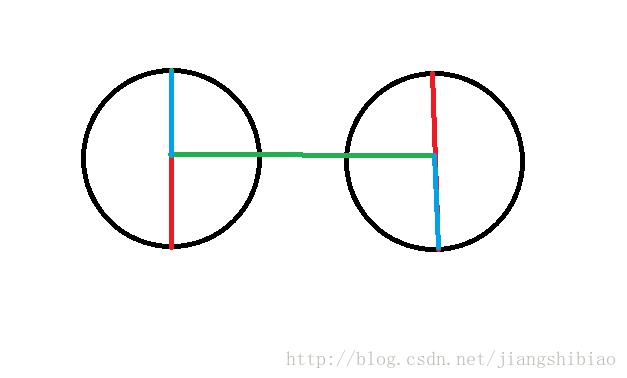
如图，假设红线是两棵树的直径（用一个数组维护）。现在我要以最佳的方式来连接使直径最小。

假设我们把两个连接点都设在原树的直径上。

显然我们要把直径的中点都连起来，这样子两条路线的构成的4种直径（红/蓝->绿->红/蓝）的max会最小。

可以证明，如果所取的连接点不在原树的直径上，结果不会更优。设某个原树的连接点是P，原直径是A和B，且P不在A和B上，那么max(dis(P,A),dis(P,B))一定大于等于直径的一半。

当然还要注意一些细节。

①如果直径是奇数，一半的话是除以2加1。

②连接两树还需要额外的1的边。

③取新树直径的时候，别忘了和原树的两个直径取max。

【代码】

#include<cstdio>
#include<algorithm>
#define N 300005
using namespace std;
struct arrint go,next;a[N*2];
int end[N],visit[N],d[N],ans[N],f[N];
int n,m,Q,i,x,y,z,father,now,num,cnt,opt;
inline void add(int u,int v)a[++cnt].go=v;a[cnt].next=end[u];end[u]=cnt;

void dfs(int k)

  visit[k]=num;f[k]=father;
  if (d[k]>d[now]) now=k;
  for (int i=end[k];i;i=a[i].next)
  
    int go=a[i].go;if (visit[go]==num) continue;
    d[go]=d[k]+1;dfs(go);
  

int get(int u)return f[u]==u?u:f[u]=get(f[u]);
int main()

  scanf("%d%d%d",&n,&m,&Q);
  for (i=1;i<=m;i++)
    scanf("%d%d",&x,&y),add(x,y),add(y,x);
  for (i=1;i<=n;i++)
    if (!visit[i]) 
    
      father=i;
      now=i;num=1;d[i]=0;dfs(i);
      num=2;d[now]=0;dfs(now);
      ans[father]=d[now];
    
  while (Q--)
  
    scanf("%d%d",&opt,&x);
    if (opt==1) printf("%d\\n",ans[get(x)]);continue;
    scanf("%d",&y);x=get(x);y=get(y);if (x==y) continue;
    f[y]=x;ans[x]=max(max(ans[y],ans[x]),(ans[x]+1)/2+(ans[y]+1)/2+1);
  
  return 0;