Codeforces Round #487 (Div. 2) A Mist of Florescence (暴力构造)

Posted 2020-11-17 qldabiaoge

C. A Mist of Florescence

time limit per test

1 second

memory limit per test

256 megabytes

input

standard input

output

standard output

As the boat drifts down the river, a wood full of blossoms shows up on the riverfront.

"I‘ve been here once," Mino exclaims with delight, "it‘s breathtakingly amazing."

"What is it like?"

"Look, Kanno, you‘ve got your paintbrush, and I‘ve got my words. Have a try, shall we?"

There are four kinds of flowers in the wood, Amaranths, Begonias, Centaureas and Dianthuses.

The wood can be represented by a rectangular grid of $\mathrm{nn rows\; and mm columns.\; In\; each\; cell\; of\; the\; grid,\; there\; is\; exactly\; one\; type\; of\; flowers.}$

According to Mino, the numbers of connected components formed by each kind of flowers are $\mathrm{aa, bb, cc and dd respectively.\; Two\; cells\; are\; considered\; in\; the\; same\; connected\; component\; if\; and\; only\; if\; a\; path\; exists\; between\; them\; that\; moves\; between\; cells\; sharing\; common\; edges\; and\; passes\; only\; through\; cells\; containing\; the\; same\; flowers.}$

You are to help Kanno depict such a grid of flowers, with $\mathrm{nn and mm arbitrarily\; chosen\; under\; the\; constraints\; given\; below.\; It\; can\; be\; shown\; that\; at\; least\; one\; solution\; exists\; under\; the\; constraints\; of\; this\; problem.}$

Note that you can choose arbitrary $\mathrm{nn and mm under\; the\; constraints\; below,\; they\; are\; not\; given\; in\; the\; input.}$

Input

The first and only line of input contains four space-separated integers $\mathrm{aa, bb, cc and dd (1\le a,b,c,d\le 1001\le a,b,c,d\le 100) —\; the\; required\; number\; of\; connected\; components\; of\; Amaranths,\; Begonias,\; Centaureas\; and\; Dianthuses,\; respectively.}$

Output

In the first line, output two space-separated integers $\mathrm{nn and mm (1\le n,m\le 501\le n,m\le 50) —\; the\; number\; of\; rows\; and\; the\; number\; of\; columns\; in\; the\; grid\; respectively.}$

Then output $\mathrm{nn lines\; each\; consisting\; of mm consecutive\; English\; letters,\; representing\; one\; row\; of\; the\; grid.\; Each\; letter\; should\; be\; among\; ‘A‘,\; ‘B‘,\; ‘C‘\; and\; ‘D‘,\; representing\; Amaranths,\; Begonias,\; Centaureas\; and\; Dianthuses,\; respectively.}$

In case there are multiple solutions, print any. You can output each letter in either case (upper or lower).

Examples

input

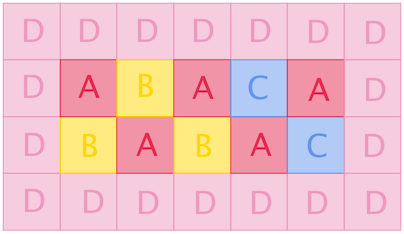
5 3 2 1

output

4 7
DDDDDDD
DABACAD
DBABACD
DDDDDDD

input

50 50 1 1

output

4 50
CCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCCC
ABABABABABABABABABABABABABABABABABABABABABABABABAB
BABABABABABABABABABABABABABABABABABABABABABABABABA
DDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDDD

input

1 6 4 5

output

7 7
DDDDDDD
DDDBDBD
DDCDCDD
DBDADBD
DDCDCDD
DBDBDDD
DDDDDDD

Note

In the first example, each cell of Amaranths, Begonias and Centaureas forms a connected component, while all the Dianthuses form one.

你们看代码吧 ！这题简直超级无脑，超级暴力，然而我还写不出 ！！！我太菜了我菜爆了

  1 #include<cstdio>
  2 #include<iostream>
  3 #include<algorithm>
  4 #include<string>
  5 #include<cstring>
  6 using namespace std;
  7 typedef long long LL;
  8 const int maxn = 3e5 + 10;
  9 
 10 char tu[55][55];
 11 int num[10];
 12 int dx[4] = {0, 0, 1, -1};
 13 int dy[4] = {1, -1, 0, 0};
 14 int check(int i, int j ) {
 15     if (i < 0 || i >= 50 || j < 0 || j >= 50) return 0;
 16     return 1;
 17 }
 18 int check1(int i, int j, char cnt ) {
 19     for (int k = 0 ; k < 4 ; k++) {
 20         int nx = i + dx[k];
 21         int ny = j + dy[k];
 22         // printf("%c %c
",tu[nx][ny],cnt);
 23         if (check(nx, ny)) {
 24             if (tu[nx][ny] == cnt) return 0;
 25         }
 26     }
 27     return 1;
 28 }
 29 int main() {
 30     char s[10] = "ABCD";
 31     scanf("%d%d%d%d", &num[0], &num[1], &num[2], &num[3]);
 32     for (int i = 0 ; i < 50 ; i++) {
 33         for (int j = 0 ; j < 50 ; j++) {
 34             if (i < 25 && j < 25)   tu[i][j] = ‘A‘;
 35             if (i < 25 && j >= 25)  tu[i][j] = ‘B‘;
 36             if (i >= 25 && j < 25)  tu[i][j] = ‘C‘;
 37             if (i >= 25 && j >= 25) tu[i][j] = ‘D‘;
 38         }
 39     }
 40     if (num[0] - 1) {
 41         int cnt = 1;
 42         for (int i = 0 ; i < 50 ; i++) {
 43             for (int j = 0 ; j < 50 ; j++) {
 44                 if (i >= 25 && j >= 25) {
 45                     int sum = 0;
 46                     for (int k = 25 ; k < 50 ; k++) {
 47                         if (tu[i - 1][k] == ‘A‘) sum++;
 48                     }
 49                     if (sum > 12) break;
 50                     if (tu[i][j] == ‘D‘ && check1(i, j, s[0] ) ) {
 51                         tu[i][j] = ‘A‘;
 52                         cnt++;
 53                     }
 54                     if (cnt == num[0]) break;
 55                 }
 56             }
 57             if (cnt == num[0]) break;
 58         }
 59     }
 60     if (num[1] - 1) {
 61         int cnt = 1;
 62         for (int i = 0 ; i < 50 ; i++) {
 63             for (int j = 0 ; j < 50 ; j++) {
 64                 if (i >= 25 && j < 25) {
 65                     int sum = 0;
 66                     for (int k = 0 ; k < 25 ; k++) {
 67                         if (tu[i - 1][k] == ‘B‘) sum++;
 68                     }
 69                     if (sum > 12) break;
 70                     if (tu[i][j] == ‘C‘ && check1(i, j, s[1]) ) {
 71                         tu[i][j] = ‘B‘;
 72                         cnt++;
 73                     }
 74                 }
 75                 if (cnt == num[1]) break;
 76             }
 77             if (cnt == num[1]) break;
 78         }
 79     }
 80     if (num[2] - 1) {
 81         int cnt = 1;
 82         for (int i = 0 ; i < 50 ; i++) {
 83             for (int j = 0 ; j < 50 ; j++) {
 84                 if (i < 25 && j >= 25) {
 85                     int sum = 0;
 86                     for (int k = 25 ; k < 50 ; k++) {
 87                         if (tu[i - 1][k] == ‘C‘) sum++;
 88                     }
 89                     if (sum > 12) break;
 90                     if (tu[i][j] == ‘B‘ && check1(i, j, s[2]) ) {
 91                         tu[i][j] = ‘C‘;
 92                         cnt++;
 93                     }
 94                 }
 95                 if (cnt == num[2]) break;
 96             }
 97             if (cnt == num[2]) break;
 98         }
 99     }
100     if (num[3] - 1) {
101         int cnt = 1;
102         for (int i = 0 ; i < 50 ; i++) {
103             for (int j = 0 ; j < 50 ; j++) {
104                 if (i < 25 && j < 25) {
105                     int sum = 0;
106                     for (int k = 0 ; k < 25 ; k++) {
107                         if (tu[i - 1][k] == ‘D‘) sum++;
108                     }
109                     if (sum > 12) break;
110                     if (tu[i][j] == ‘A‘ && check1(i, j, s[3]) ) {
111                         tu[i][j] = ‘D‘;
112                         cnt++;
113                     }
114                 }
115                 if (cnt == num[3]) break;
116             }
117             if (cnt == num[3]) break;
118         }
119     }
120     printf("50 50
");
121     for (int i = 0 ; i < 50 ; i++)
122         printf("%s
", tu[i]);
123     return 0;
124 }

View