HOROWITZ

Posted 2021-03-15 arconicsoftwares

Horowitz-Ostrogradsky方法

Hermite方法避免了完全分解，但仍要求无平方分解和关于的部分分式分解。Horowitz-Ostrogradsky方法则不需要其他工具，通过待定系数法将问题归结为线性方程的求解问题。

设其中为积分的有理部分，由部分分式的结果可知，。求导得到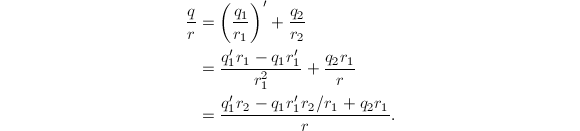从而问题归结为求，满足且，。以，的系数作为未知变量，这是一个至多维的线性方程组问题。

注1 设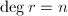，则Hermite方法的时间复杂度为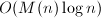[3]，其中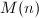为乘法的复杂度。而Horowitz-Ostrogradsky方法的复杂度为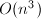（解线性方程组），尽管渐进意义上后者要比前者慢将近两个量级，但在实践中还是要分析具体情况而定。

Rothstein-Trager方法

下面的问题是如何求出对数部分，因此我们设无平方因子的首一多项式，并可设其中为互不相同的常数，为无平方因子的首一多项式，两两互素（可以通过合并项来满足这些条件）。求导得到由于无平方因子且两两互素可知记，则有及于是对于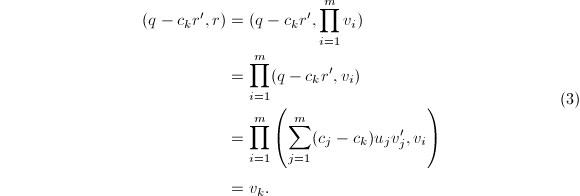最后一个等式成立是因为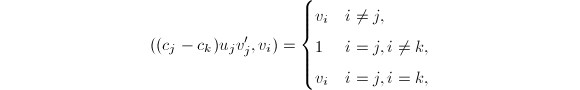从而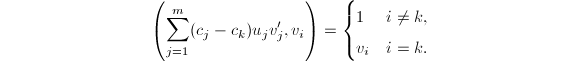

由式(3)可以看出，当我们找出系数后即可通过最大公因子的计算求出。如何求出呢？同样的论证可以知道，而（此结式称为Rothstein-Trager结式），故求解关于的方程即可得到所有的系数。

例2 设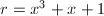，，则Rothstein-Trager结式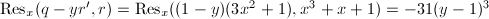。从而方程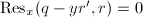有唯一的根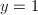，最大公因子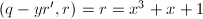，故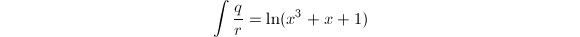即为积分结果。 ◇

注2 Rothstein-Trager方法的时间复杂度为[3]。

注3 对于高次Rothstein-Trager结式，其根可能无法显式地表达出来。设无平方因子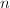，个根为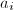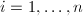（），不难求得(1)中的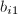，此时可直接将积分表示为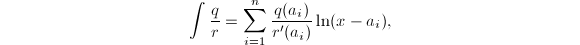便于进一步的运算。