部分题解乱写

Posted 2020-11-15 hzoi-kx

csps模拟测试59

T2 Silhouette

神题

首先有一个很显然的性质：答案与a，b的顺序无关，这个很好证。

每个点有一个最大值，即点（i,j）为min(a_i,b_j) 

手模一下样例可以发现，整张网格的最大值分成若干块，而块的数目不会超过2*n。

考虑在这些块上入手，容易发现块与块之间是互不影响的。

枚举每一块，又可以发现一个十分优秀的性质：这些块要么是矩形，要么是L形。

提取子问题：

给定一个a*b的矩形，矩形内每个数为[0,s],要求每一行每一列的最大值为s求方案数。

容斥，设f[i]表示至少i行不满足条件的方案数。

先选出i行，是一个C(a,i),

然后枚举每一列的情况，这一列中枚举的那i个位置是一定要不合法的，也就是只能在[0,s-1]中选，即s^i。

要保证这一列合法，另外a-i个位置一定要有一个s，(s+1)^(a-i)-s^(a-i)。

每一列都是这样，最后再直接^b即可

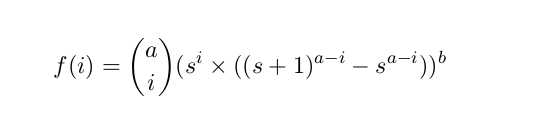
再考虑L形的情况，首先L形的只有可能是两个矩形的交汇处不合法（想一想为什么），我们在枚举的时候不合法的点只能在那个矩形里面选取

所以我们只需要把上式略微改一下就行了。

容斥一下就好了

 

 1 #include<cstdio>
 2 #include<algorithm>
 3 #include<iostream>
 4 #define int long long
 5 using namespace std;
 6 const int mod=1e9+7,N=1e5+5;
 7 int a[N],b[N],n,js[N],js_inv[N],ans=1,tt[N<<1],tot;
 8 inline int read()
 9 {
10     int x=0;char c=getchar();
11     while(c<‘0‘||c>‘9‘)   c=getchar();
12     while(c>=‘0‘&&c<=‘9‘) x=x*10+c-48,c=getchar();
13     return x;
14 }
15 inline int C(int n,int m){return js[n]*js_inv[m]%mod*js_inv[n-m]%mod;}
16 inline int pow(int a,int b)
17 {
18     int ans=1;
19     for(;b;b>>=1,a=a*a%mod) if(b&1) ans=ans*a%mod;
20     return ans;
21 }
22 inline int calc(const int a,const int b,const int c,const int d,const int s)
23 {
24     int cnt=0;
25     for(register int i=0;i<=a;i++)
26     {
27         int sum=C(a,i)*pow(pow(s,i)%mod*((pow(s+1,a+c-i)-pow(s,a+c-i))%mod+mod)%mod,b)%mod*pow(pow(s,i)*pow(s+1,a-i)%mod,d)%mod;
28         if(i&1) (cnt-=sum)%=mod;
29         else (cnt+=sum)%=mod;
30     }
31     return (cnt%mod+mod)%mod;
32 }
33 inline void work()
34 {
35     register int i=n,j=n,lst1=n+1,lst2=n+1;
36     for(int k=tot;k;k--)
37     {
38         while(i&&a[i-1]==tt[k]) i--;
39         while(j&&b[j-1]==tt[k]) j--;
40         (ans*=calc(lst1-i,lst2-j,n-lst1+1,n-lst2+1,tt[k]))%=mod;
41         lst1=i,lst2=j;
42     }
43 }
44 signed main()
45 {
46     js[0]=1;
47     for(register int i=1;i<=N-5;i++) js[i]=js[i-1]*i%mod;
48     js_inv[N-5]=pow(js[N-5],mod-2);
49     for(register int i=N-6;i>=1;i--) js_inv[i]=js_inv[i+1]*(i+1)%mod;
50     js_inv[0]=1;
51     n=read();
52     for(register int i=1;i<=n;i++) a[i]=read(),tt[++tot]=a[i];
53     for(register int i=1;i<=n;i++) b[i]=read(),tt[++tot]=b[i];
54     sort(tt+1,tt+tot+1);tot=unique(tt+1,tt+tot+1)-tt-1;
55     sort(a+1,a+n+1);sort(b+1,b+n+1);
56     if(a[n]^b[n]) return puts("0"),0;
57     work();
58     printf("%lld
",ans);
59     return 0;
60 }

View Code