题解 LG P2264

Posted 2021-03-08 ct666rp

tags:

篇首语：本文由小常识网(cha138.com)小编为大家整理，主要介绍了题解 LG P2264相关的知识，希望对你有一定的参考价值。

这是题解P2264

先讲一下Trie，其实Trie也名前缀树，就是说：如果Trie中某串是某串的前缀，那么我们可以共用这个串也就是这样：

插入h、hk、jc,jcfa

那么，h节点会给h和hk共用,jc串也会给jcfa共用。

对于本题来说，因为要考虑每个句子后要标记次数，所以Trie要有附加数组。

拿样例为例，我们原本会构建这样的一棵TRIE树：

技术图片

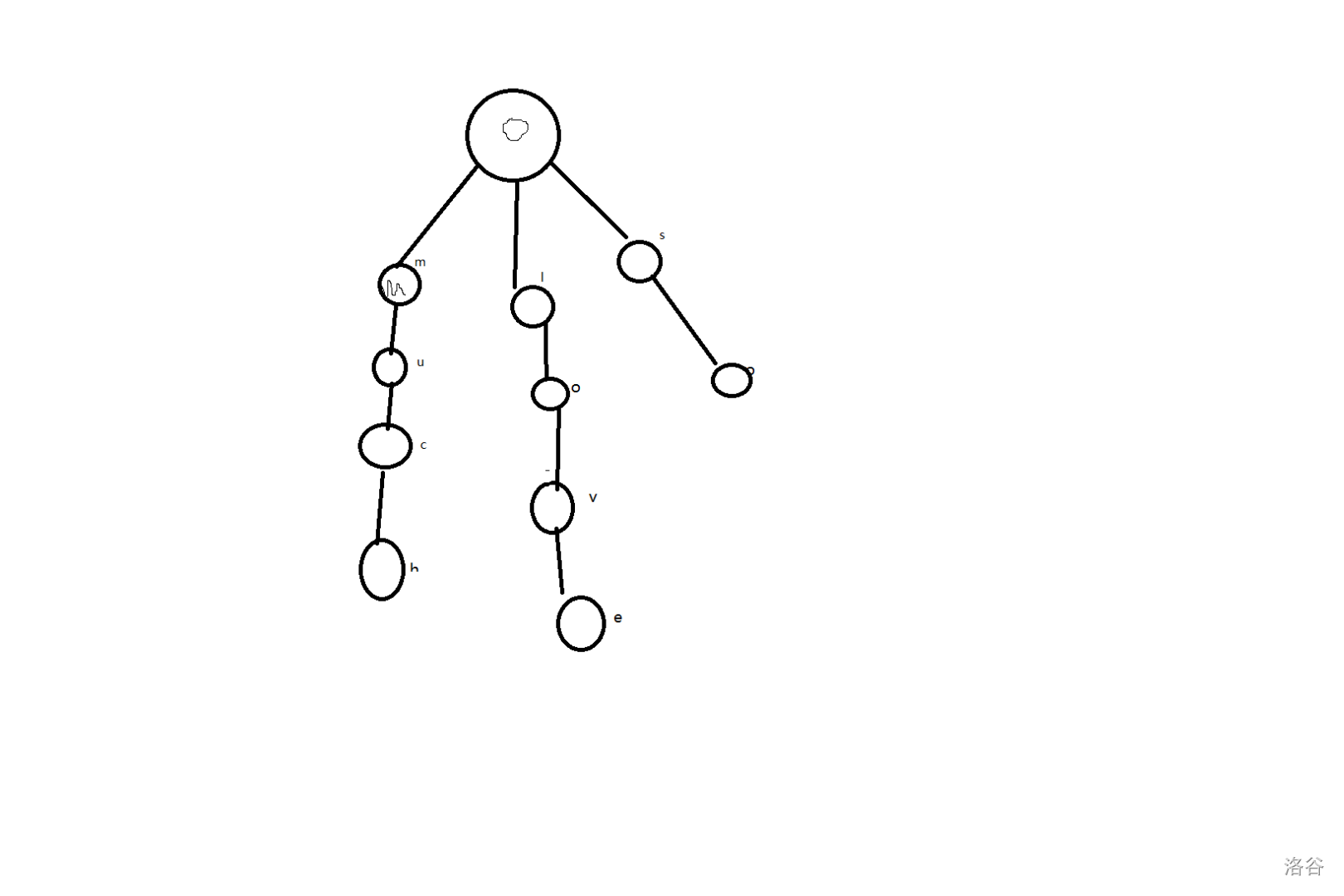
但我们只要在底下最后一个点附加一个val数组来记录次数，因为要记录次数。

那么trie就沉了这样：

int ch[N][C],tot=1;
int check[N];

怎么写也就更简单了：

#include<iostream>
#include<string>
#include<cstdio>
using namespace std;
#define N 50005
#define rr register
#define C 27
string s,w;
int n; 
int ch[N][C],tot=1,ans;
string w1[N];
int check[N]; 
int idx(char s);
void insert(string s);
int find(string s);
char turn(char x);
int main()
{ 
  cin>>n;
  for(rr int i=1;i<=n;i++)
  {
      cin>>w1[i];
  for(rr int j=0;j<=w1[i].size()-1;j++)
      w1[i][j]=turn(w1[i][j]);
      insert(w1[i]);
  }
  int len;
  getchar();
  getline(cin,s);
  len=s.size()-1;
  for(rr int i=0;i<=len;i++)
  {
          s[i]=turn(s[i]);
        if(s[i]==‘.‘ or s[i]==‘,‘ or s[i]==‘ ‘)
        {
            if(find(w)==1)
             ans++;
            if(s[i]==‘.‘)
             for(rr int j=1;j<=n;j++)
             insert(w1[j]); 
            w="";
        }
        else
            w+=s[i];
    }
    cout<<ans;
  return 0; 
}
int idx(char x)
{
    int v;
    v=(int)(x-‘a‘);
    return v;
}
void insert(string s)
{
    int len=s.size()-1;
    int las=0,w;
    for(rr int i=0;i<=len;i++)
    {
        w=idx(s[i]);
        if(ch[las][w]==0) 
         ch[las][w]=++tot;
        las=ch[las][w];
    }
    check[las]=1; 
    return;
}
int find(string s)
{
    int len=s.size()-1;
    int las=0,w;
    for(rr int i=0;i<=len;i++)
    {
        w=idx(s[i]);
        if(ch[las][w]==0) 
         return 0; 
        las=ch[las][w];
    }
    if(check[las]==1) 
    {
     check[las]++;
     return 1;    
    }
    else return 0;
} 
char turn(char x)
{
    if(x>=‘A‘&&x<=‘Z‘) return (x+32);
    else return x;
}