Huffman coding

Posted 2021-01-25 lab601

Huffman coding

1、Basic Technique

Huffmam coding是David A. Huffman在MIT上学期间发明的一种编码方式，并以他的名字命名。Huffman coding 和 Shannon-Fano coding方法正好相反，Huffman coding是从下到上，Shannon-Fano coding 是从上到下。

编码步骤

• 1 为每个符号建立一个叶子节点，将它们放到一个队列中，并为每个节点分配权重（符号出现次数）；
• 2 当队列中的符号个数大于1：
  • 2.1 将权重最低的两个节点从队列中移除；
  • 2.2 为这两个节点分配符号0和1；
  • 2.3 新建一个新节点，作为上面两个节点的父节点，权重是子节点的权重和；
  • 2.4 将新节点加入队列

• 3 剩下的一个节点是根节点，编码结束。

  2、Example

  Symbol	A	B	C	D	E
  count	15	7	6	6	5
  probabilities	0.38461538	0.17948718	0.15384615	0.15384615	0.12830513

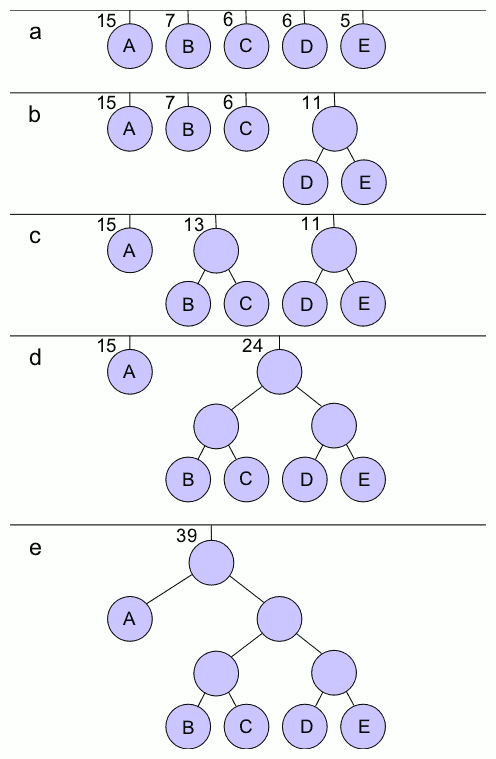
首先，D&E权重最小，将它们相加构成新的节点，权重0.28205128, 两个子节点分配0和1，如上图b所示.

Symbol	A	D&E	B	C
count	15	11	7	6
probabilities	0.38461538	0.28205128	0.17948718	0.15384615

然后，合并最小的两个符号B&C，组成新节点B&C,权重0.33333333,两个子节点分配0和1，如上图c所示

Symbol	A	B&C	D&E
count	15	13	11
probabilities	0.38461538	0.33333333	0.28205128

再将B&C和D&E合并,组成新节点B&C&D&E，如上图d所示；最后，将A和B&C&D&E合并组成root节点。最后得到编码如下表所示

Symbol	A	B	C	D	E
code	0	100	101	110	111

计算得到平均字长：[frac{1 bits*15+3 bits*(7+6+6+5)}{39 symbols} approx 2.23 bits per symbol]

3、实验

#include <stdio.h>
#include <malloc.h>

char word[10] = {‘A‘, ‘B‘, ‘C‘, ‘D‘, ‘E‘, ‘F‘, ‘G‘, ‘H‘, ‘I‘, ‘J‘};
int  weight[10] = {10, 9, 8, 7, 6, 5, 4, 3, 2, 1};

typedef struct HuffmanNode{
    int word;
    int weight;
    int parrent, lchild, rchild;
}HN;

HN *HT=NULL;

int Select(HN *H, int n, int *s1, int *s2)
{
    /*寻找权值最小节点*/
    /*先找到第一个没有父母节点的节点*/
    int i=0;
    for(; i<n; i++){
        if(H[i].parrent==0) {
            *s1 = i;
            break;
        }
    }
    /*寻找权值最小节点*/
    for(; i<n; i++){
        if(H[i].parrent == 0 && H[i].weight < H[*s1].weight){
            *s1=i;
        }
    }
    /*寻找权值次小节点*/
    int j=0;
    for(; j<n; j++){
        if(H[j].parrent==0 && *s1!=j) {
            *s2 = j;
            break;
        }
    }
    for(; j<n; j++){
        if(H[j].parrent == 0 && *s1!=j && H[j].weight < H[*s2].weight){
            *s2=j;
        }
    }
}

int creatHuffmanTree(int n)
{
    /*分配并初始化节点*/
    int m=2*n-1;
    HT = (HN*)malloc(sizeof(HN)*m);
    for(int i=0; i<m; i++) {
        HT[i].parrent=0;
        HT[i].lchild=0;
        HT[i].rchild=0;
    }
    for(int i=0; i<n; i++){
        HT[i].weight=weight[i];
        HT[i].word=word[i];
    }
    //printf("init is OK.
");
    /*构造Huffman树*/
    for(int i=n; i<m; i++){
        int s1, s2;
        /*寻找前i个数据中的最小值*/
        Select(HT, i, &s1, &s2);
        HT[i].weight=HT[s1].weight+HT[s2].weight;
        HT[i].lchild=s1;
        HT[i].rchild=s2;
        HT[s1].parrent=i;
        HT[s2].parrent=i;
        //printf("%d and %d have some parents %d!
", s1, s2, i);
    }
}


int main() {
    printf("Huffman coding test!
");
    creatHuffmanTree(10);
    for(int i=0; i<10; i++){
        int j=i;
        /*编码从叶子节点遍历到根节点，逆序输出编码*/
        while(HT[j].parrent != 0){
            if(HT[HT[j].parrent].lchild == j)
                printf("0");
            else if(HT[HT[j].parrent].rchild == j)
                printf("1");
            else {
                printf("ERROR! Parents‘ child is not me.");
                free(HT);
                return 1;
            }
            j=HT[j].parrent;
        }
        /*输出编码对应字符*/
        printf(" word is %c
", HT[i].word);
    }
    free(HT);
    return 0;
}

4、参考文献

1.Huffman Algorithm Wikipedia