（学习13）最短圆排列问题

Posted 2020-12-08 pipihoudewo

问题描述：

给定n个圆的半径序列，将它们放到矩形框中，各圆与矩形底边相切，求具有最小排列长度的圆排列。

问题解析：

根据示例：

 

 

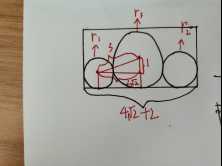
可得两个圆之间的距离计算为:sqrt((r₁+r₃)²-(r₁-r₃)²)，即 2*sqrt(r₁*r₃)

由于当前的圆不一定恰好与它前面的圆相切，故我们可以通过计算当前圆与其前面所有圆的距离，得到的最大结果即当前圆的横坐标值。

搜索树：叶子节点即所有结果集

 

 

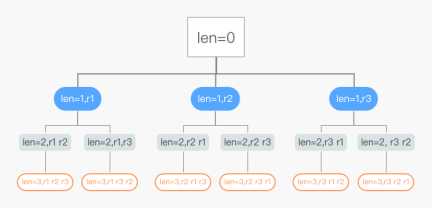
设计

计算方法:

核心伪代码

 

 1 double calCircleCenter(int n){//计算圆心坐标
 2     for(int i=1;i<n;i++){
 3        因为当前该圆可能与排在其前面的所有圆相切
 4        所以需要逐个计算与不同圆相切的情况。
 5     }
 6      返回圆心横坐标值
 7 }
 8 
 9 void calCircleSortLength(){//计算圆排列的总长度
10     double low=99999,high=0;
11 for(int i=1;i<=CIRCLENUM;i++){
12 //分别找出改序列的起点值与终点值
13 //该问题可以抽象成找出圆的横坐标减去其半径值，通过遍历所有圆，找出计算结果最小的，即第一个圆。而终点值即最后一个圆的横坐标值加上其半径值，得到的最大结果即该圆序列的终点值。
14         if(x[i]-r[i]<low){
15             low=x[i]-r[i];
16         }
17         if(x[i]+r[i]>high){
18             high=x[i]+r[i];
19         }
20     }
21     if(high-low<minLen){
22         minLen=high-low;
23         for(int i=1;i<=CIRCLENUM;i++){//保存当前的最短圆序列
24             circleSeq[i]=r[i];
25         }       
26     }
27 }
28 
29 
30 void findCircleSort(int n){ //查找圆排列
31 if(n==CIRCLENUM+1){
32 //若已经搜索完查找树的最后一层，说明已经形成一个结果
33 可以计算该结果的圆序列长度
34         calCircleSortLength();
35     }
36     for(int i=n;i<=CIRCLENUM;i++){//搜索所有可能性的结果
37         swap(r[n],r[i]);
38         double center=calCircleCenter(n);//获取圆当前的横坐标
39         x[n]=center;
40         findCircleSort(n+1);//向下继续搜索
41         swap(r[n],r[i]);//回溯
42     }
43 }

View Code

 

 

 

实现代码

 1 //
 2 //  main.cpp
 3 //  circleSort
 4 //
 5 //  Created by yizhihenpidehou on 2020/6/4.
 6 //  Copyright © 2020 yizhihenpidehou. All rights reserved.
 7 //
 8 
 9 #include <iostream>
10 #include <algorithm>
11 #include <stdio.h>
12 #include <string.h>
13 #include <cmath>
14 using namespace std;
15 const int CIRCLENUM =3;
16 double x[200];//存放每个圆的x轴位置
17 double r[200];//存放每个圆的半径
18 double circleSeq[200];//存放最短圆序列的半径
19 int minLen=999999;//存放最短圆排列长度
20 double calCircleCenter(int n){//计算圆心坐标
21     double maxx=0;
22     for(int i=1;i<n;i++){
23         int num=x[i]+2.0*sqrt(r[i]*r[n]);
24         if(num>maxx){
25             maxx=num;
26         }
27     }
28     return maxx;
29 }
30 void calCircleSortLength(){//计算圆排列的总长度
31     double low=99999,high=0;
32     for(int i=1;i<=CIRCLENUM;i++){
33         if(x[i]-r[i]<low){
34             low=x[i]-r[i];
35         }
36         if(x[i]+r[i]>high){
37             high=x[i]+r[i];
38         }
39     }
40     if(high-low<minLen){
41         minLen=high-low;
42         //printf("输出最短圆序列
");
43         for(int i=1;i<=CIRCLENUM;i++){
44             circleSeq[i]=r[i];
45         }
46       //  printf("
");
47     }
48 }
49 void findCircleSort(int n){ //查找圆排列
50     if(n==CIRCLENUM+1){
51         calCircleSortLength();
52     }
53     for(int i=n;i<=CIRCLENUM;i++){
54         swap(r[n],r[i]);
55         double center=calCircleCenter(n);//获取圆当前的横坐标
56         x[n]=center;
57         findCircleSort(n+1);//向下继续搜索
58         swap(r[n],r[i]);//回溯
59     }
60 }
61 int main(int argc, const char * argv[]) {
62     r[1]=1;r[2]=1;r[3]=2;//初始化半径
63     findCircleSort(1);
64     printf("最短圆序列:
");
65     for(int i=1;i<=CIRCLENUM;i++){//输出最短圆序列
66         printf("%f ",circleSeq[i]);
67     }
68     printf("
");
69     return 0;
70 }

View Code

 

算法复杂度

因为根据不同的排列方法取最小长度的圆排列，所以根据全排列公式要进行n!次查找，由于每次完成一个结果集后，还要找到起点值与终点值，即需要O((n+1)!)的时间复杂度

空间复杂度为O(n)，因为该算法中只用到几个一维数组