HDU 4652 Dice

Posted 2020-12-04 yuzao

Description

(m) 面的骰子,求:
1.出现 (n) 个连续的相同颜色的时候停止
2.出现 (n) 个连续的不同颜色的时候停止
的期望次数
题面

Solution

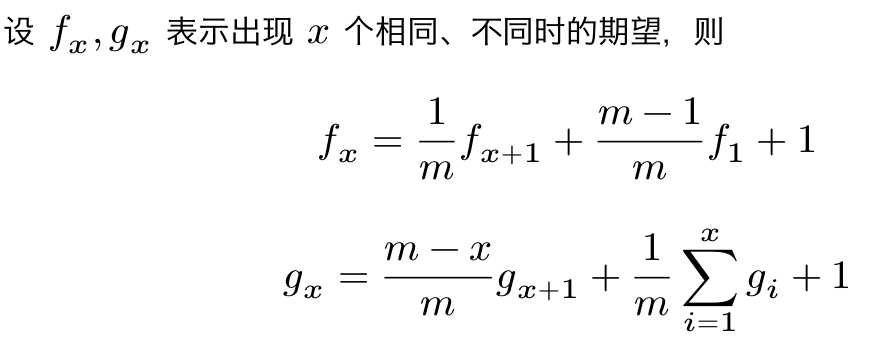
然后对于第一问,做差:
(f[i]=frac{1}{m}f[i+1]+frac{m-1}{m}f[1]+1)
(f[i+1]=frac{1}{m}f[i+2]+frac{m-1}{m}f[1]+1)
(f[i+1]-f[i]=frac{1}{m}(f[i+2]-f[i+1]))
求和之后得到 (f[n]-f[0]=frac{1-m^{n}}{1-m})
因为 (f[n]=0),所以代入就可以 (O(1)) 得到答案 (f[0])

对于第二问,同理:
(g[i+2]-g[i+1]=frac{m-i-1}{m}(g[i+1]-g[i]))
这个式子就不能 (O(1)) 算了,我们 (O(n)) 递推然后再求和就行了

#include<bits/stdc++.h>
using namespace std;
int n,m,op;
inline void work(){
    scanf("%d%d%d",&op,&m,&n);
    if(op==0)printf("%.9lf
",(1.0-pow(m,n))/(1.0-m));
    else{
        double t=1.0,ans=0;
        for(int i=1;i<=n;i++){
            ans+=t;
            t*=1.0*m/(m-i);
        }
        printf("%.9lf
",ans);
    }
}
int main(){
  freopen("pp.in","r",stdin);
  freopen("pp.out","w",stdout);
  int T;
  while(~scanf("%d",&T)){
      while(T--)work();
  }
  return 0;
}