2022-06-20:一个二维矩阵,上面只有 0 和 1,只能上下左右移动, 如果移动前后的元素值相同,则耗费 1 ,否则耗费 2。 问从左上到右下的最小耗费。 来自网易。3.27笔试。
Posted 福大大架构师每日一题
tags:
篇首语:本文由小常识网(cha138.com)小编为大家整理,主要介绍了2022-06-20:一个二维矩阵,上面只有 0 和 1,只能上下左右移动, 如果移动前后的元素值相同,则耗费 1 ,否则耗费 2。 问从左上到右下的最小耗费。 来自网易。3.27笔试。相关的知识,希望对你有一定的参考价值。
2022-06-20:一个二维矩阵,上面只有 0 和 1,只能上下左右移动,
如果移动前后的元素值相同,则耗费 1 ,否则耗费 2。
问从左上到右下的最小耗费。
来自网易。3.27笔试。
答案2022-06-20:
1.网上非常流行的方法,但这是错误的。这道题动态规划是做不了的。因为上下左右四个方向都可能走,而不是右下两个方向。
2.要用dijskra+小根堆才能实现。
代码里1和2两种方法都实现了,运行结果可以证明方法1是错误的。
代码用rust编写。代码如下:
use rand::Rng;
fn main()
let n: i32 = 100;
let m: i32 = 100;
let test_time: i32 = 10000;
println!("测试开始");
for i in 0..test_time
let mut map = random_matrix(n, m);
let ans1 = best_walk1(&mut map);
let ans2 = best_walk2(&mut map);
if ans1 != ans2
println!("出错了!", i);
println!("ans1 = ", ans1);
println!("ans2 = ", ans2);
break;
println!("测试结束");
// 一个错误的贪心
// 网上帖子最流行的解答,看似对,其实不行
fn best_walk1(map: &mut Vec<Vec<i32>>) -> i32
let n = map.len() as i32;
let m = map[0].len() as i32;
let mut dp: Vec<Vec<i32>> = vec![];
for i in 0..n
dp.push(vec![]);
for _ in 0..m
dp[i as usize].push(0);
for i in 1..m
dp[0][i as usize] = dp[0][(i - 1) as usize]
+ if map[0][(i - 1) as usize] == map[0][i as usize]
1
else
2
;
for i in 1..n
dp[i as usize][0] = dp[(i - 1) as usize][0]
+ if map[(i - 1) as usize][0] == map[i as usize][0]
1
else
2
;
for i in 1..n
for j in 1..m
dp[i as usize][j as usize] = dp[(i - 1) as usize][j as usize]
+ (if map[(i - 1) as usize][j as usize] == map[i as usize][j as usize]
1
else
2
);
dp[i as usize][j as usize] = get_min(
dp[i as usize][j as usize],
dp[i as usize][(j - 1) as usize]
+ (if map[i as usize][(j - 1) as usize] == map[i as usize][j as usize]
1
else
2
),
);
return dp[(n - 1) as usize][(m - 1) as usize];
fn get_min<T: Clone + Copy + std::cmp::PartialOrd>(a: T, b: T) -> T
if a < b
a
else
b
// 正确的解法
// Dijskra
fn best_walk2(map: &mut Vec<Vec<i32>>) -> i32
let n = map.len() as i32;
let m = map[0].len() as i32;
// 小根堆:[代价,行,列]
// 根据代价,谁代价小,谁放在堆的上面
let mut heap: Vec<Vec<i32>> = vec![];
// poped[i][j] == true 已经弹出过了!不要再处理,直接忽略!
// poped[i][j] == false 之间(i,j)没弹出过!要处理
let mut poped: Vec<Vec<bool>> = vec![];
for i in 0..n
poped.push(vec![]);
for _ in 0..m
poped[i as usize].push(false);
heap.push(vec![0, 0, 0]);
let mut ans = 0;
while heap.len() > 0
// 当前弹出了,[代价,行,列],当前位置
heap.sort_by(|a, b| b[0].cmp(&a[0]));
let cur = heap.pop().unwrap();
let dis = cur[0];
let row = cur[1];
let col = cur[2];
if poped[row as usize][col as usize]
continue;
// 第一次弹出!
poped[row as usize][col as usize] = true;
if row == n - 1 && col == m - 1
ans = dis;
break;
add(
dis,
row - 1,
col,
map[row as usize][col as usize],
n,
m,
map,
&mut poped,
&mut heap,
);
add(
dis,
row + 1,
col,
map[row as usize][col as usize],
n,
m,
map,
&mut poped,
&mut heap,
);
add(
dis,
row,
col - 1,
map[row as usize][col as usize],
n,
m,
map,
&mut poped,
&mut heap,
);
add(
dis,
row,
col + 1,
map[row as usize][col as usize],
n,
m,
map,
&mut poped,
&mut heap,
);
return ans;
// preDistance : 之前的距离
// int row, int col : 当前要加入的是什么位置
// preValue : 前一个格子是什么值,
// int n, int m :边界,固定参数
// map: 每一个格子的值,都在map里
// boolean[][] poped : 当前位置如果是弹出过的位置,要忽略!
// PriorityQueue<int[]> heap : 小根堆
fn add(
pre_distance: i32,
row: i32,
col: i32,
pre_value: i32,
n: i32,
m: i32,
map: &mut Vec<Vec<i32>>,
poped: &mut Vec<Vec<bool>>,
heap: &mut Vec<Vec<i32>>,
)
if row >= 0 && row < n && col >= 0 && col < m && !poped[row as usize][col as usize]
heap.push(vec![
pre_distance
+ if map[row as usize][col as usize] == pre_value
1
else
2
,
row,
col,
]);
// 为了测试
fn random_matrix(n: i32, m: i32) -> Vec<Vec<i32>>
let mut ans: Vec<Vec<i32>> = vec![];
for i in 0..n
ans.push(vec![]);
for _ in 0..m
ans[i as usize].push(rand::thread_rng().gen_range(0, 2));
return ans;
执行结果如下:
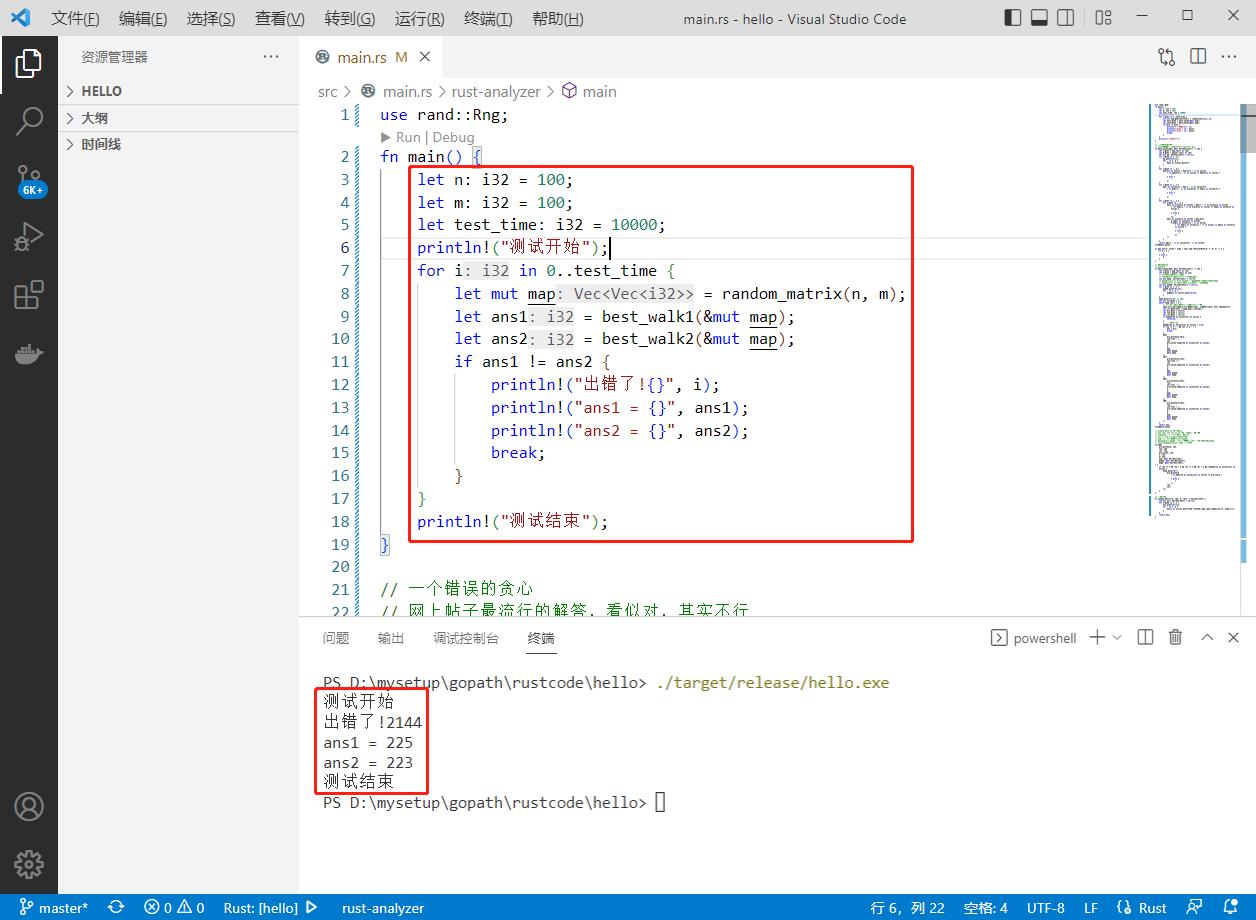
以上是关于2022-06-20:一个二维矩阵,上面只有 0 和 1,只能上下左右移动, 如果移动前后的元素值相同,则耗费 1 ,否则耗费 2。 问从左上到右下的最小耗费。 来自网易。3.27笔试。的主要内容,如果未能解决你的问题,请参考以下文章