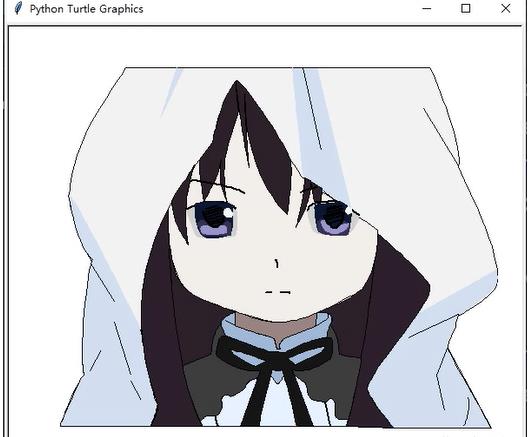
Python Turtle库 画魔法少女里的小圆晓美焰
Posted yunyun云芸
tags:
篇首语:本文由小常识网(cha138.com)小编为大家整理,主要介绍了Python Turtle库 画魔法少女里的小圆晓美焰相关的知识,希望对你有一定的参考价值。
第一步:
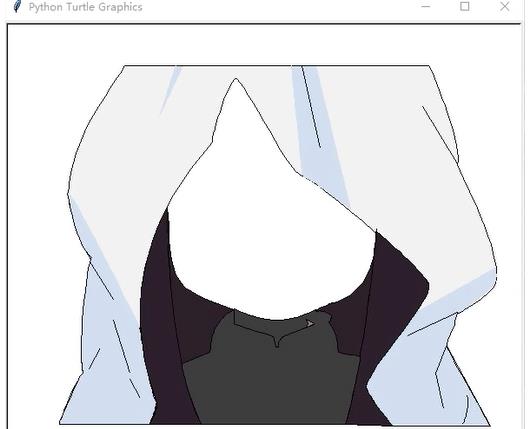
第二步:
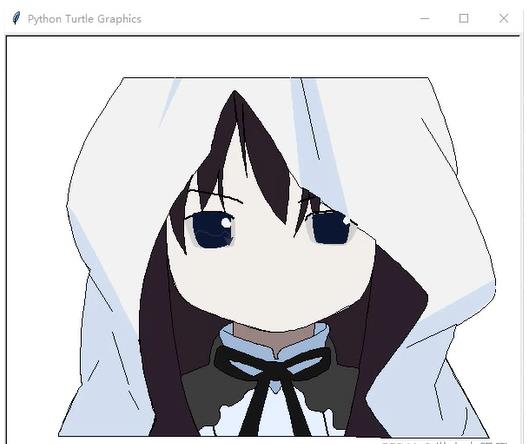
第三步:
①兼职交流,行业咨询、大佬在线专业解答有
②Python开发环境安装教程有
③Python400集自学视频有
④软件开发常用词汇有
⑤Python学习路线图有
⑥3000多本Python电子书有
如果你用得到的话可以直接拿走,Q群号: 675240729(纯技术交流和资源共享)以自助拿走。
源码:
import turtle as te
import time
WriteStep = 15 # 贝塞尔函数的取样次数
Speed = 5
Width = 600 # 界面宽度
Height = 500 # 界面高度
Xh = 0 # 记录前一个贝塞尔函数的手柄
Yh = 0
def Bezier(p1, p2, t): # 一阶贝塞尔函数
return p1 * (1 - t) + p2 * t
def Bezier_2(x1, y1, x2, y2, x3, y3): # 二阶贝塞尔函数
te.goto(x1, y1)
te.pendown()
for t in range(0, WriteStep + 1):
x = Bezier(Bezier(x1, x2, t / WriteStep),
Bezier(x2, x3, t / WriteStep), t / WriteStep)
y = Bezier(Bezier(y1, y2, t / WriteStep),
Bezier(y2, y3, t / WriteStep), t / WriteStep)
te.goto(x, y)
te.penup()
def Bezier_3(x1, y1, x2, y2, x3, y3, x4, y4): # 三阶贝塞尔函数
x1 = -Width / 2 + x1
y1 = Height / 2 - y1
x2 = -Width / 2 + x2
y2 = Height / 2 - y2
x3 = -Width / 2 + x3
y3 = Height / 2 - y3
x4 = -Width / 2 + x4
y4 = Height / 2 - y4 # 坐标变换
te.goto(x1, y1)
te.pendown()
for t in range(0, WriteStep + 1):
x = Bezier(Bezier(Bezier(x1, x2, t / WriteStep), Bezier(x2, x3, t / WriteStep), t / WriteStep),
Bezier(Bezier(x2, x3, t / WriteStep), Bezier(x3, x4, t / WriteStep), t / WriteStep), t / WriteStep)
y = Bezier(Bezier(Bezier(y1, y2, t / WriteStep), Bezier(y2, y3, t / WriteStep), t / WriteStep),
Bezier(Bezier(y2, y3, t / WriteStep), Bezier(y3, y4, t / WriteStep), t / WriteStep), t / WriteStep)
te.goto(x, y)
te.penup()
def Moveto(x, y): # 移动到svg坐标下(x,y)
te.penup()
te.goto(-Width / 2 + x, Height / 2 - y)
def line(x1, y1, x2, y2): # 连接svg坐标下两点
te.penup()
te.goto(-Width / 2 + x1, Height / 2 - y1)
te.pendown()
te.goto(-Width / 2 + x2, Height / 2 - y2)
te.penup()
def lineto(dx, dy): # 连接当前点和相对坐标(dx,dy)的点
te.pendown()
te.goto(te.xcor() + dx, te.ycor() - dy)
te.penup()
def Lineto(x, y): # 连接当前点和svg坐标下(x,y)
te.pendown()
te.goto(-Width / 2 + x, Height / 2 - y)
te.penup()
def Horizontal(x): # 做到svg坐标下横坐标为x的水平线
te.pendown()
te.setx(x - Width / 2)
te.penup()
def horizontal(dx): # 做到相对横坐标为dx的水平线
te.seth(0)
te.pendown()
te.fd(dx)
te.penup()
def vertical(dy): # 做到相对纵坐标为dy的垂直线
te.seth(-90)
te.pendown()
te.fd(dy)
te.penup()
te.seth(0)
def polyline(x1, y1, x2, y2, x3, y3): # 做svg坐标下的折线
te.penup()
te.goto(-Width / 2 + x1, Height / 2 - y1)
te.pendown()
te.goto(-Width / 2 + x2, Height / 2 - y2)
te.goto(-Width / 2 + x3, Height / 2 - y3)
te.penup()
def Curveto(x1, y1, x2, y2, x, y): # 三阶贝塞尔曲线到(x,y)
te.penup()
X_now = te.xcor() + Width / 2
Y_now = Height / 2 - te.ycor()
Bezier_3(X_now, Y_now, x1, y1, x2, y2, x, y)
global Xh
global Yh
Xh = x - x2
Yh = y - y2
def curveto_r(x1, y1, x2, y2, x, y): # 三阶贝塞尔曲线到相对坐标(x,y)
te.penup()
X_now = te.xcor() + Width / 2
Y_now = Height / 2 - te.ycor()
Bezier_3(X_now, Y_now, X_now + x1, Y_now + y1,
X_now + x2, Y_now + y2, X_now + x, Y_now + y)
global Xh
global Yh
Xh = x - x2
Yh = y - y2
def Smooth(x2, y2, x, y): # 平滑三阶贝塞尔曲线到(x,y)
global Xh
global Yh
te.penup()
X_now = te.xcor() + Width / 2
Y_now = Height / 2 - te.ycor()
Bezier_3(X_now, Y_now, X_now + Xh, Y_now + Yh, x2, y2, x, y)
Xh = x - x2
Yh = y - y2
def smooth_r(x2, y2, x, y): # 平滑三阶贝塞尔曲线到相对坐标(x,y)
global Xh
global Yh
te.penup()
X_now = te.xcor() + Width / 2
Y_now = Height / 2 - te.ycor()
Bezier_3(X_now, Y_now, X_now + Xh, Y_now + Yh,
X_now + x2, Y_now + y2, X_now + x, Y_now + y)
Xh = x - x2
Yh = y - y2
te.tracer(10)
te.setup(Width, Height, 0, 0)
te.pensize(1)
te.speed(Speed)
te.penup()
# 图层_2
time.sleep(20)
te.color("black", "#F2F2F2") # 外套
Moveto(61, 462)
te.begin_fill()
smooth_r(12, -41, 27, -58)
curveto_r(-6, -36, 6, -118, 9, -132)
curveto_r(-15, -27, -23, -51, -26, -74)
curveto_r(4, -66, 38, -105, 65, -149)
Horizontal(486)
curveto_r(12, 24, 40, 99, 33, 114)
curveto_r(39, 82, 55, 129, 39, 144)
smooth_r(-31, 23, -39, 28)
smooth_r(-12, 37, -12, 37)
lineto(50, 92)
Horizontal(445)
smooth_r(-29, -38, -31, -46)
smooth_r(78, -107, 72, -119)
Smooth(355, 178, 340, 176)
Smooth(272, 63, 264, 64)
smooth_r(-29, 67, -27, 73)
Curveto(99, 292, 174, 428, 173, 439)
smooth_r(-8, 23, -8, 23)
Lineto(61, 462)
te.end_fill()
Moveto(60.5, 461.5) # 阴影
te.color("black", "#D3DFF0")
te.begin_fill()
curveto_r(0, 0, 17, -42, 27, -59)
curveto_r(-6, -33, 6, -128, 10, -133)
curveto_r(-15, -10, -27, -66, -27.285, -75)
te.pencolor("#D3DFF0")
curveto_r(12.285, 11, 82.963, 156, 82.963, 156)
te.pencolor("black")
smooth_r(12.322, 75, 19.322, 86)
curveto_r(-1, 11, -8, 25, -8, 25)
Horizontal(60.5)
te.end_fill()
Moveto(444.5, 464)
te.begin_fill()
curveto_r(0, 0, -29, -36, -31, -46)
smooth_r(53.59, -82.337, 53.59, -82.337)
te.pencolor("#D3DFF0")
smooth_r(86.41, -47.663, 96.072, -54.85)
Curveto(563.5, 297.5, 570.5, 299.5, 518.5, 334)
te.pencolor("black")
curveto_r(-2, 16, -12, 33, -12, 37)
smooth_r(50, 92, 50, 93)
Horizontal(444.5)
te.end_fill()
Moveto(195, 49)
te.begin_fill()
te.pencolor("#D3DFF0")
polyline(195, 49, 175.5, 106.5, 202.522, 49)
te.pencolor("black")
Horizontal(195)
te.pencolor("#D3DFF0")
te.end_fill()
Moveto(327.997, 49)
te.begin_fill()
te.pencolor("#D3DFF0")
curveto_r(0, 0, 11.503, 121.087, 13.503, 128.087)
curveto_r(11, 2, 54, 37, 54, 37)
lineto(-40, -165.087)
te.pencolor("black")
Horizontal(327.997)
te.pencolor("#D3DFF0")
te.end_fill()
te.pencolor("black")
line(94.5, 397.5, 107.5, 373.5) # 皱纹
line(122.5, 317.5, 95.875, 274.699)
line(122.5, 341.5, 141.5, 402.5)
line(141.5, 409.5, 153.5, 431.5)
# line(328,47.712,344,175.977)
line(340.023, 49, 360.5, 144)
# line(353.5,47.5,395.5,208.5)
line(478.5, 95.5, 518.5, 161.5)
line(518.5, 332.5, 460.5, 359.5)
polyline(506.5, 369.5, 493.5, 402.5, 502.5, 443.5)
Moveto(530, 429)
curveto_r(4, 16, -5, 33, -5, 33)
# 图层_3
te.color("black", "#2b1d2a") # 外套内侧
Moveto(225, 462)
te.begin_fill()
Horizontal(165)
smooth_r(9, -15, 8, -25)
curveto_r(-47, -126, 6, -212, 12, -225)
Curveto(185, 305, 202, 428, 225, 462)
Lineto(225, 462)
te.end_fill()
Moveto(390, 462)
te.begin_fill()
curveto_r(10, -23, 34, -180, 35, -222) # !!!227
curveto_r(7, 4, 54, 45, 61, 61) # 61
smooth_r(-73, 101, -72, 118)
curveto_r(5, 15, 31, 46, 31, 45)
Lineto(390, 462)
te.end_fill()
# 图层_4
te.color("black", "#2b1d29") # 外套内侧
Moveto(225, 462)
te.begin_fill()
curveto_r(-28, -50, -40, -166, -40, -250)
curveto_r(6, 51, -6, 87, 45, 106)
smooth_r(Python Turtle库 画魔法少女里的小圆晓美焰