解密树的平衡：二分搜索树 → AVL自平衡树 → 红黑树

Posted 2021-12-24 Debroon

tags:

篇首语：本文由小常识网(cha138.com)小编为大家整理，主要介绍了解密树的平衡：二分搜索树 → AVL自平衡树 → 红黑树相关的知识，希望对你有一定的参考价值。

解密树的平衡：二分搜索树 → AVL自平衡树 → 红黑树

二叉树框架
二分搜索树
验证
查找
添加
删除

AVL平衡树
插入
删除

红黑树

二叉树框架

二叉树或者应该称为，二叉链表树。

每个节点都最多有两个叉的树
实现树的底层结构是链表

所以，二叉树和链表会有很多相同之处。

链表遍历框架：

class ListNode 
	int val;
	ListNode next;


void traverse( ListNode head ) 
	for( ListNode p = head; p != NULL; p = p.next )   // 迭代遍历
		do ···


void traverse( ListNode head )                       // 前序遍历
	print(head.val);
	traverse(head.next);


void traverse( ListNode head )                       // 后序遍历
	traverse(head.next);
	print(head.val);

二叉树遍历框架：

class TreeNode 
	int val;
	TreeNode left, right;


void traverse( TreeNode root )                        // 前序遍历
	print(root.val);
	traverse(root.left);
	traverse(root.right);


void traverse( TreeNode root )                        // 中序遍历
	traverse(root.left);
	print(root.val);
	traverse(root.right);


void traverse( TreeNode root )                        // 后序遍历
	traverse(root.left);
	traverse(root.right);
	print(root.val);

二叉树遍历框架拓展为多叉树遍历框架：

class TreeNode 
	int val;
	TreeNode[] children;


void traverse( TreeNode root ) 
	for( TreeNode child: root.children )
		traverse(child);

我们把这二叉树框架映入脑海里，下文的二分搜索树就是二叉树的延伸。

二分搜索树

数组的查找快，插入、删除慢，链表的插入、删除快，查找慢。

高效率的动态修改和高效率的静态查找，究竟能否同时兼顾？

通过对二分查找策略的抽象与推广，定义并实现二分搜索树结构，兼顾了：

链表插入的灵活性（直接插入即可，不需要挪动其他元素）
数组查找的高效性（二分查找）

插入、删除、查询操作均为 $O (l o g n)$ 。

实现二分搜索树，满足以下俩个条件：

若它的左子树不为空，左子树上所有节点的值都小于它的根节点。
若它的右子树不为空，右子树上所有的节点的值都大于它的根节点。

struct TreeNode 
	int val;
	TreeNode *left;
	TreeNode *right;
	
	TreeNode() : val(0), left(nullptr), right(nullptr) 
	TreeNode(int x) : val(x), left(nullptr), right(nullptr) 
	TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) 
;

验证

请打开题目：

验证二分搜索树的合法性：98. 验证二叉搜索树

按照二叉树框架：

void traverse( TreeNode root )                        
	/* 明确一个节点（root） 要做的事情 */

	// 其他部分，交给递归
	traverse(root.left);
	traverse(root.right);

按照二分搜索树的定义，一个节点自己要做的事情不就是比左孩子大，比右孩子小吗！

class Solution 
public:
    bool isValidBST(TreeNode* root) 
    	/* 明确一个节点（root） 要做的事情 */
		if( !root )                                         // 定义规定：空也是一颗二分搜索树
			return true;  
		if( root->left && root->val <= root->left->val )    // 定义规定：根节点 > 左孩子
			return false;
		if( root->right && root->val >= root->right->val )  // 定义规定：根节点 < 右孩子
			return false;

		// 其他部分，交给递归
		return isValidBST(root.left) && isValidBST(root.right);
    
;

提交上去，发现居然不对。

二分搜索树的定义，不只是：

根节点 > 左孩子
根节点 < 右孩子

还有：

左子树上所有节点的值都小于它的根节点
右子树上所有的节点的值都大于它的根节点

比如：

   10
   / \\
  5   15
     / \\
    6   20
P.S. 6 处于根节点的右子树，按照定义，凡右子树任何的子节点都要大于根节点 10

那么一个节点要做的事，不仅是和俩个孩子节点比较，还得和整个左子树、右子树的所有节点比较。

但其实我们也没必要和子树里面所有节点都比较，只需要知道一个子树的上界、下界，如果比较节点的值与 Min&Max，在范围外则 return false。

这个方法最关键的是理解对于每个节点来说，什么是他的 Min&Max。

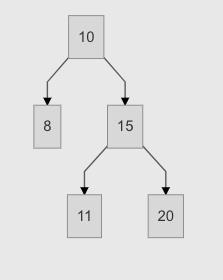
以上图的树为例进行分析：

从 10 开始，因为是 root，所以可以为任何值。也就是在【Integer.MIN_VALUE, Integer.MAX_VALUE】范围内。
递归到 10 的右子树 15，15 的范围是多少呢？首先需要比 parent 要大，也就是 >10。上边界呢？没有改变，可以非常大，只要 >10 就行了。所以范围是【10+1，Integer.MAX_VALUE】。
递归到 15 的右子树 20，20 的范围是多少呢？首先需要比 parent 要大，也就是>15。上边界呢？没有改变，可以非常大，只要 >16 就行了。所以范围是【15+1，Integer.MAX_VALUE】。
递归到 15 的左子树 11，11 的范围是多少呢？首先需要比 parent 要小，也就是 <15。下边界呢？没有改变。所以范围是【11，15-1】。

class Solution 
public:
	bool isValidBST(TreeNode* root) 
    	return isValidBST(root, LONG_MIN, LONG_MAX);
    	// 使用 INT_MAX 会溢出，改用 LONG_MAX
  	

	// 因为函数参数列表要增加参数，需要使用辅助函数
	bool isValidBST(TreeNode* root, long long min, long long max) 
    	if( !root )       // 节点为空，则说明一直到此的节点都在边界范围内，树没有问题
    		return true;
    
    	if( root->val <= min || root->val >= max )
        	return false;
        
    	return isValidBST(root->left, min, root->val) && isValidBST(root->right, root->val, max);
    	// 节点不是null，那么继续开始递归到左右子树（更新Min&Max）
    	// 并且只有他的左右子树都能通过测试时，这个节点才能通过测试。所以用 &&
    
;

查找

按照二叉树框架：

void traverse( TreeNode root )                        
	/* 明确一个节点（root） 要做的事情 */

	// 其他部分，交给递归
	traverse(root.left);
	traverse(root.right);

在二分搜索树中查找一个数是否查找：

int target;
bool isInBST( TreeNode root ) 
	/* root 该做的事 */
	if( !root )
		return false;
	if( root->val == target )
		return true;

	// 递归框架
	return isInBST(root->left) || isInBST(root->right);

大框架是如此，此外 BST 还有 “左小右大” 的特点。

bool isInBST( TreeNode root, int target ) 
	/* root 该做的事 */
	if( !root )
		return false;
	if( root->val == target )
		return true;

	// 二分递归：一次排除一半
	if( root->val < target )
		return isInBST(root->right, target);
	if( root->val > target )
		return isInBST(root->left, target);

请打开题目：

700. 二叉搜索树中的搜索

class Solution 
public:
    TreeNode* searchBST(TreeNode* root, int val) 
        if (root == NULL || root->val == val) return root;
        return root->val > val ? searchBST(root->left, val) : searchBST(root->right, val);
    
;

添加

在二分搜索树中插入一个数。

// 涉及到改，函数应该返回 TreeNode 类型，通过函数返回值接收
TreeNode insertIntoBST(TreeNode root, int val) 
	if( !root )                                             // 找到空位置
		return new TreeNode(val);                           // 插入新节点
	if( root->val == val )                                  // 已存在
		return root;							            // 不重复，直接返回
	if( root->val < val )						            // val 大
		root->right = insertIntoBST( root->right, val );    // 则插到右子树
	if( root->val > val )                                   // val 小
		root->left = insertIntoBST( root->left, val );      // 则插到左子树

请打开题目：

701. 二叉搜索树中的插入操作

class Solution 
public:
    TreeNode* insertIntoBST(TreeNode* root, int val)       // 俩个条件表达式嵌套
        return !root ? new TreeNodeval : 
        (val < root->val ? root->left = insertIntoBST( root->left, val) : 
        root->right = insertIntoBST(root->right, val) , root);
    
;

删除

先写出删除框架：

TreeNode deleteNode(TreeNode root, int key) 
	if( root->val == key )
		// 找到了，删除
	else if( root->val > key )	
		root->left = deleteNode(root->left, key);        // 去左子树寻找 key
	else if( root->val < key )
		root->right = deleteNode(root->right, key);      // 去右子树寻找 key
	return root;

删除有三种情况：

// 第一种：删除的是末端节点，没有孩子节点
if( !root->left && !root->right )
	return NULL;

// 第二种：待删除节点有一个子节点
if( !root->left )
	return root->right;
if( !root->right )
	return root->left;

// 第三种，待删除节点有俩个子节点，必须找到左子树最大节点，或者右子树最小节点，这里找右子树最小节点
if( root->left && root->right )
	TreeNode minNode = getMin(root->right);                   // 找到右子树的最小节点
	root->val = minNode->val;                                 // 最小节点上移到 root
	root->right = deleteNode(root->right, minNode->val);      // 删除最小节点

完整代码：

TreeNode deleteNode(TreeNode root, int key) 
	if( !root )
		return NULL;
	if( root->val == key ) 
		// 第二种：待删除节点有一个子节点，包含了第一种情况
		if( !root->left ) return root->right;
		if( !root->right ) return root->left; 
		
		// 左右孩子都存在，第三种情况
		TreeNode minNode = getMin(root->right);               // 找到右子树的最小节点
		root->val = minNode->val;                             // 最小节点上移到 root
		root->right = deleteNode(root->right, minNode->val);  // 删除最小节点
	
			
	else if( root->val > key )	
		root->left = deleteNode(root->left, key);             // 去左子树寻找 key
	else if( root->val < key )
		root->right = deleteNode(root->right, key);           // 去右子树寻找 key
	return root;


TreeNode getMin(TreeNode node) 
	while( !node->left )                                      // 最左边的就是最小的
		node = node->left;
	return node;

无论是增、删、改、查：

二叉树的总体设计思路，把当前节点要做的事做好，其他的抛给递归框架，不用操心
如果当前节点会对下面的子节点有整体影响，可通过辅助函数增长参数列表，借助参数传递信息

请打开题目：

450. 删除二叉搜索树中的节点

class Solution 
public:
    TreeNode* deleteNode(TreeNode* root, int key) 
        if (root == nullptr)    
        	return nullptr;
        if (key > root->val)    
        	root->right = deleteNode(root->right, key);       // 去右子树删除
        else if (key < root->val)    
        	root->left = deleteNode(root->left, key);         // 去左子树删除
        else                                                  // 当前节点就是要删除的节点
        
            if (! root->left)   
            	return root->right;                           // 情况1，欲删除节点无左子
            if (! root->right)  
            	return root->left;                            // 情况2，欲删除节点无右子
            TreeNode* node = root->right;                     // 情况3，欲删除节点左右子都有 
            
            while (node->left)                                // 寻找欲删除节点右子树的最左节点
                node = node->left;
            node->left = root->left;                          // 将欲删除节点的左子树成为其右子树的最左节点的左子树
            root = root->right;                               // 欲删除节点的右子顶替其位置，节点被删除
        
        return root;    
    
;

AVL平衡树

平衡树：对于任意一个节点，左子树与右子树的高度差不能超过 1。

所以，平衡树的成员还需要添加一个高度 height 以及计算出每个节点的平衡因子（左右子树高度差）是多少。

// 计算每个节点的平衡因子
int getBalanceFactor(TreeNode *root) 
	if( !root )
		return 0;
	return getBalanceFactor(root.left) - getBalanceFactor(root.right);

插入

在插入时，平衡树可能就不平衡了。

#include<bits/stdc++.h>
using namespace std;

class Node 
	public:
	int key;
	Node *left;
	Node *right;
	int height;
;

int max(int a, int b);

int height(Node *N) 
	if (N == NULL)
		return 0;
	return N->height;


int max(int a, int b)  return (a > b)? a : b; 

Node* newNode(int key) 
	Node* node = new Node();
	node->key = key;
	node->left = NULL;
	node->right = NULL;
	node->height = 1; 
	return(node);


Node *rightRotate(Node *y) 
	Node *x = y->left;
	Node *T2 = x->right;

	x->right = y;
	y->left = T2;
	y->height = max(height(y->left), height(y->right)) + 1;
	x->height = max(height(x->left), height(x->right)) + 1;
	return x;


Node *leftRotate(Node *x) 
	Node *y = x->right;
	Node *T2 = y->left;

	y->left = x;
	x->right = T2;
	x->height = max(height(x->left), height(x->right)) + 1;
	y->height = max(height(y->left), height(y->right)) + 1;

	return y;


int getBalance(Node *N) 
	if (N == NULL)
		return 0;
	return height(N->left) - height(N->right);


Node* insert(Node* node, int key) 
	if (node == NULL)
		return(newNode(key));

	if以上是关于解密树的平衡：二分搜索树 → AVL自平衡树 → 红黑树的主要内容，如果未能解决你的问题，请参考以下文章 
 解密树的平衡：二分搜索树 → AVL自平衡树 → 红黑树
 13-自平衡二分搜索树 AVLTree
 数据结构AVL
 数据结构AVL
 十三彻底搞懂平衡二叉树AVL
 动画 | 什么是平衡二分搜索树（AVL）？