Java集合数据结构——优先级队列 (堆PriorityQueue)

Posted 2021-09-10 爱敲代码的三毛

文章目录

• 一、二叉树的顺序存储
• • 1. 存储方式
  • 2. 父亲和孩子的下标关系
• 二、堆(heap)
• • 1. 堆的基本概念
  • 2. 堆的操作
  • • (1) 建堆（向下调整）
    • (2) 建堆的时间复杂度
    • (3) 入队（向上调整）
    • (4) 出队（向下调整）
• 三、Java中的优先级队列（PriorityQueue）
• 四、堆排序
• 完！

---

一、二叉树的顺序存储

1. 存储方式

使用数组保存二叉树结构，方式即将二叉树用层序遍历方式放入数组中。
一般只适合表示完全二叉树，因为非完全二叉树会有空间的浪费。
这种方式的主要用法就是堆的表示

2. 父亲和孩子的下标关系

已知父亲(parent)的下标

• 左孩子(left)下标 = 2 * parent + 1;
• 右孩子(right)下标 = 2 * parent + 2;

已知孩子（不区分左右）(child)下标

• 父亲ji(parent)下标 = (child - 1) / 2;

二、堆(heap)

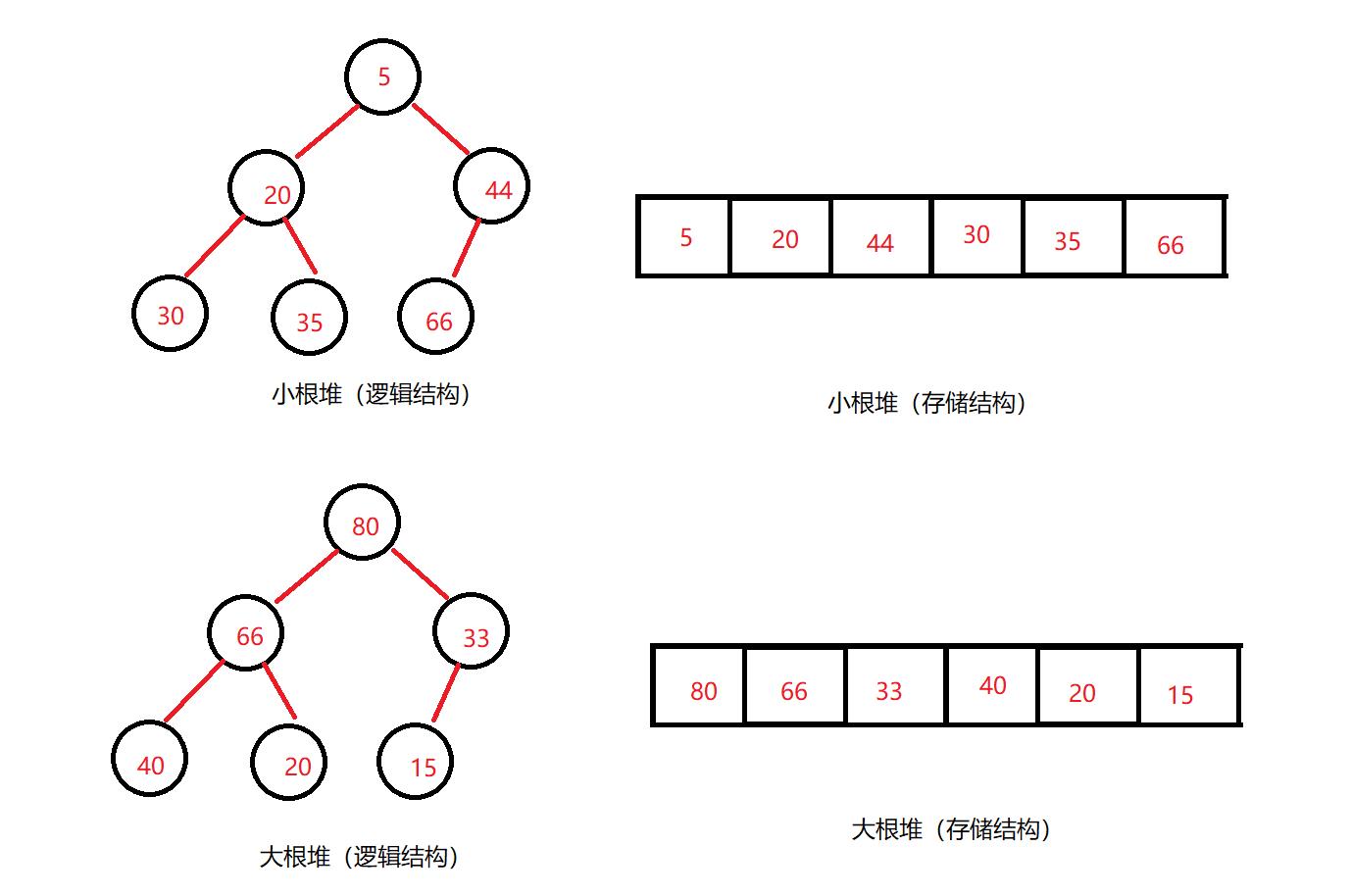
1. 堆的基本概念

1. 堆逻辑上是一棵完全二叉树
2. 堆物理上是保存在数组中
3. 满足任意结点的值都大于其子树中结点的值，叫做大堆，或者大根堆，或者最大堆
4. 反之，则是小堆，或者小根堆，或者最小堆
5. 堆的基本作用是，快速找集合中的最值

2. 堆的操作

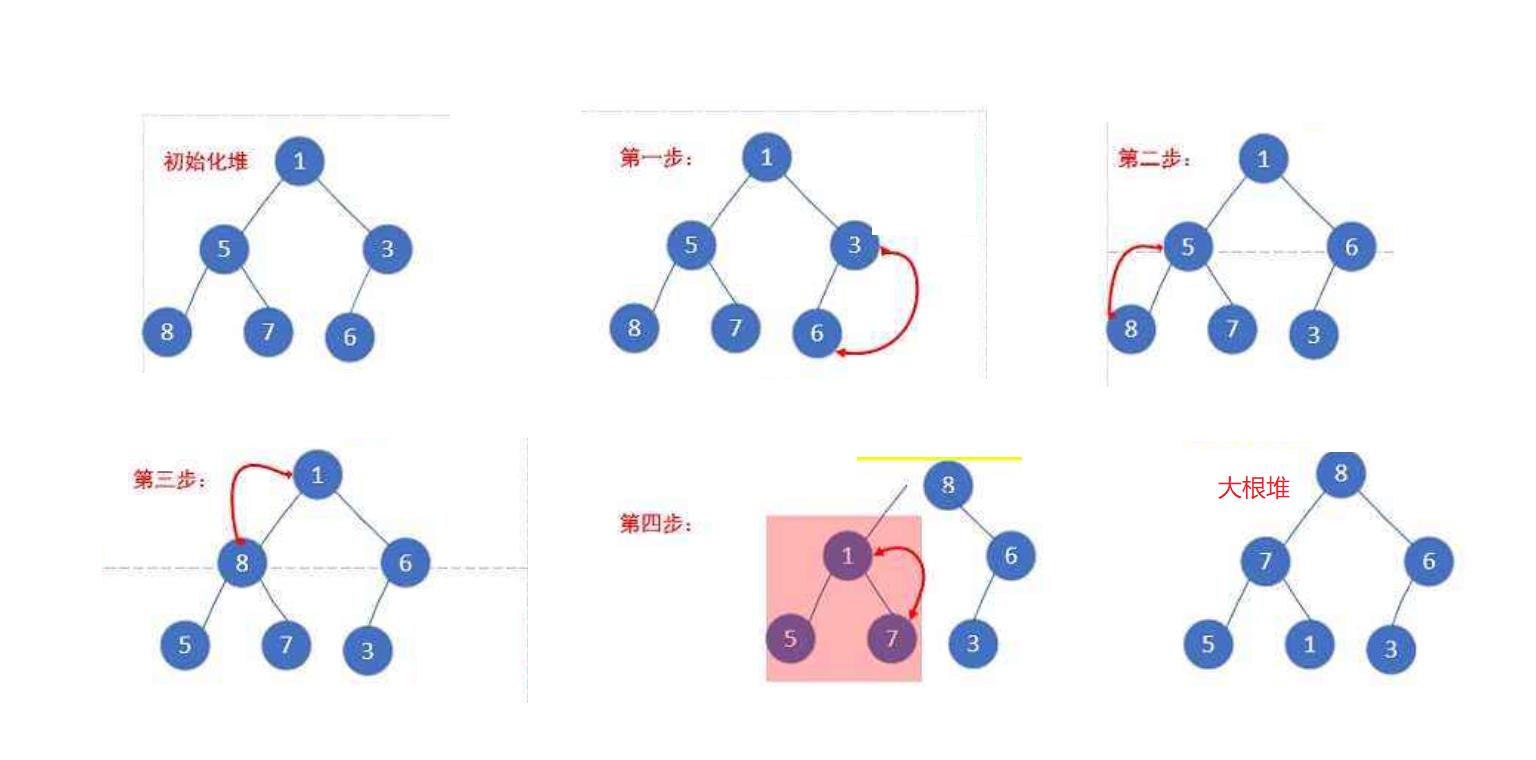
(1) 建堆（向下调整）

下面我们给出一个数组，这个数组逻辑上可以看做一颗完全二叉树，但是还不是一个堆，现在我们通过算法，把它构建成一个堆。根节点左右子树不是堆，我们怎么调整呢？这里我们从倒数的第一个非叶子节点的子树开始调整，一直调整到根节点的树，就可以调整成堆。

向上调整的思路：从堆的最后一个元素的父亲。左右孩子比较大小后再和父亲比较如果大就交换。再更新父亲和孩子的下标，直到当所有孩子都没有父亲大时就是大根堆了。

代码示例：

public class TestHeap {
    public int[] elem;
    public int usedSize;

    TestHeap() {
        this.elem = new int[10];
    }

    /**
     * 建大堆
     * @param array
     */
    public void creteHeap(int[] array) {
        for (int i = 0; i < array.length; i++) {
            this.elem[i] = array[i];
            this.usedSize++;
        }
        for (int parent = (array.length-1-1)/2; parent >= 0; parent--) {
            adjustDown(parent,this.usedSize);//向上调整
        }
    }
    public void adjustDown(int root,int len) {
        int parent = root;
        int child = 2*parent+1;

        while (child < len) {
            //判断是否有右孩子
            if(child+1 < len && this.elem[child] < this.elem[child+1]) {
                child++;
            }
            //如果左右孩子的最大值大于父亲的值就交换
            if(this.elem[parent] < this.elem[child]) {
                int tmp = this.elem[parent];
                this.elem[parent] = this.elem[child];
                this.elem[child] = tmp;
                parent = child;
                child = 2*parent+1;
            }else {
                break;
            }
        }
    }
}

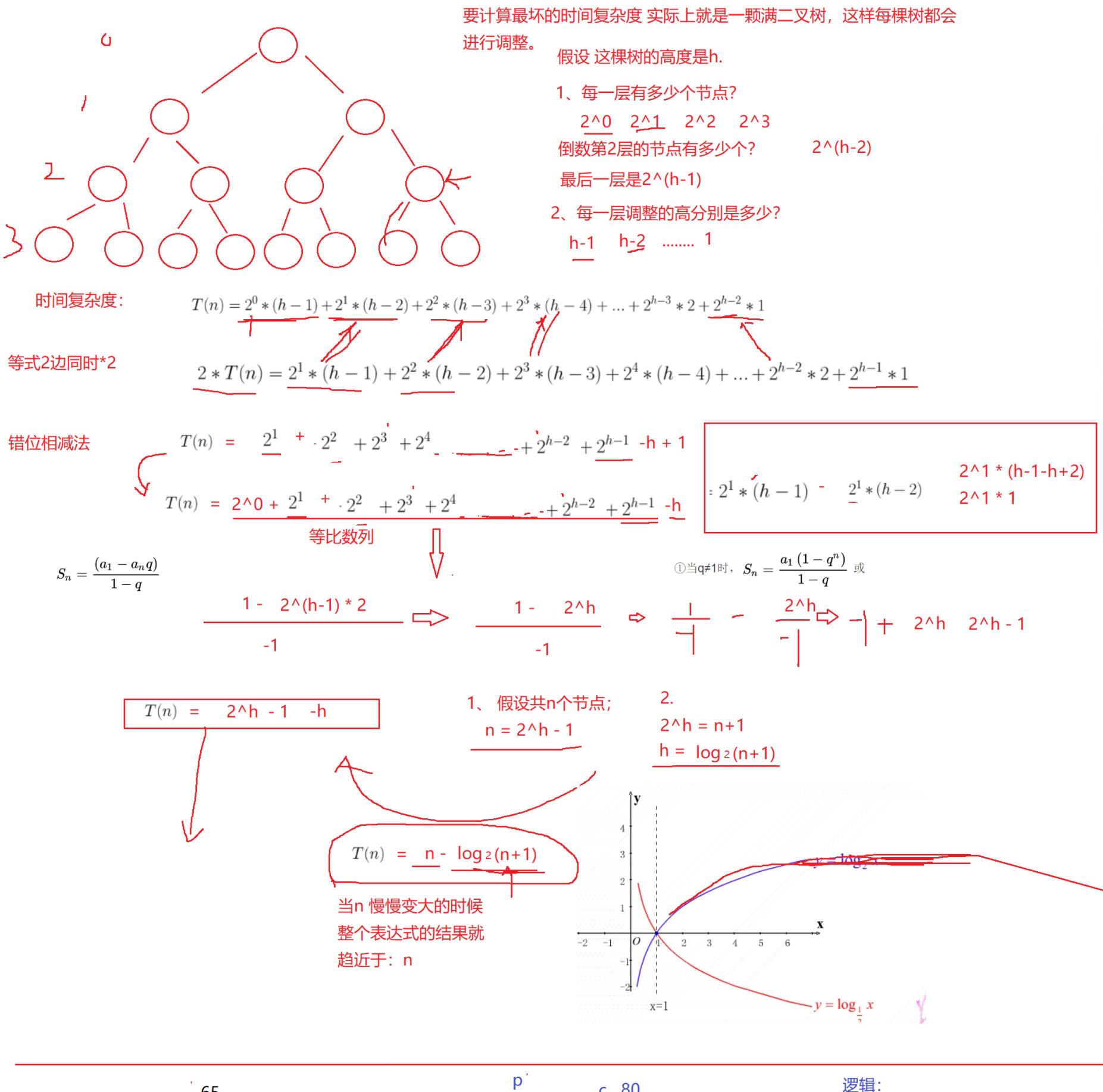
(2) 建堆的时间复杂度

通过公式一步计算得到： n - log₂(n + 1)
当n慢慢变大，时间复杂度就是 O(n)
建堆的时间复杂度

(3) 入队（向上调整）

//向上调整
public void adjustUp(int child) {
        int parent = (child-1)/2;
        while(parent >= 0) {
            if(this.elem[parent] < this.elem[child]) {
                int tmp = this.elem[parent];
                this.elem[parent] = this.elem[child];
                this.elem[child] = tmp;
                child = parent;
                parent = (child-1)/2;
            } else {
                break;
            }
        }
    }

    /**
     * 入队
     * @param val
     */
    public void push(int val) {
        //扩容
        if(this.isFull()) {
            this.elem = Arrays.copyOf(this.elem,this.elem.length*2);
        }
        this.elem[this.usedSize] = val;
        this.usedSize++;
        adjustUp(this.usedSize-1);
    }

(4) 出队（向下调整）

让堆顶元素和堆的最后一个元素交换，元素个数减1。再从0下标向下调整

	/**
     * 出队首元素
     */
    public void pop() {
        if(this.isEmpty()) return;

        int tmp = this.elem[0];
        this.elem[0] = this.elem[this.usedSize-1];
        this.elem[this.usedSize-1] = tmp;
        this.usedSize--;
        this.adjustDown(0,this.usedSize);//堆顶和堆尾下标
    }
    public void adjustDown(int root,int len) {
        int parent = root;
        int child = 2*parent+1;
        while(child < len) {
            //找到左右孩子的最大值
            //1、前提是你得有右孩子
            if(child+1 < len && this.elem[child] < this.elem[child+1]) {
                child++;
            }
            //保证，child下标的数据  一定是左右孩子的最大值的下标
            if(this.elem[child] > this.elem[parent]) {
                int tmp = this.elem[child];
                this.elem[child] = this.elem[parent];
                this.elem[parent] = tmp;
                parent = child;
                child = 2*parent+1;
            }else {
                break;
            }
        }
    }
    public boolean isEmpty() {
        return this.usedSize == 0;
    }

三、Java中的优先级队列（PriorityQueue）

Java中的PriorityQueue默认是一个小堆，需要指定比较规则才能改为大堆。下篇博客会讲到。

 PriorityQueue<List<Integer>> heap = new PriorityQueue<>();

四、堆排序

从大到小：建一个小堆
从小到大：建一个大堆

时间复杂度：O(n*logN)
空间复杂度：O(1)
稳定性：不稳定

这里不细说，到后面常见排序算法中还会提到。

	/**
     * 堆排序
     * 先交换第一个和最后一个元素，再调整树
     * 从小到大排序
     */
    public void heapSort() {
        if(this.isEmpty()) return;

        int len = this.usedSize-1;
        while(len > 0) {
            int tmp = this.elem[len];
            this.elem[len] = this.elem[0];
            this.elem[0] = tmp;
            adjustDown(0,len);
            len--;
        }
    }
    public void adjustDown(int root,int len) {
        int parent = root;
        int child = 2*parent+1;

        while (child < len) {
            //判断是否有右孩子
            if(child+1 < len && this.elem[child] < this.elem[child+1]) {
                child++;
            }
            //如果左右孩子的最大值大于父亲的值就交换
            if(this.elem[parent] < this.elem[child]) {
                int tmp = this.elem[parent];
                this.elem[parent] = this.elem[child];
                this.elem[child] = tmp;
                parent = child;
                child = 2*parent+1;
            }else {
                break;
            }
        }

---

完！