用booth算法求[x*y]补。x=0.1101,y=-0.1010

Posted 2023-03-18

参考技术A 首先编程把原码变为补码，这个资料很多，可以查看
booth乘法的源码是：
library IEEE;
use IEEE.STD_LOGIC_1164.ALL;
use IEEE.STD_LOGIC_ARITH.ALL;
use IEEE.STD_LOGIC_UNSIGNED.ALL;

---- Uncomment the following library declaration if instantiating
---- any Xilinx primitives in this code.
--library UNISIM;
--use UNISIM.VComponents.all;

entity mp is
Port ( ai : in std_logic_vector(7 downto 0);
bi : in std_logic_vector(7 downto 0)
done : out std_logic;
clk : in std_logic;
op : out std_logic_vector(15 downto 0));
end mp;
architecture Behavioral of mp is
begin

process(ai,bi,clk)
variable a,b,m : std_logic_vector( 7 downto 0);
variable cp: std_logic_vector( 1 downto 0);
variable t: std_logic ;
variable counter: integer;
begin
if clk'event and clk='1' then
counter:=0;
t:='0';
a:=ai;
b:=bi;
m:="00000000";
cp:=b(0)&'0';
done<='0';
while counter<8 loop
case cp is
when "10"=> m:=m-a;
when "01"=> m:=m+a;
when others=>m:=m;
end case;
t:=b(0);
b:=m(0)&b(7 downto 1);
m:=m(7)&m(7 downto 1);
cp:=b(0)&t;
counter:=counter+1;
end loop;
op<= m&b;
done<='1';
end if;
end process;
end Behavioral;
这个是8位乘法器，你可以稍作修改本回答被提问者采纳

计算机组成原理——补码乘法运算

文章目录

• 补码的一位乘法（Booth算法）
• • 手算模拟
  • • 例题
    • 做题总结
• 原码，补码一位乘法的对比

补码的一位乘法（Booth算法）

1. 进行 n 轮加法、移位，最后再多来一次加法
2. 每次加法可能 +0 、+[x]补、+[-x]补
3. 每次移位是“补码的算数右移”
4. 符号位参与运算

在第二个步骤中，需要根据MQ中的最低位、辅助位 来确定加什么：

• 辅助位 - MQ中最低位 = 1时，(ACC)+[x]补
• 辅助位 - MQ中最低位 = 0时，(ACC)+0
• 辅助位 - MQ中最低位 = -1时，(ACC)+[-x]补

手算模拟

例题

设机器字长为5位（含1位符号位，n=4），x = −0.1101，y = +0.1011，采用Booth算法求x·y

解：手动计算是这样

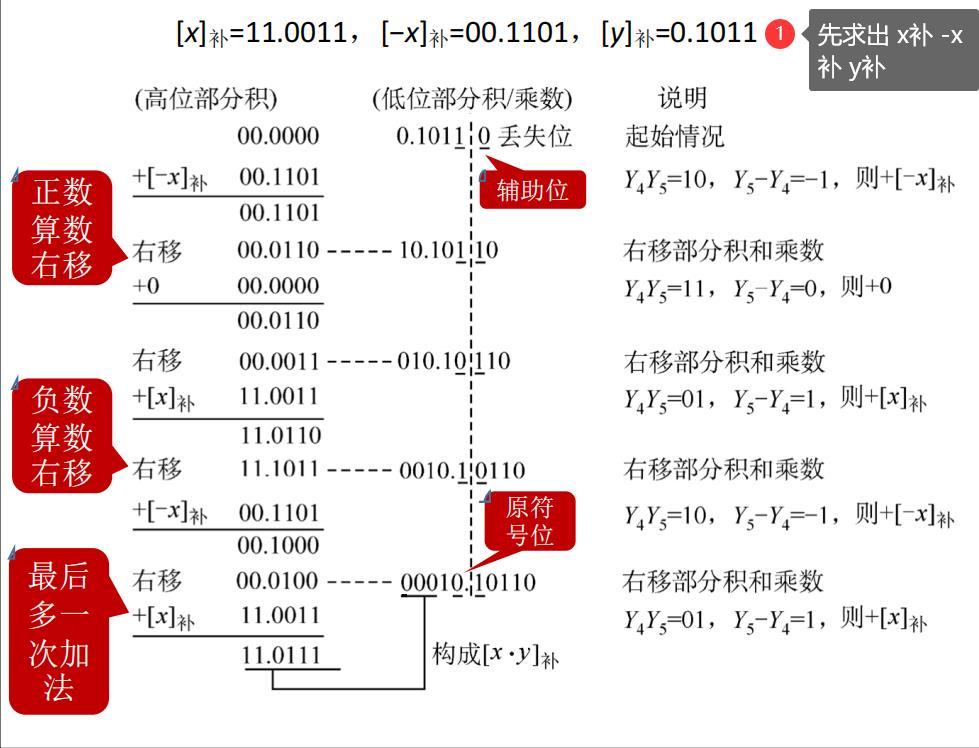
最后得 [x·y]补 = 11.01110001
即x·y = −0.10001111

做题总结

1. n轮加法、算数右移，加法规则如下：
   辅助位 - MQ中最低位 = 1时，(ACC)+[x]补
   辅助位 - MQ中最低位 = 0时，(ACC)+0
   辅助位 - MQ中最低位 = -1时，(ACC)+[-x]补
2. 补码的算数右移：
   符号位不动，数值位右移，正数右移补0，
   负数右移补1（符号位是啥就补啥）
3. 一般来说，Booth算法的被乘数、部分积采用双符号位补码

原码，补码一位乘法的对比

原码一位乘法：	补码一位乘法：
进行 n 轮加法、移位	进行 n 轮加法、移位，最后再多来一次加法
每次加法可能 +0、+[|x|]原	每次加法可能 +0、+[x]补、+[-x]补
每次移位是“逻辑右移”	每次移位是“补码的算数右移”
符号位不参与运算	符号位参与运算