优美的曲线-译

Posted 2023-01-23 zhangrelay

来源：Some equations that describe beautiful curves

链接：

1. iviveros.github.io/param-eq/#introduction

2. mathworld.wolfram.com/ButterflyCurve.html

---

介绍
在本文中，我收集了一些生成我喜欢的曲线的方程式。
如果你喜欢数学艺术，我建议你看看哈米德·纳德里·叶加内的作品，他根据数学概念构建了美丽的图像。
蝴蝶曲线
Fay（1989）在论文中定义了蝶形曲线。该曲线根据以下参数方程进行描述：

x=sin(t)(ecos(t)−2cos(4t)−sin5(t12))

y=cos(t)(ecos(t)−2cos(4t)−sin5(t12))

t is in the range: [0,12π]

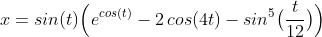
注意，t的范围。

绘制曲线网站：www.desmos.com/calculator/igjkczpymw?lang=zh-CN

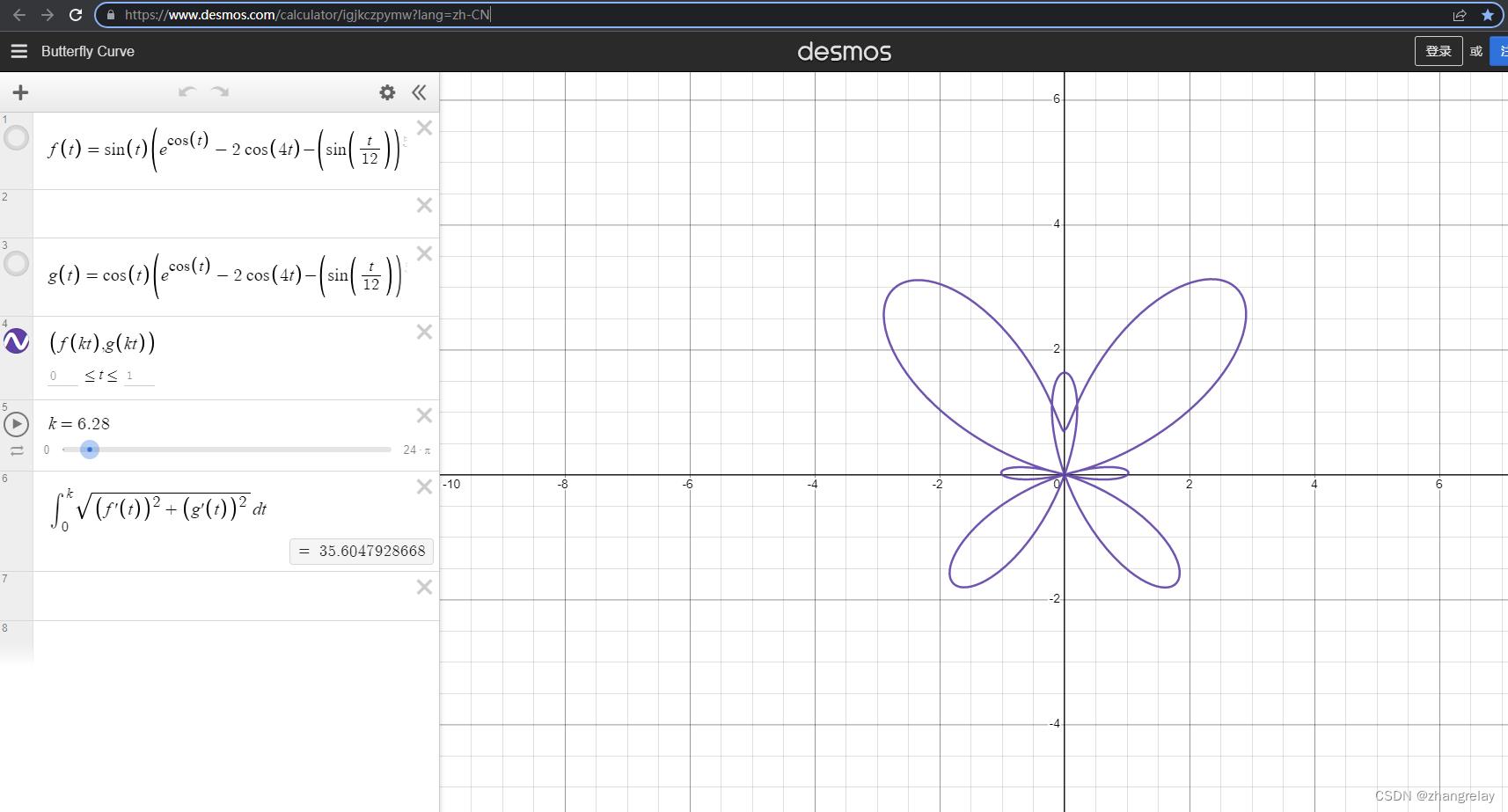
# the function butterfly generates a data frame
# by evaluating the parametric equations that
# define the rose curve in the range of 0 to 12pi
butterfly <- function(n=1000)
  data.frame(t = seq(0,12*pi,length.out = n)) %>%
    mutate(x = sin(t)*(exp(cos(t))-2*cos(4*t)-(sin(t/12))^5),
           y = cos(t)*(exp(cos(t))-2*cos(4*t)-(sin(t/12))^5))

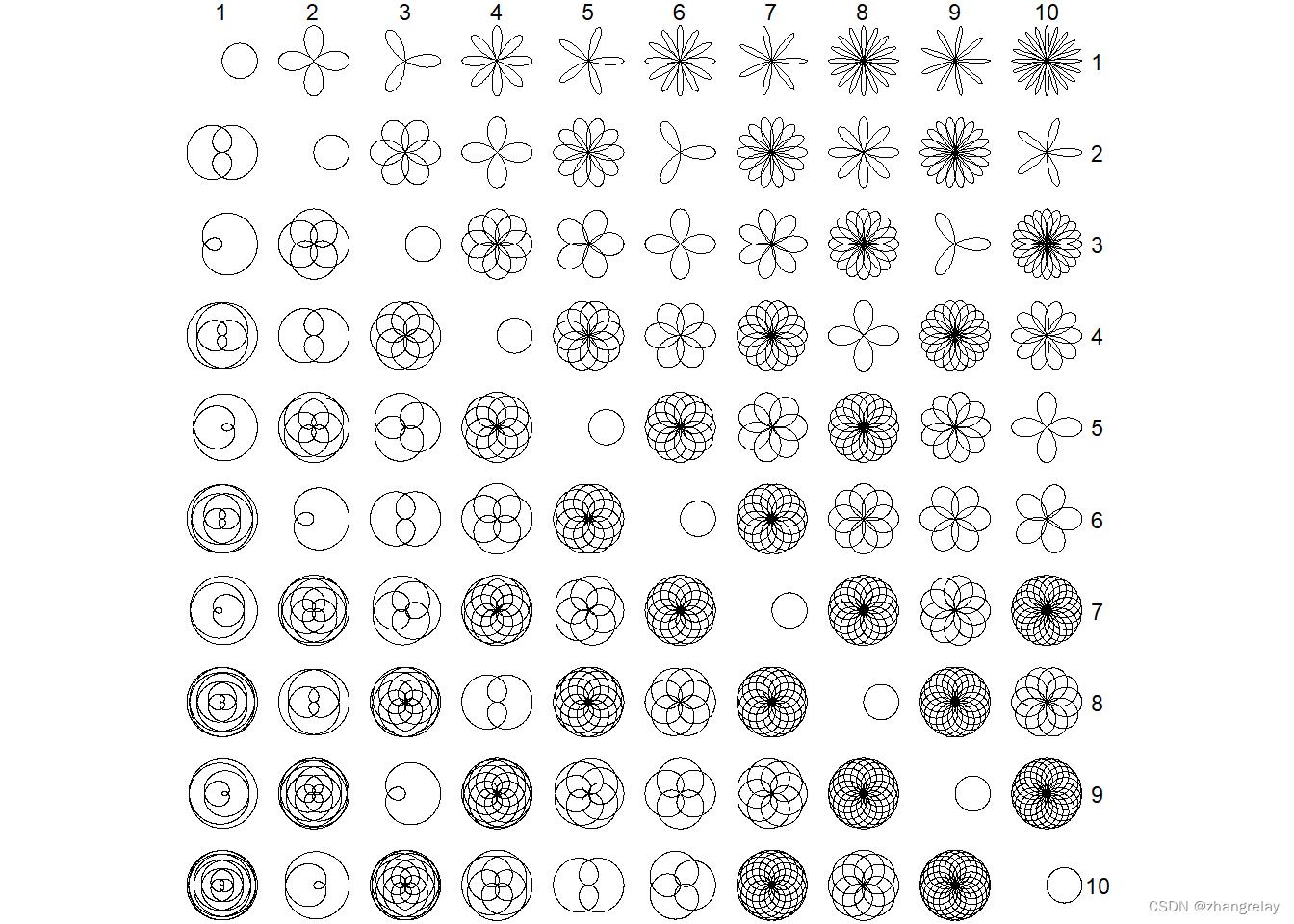
玫瑰
该曲线由以下参数方程描述：

 

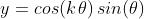
n和d值组合的玫瑰曲线，定义k=n/d。
n和d跨度在[1，10]范围内

rose_curve <- function(n,d) 
  data.frame(theta = seq(0, 32*pi,pi/180)) %>%
    mutate(x = cos(n/d*theta)*cos(theta),
           y = cos(n/d*theta)*sin(theta)) %>%
    select(x, y)

 

---

次摆线
有关内摆线的详细信息，请参阅Wolfram MathWorld文章。
当x（0）=a时，定义内摆线的参数方程为：

 

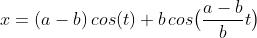
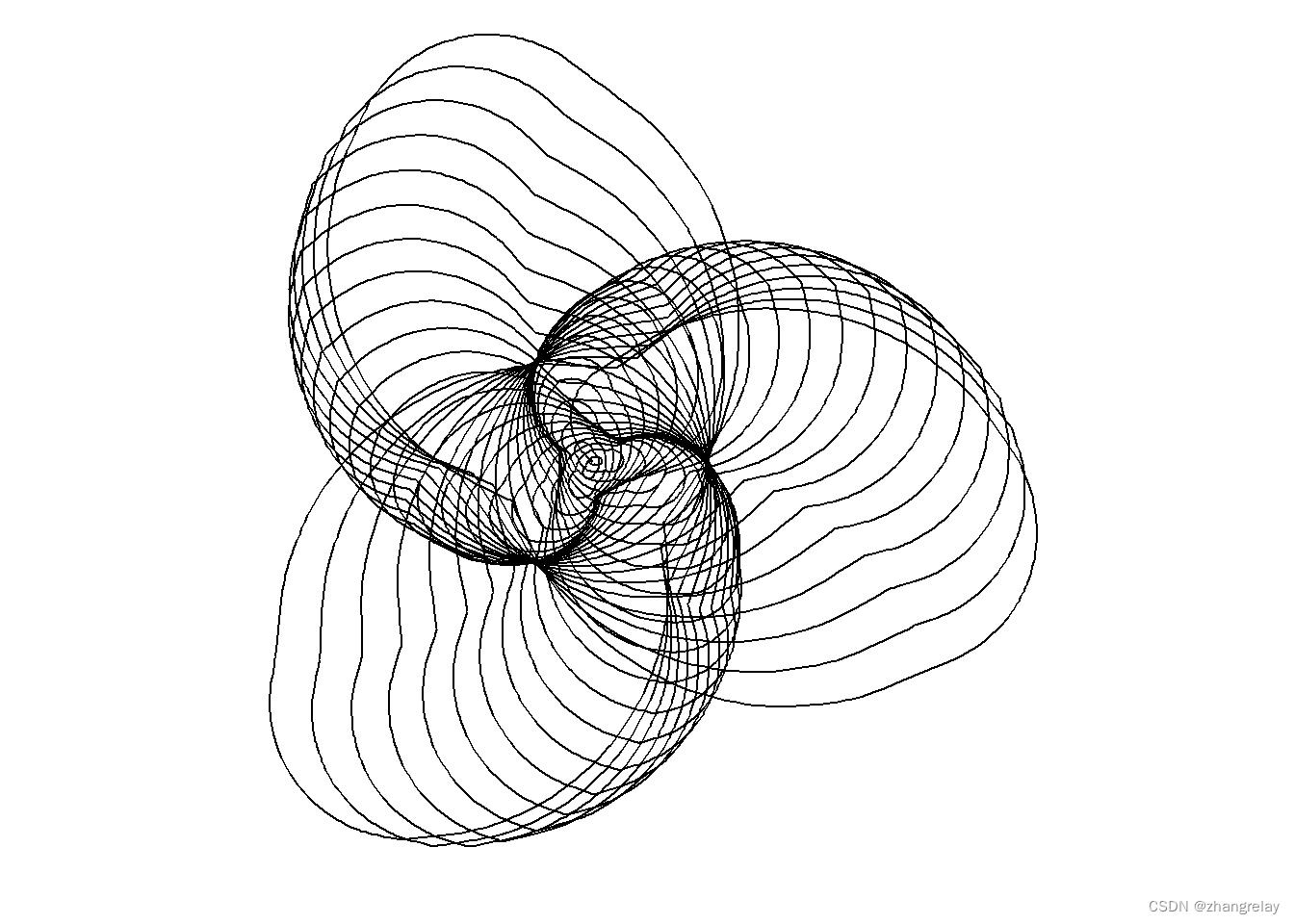
hypocycloid <- function(n = 200, a_inc,b_inc,t_inc)
  a0 = seq(1,n)
  b0 = seq(1,n)
  t0 = seq(0,n-1)
  for (i in 2:n) 
    a0[i]=a0[i-1]+a_inc
    b0[i]=b0[i-1]+b_inc
    t0[i]=t0[i-1]+t_inc
  
  data.frame(a0,b0,t0) %>%
    mutate(  x0 = (a0 - b0)*cos(t0) + b0 * cos((a0/b0-1)*t0),
             y0 = (a0 - b0)*sin(t0) - b0 * sin((a0/b0-1)*t0),
             x1 = c(x0[2:n],x0[1]),
             y1 = c(y0[2:n],y0[1])) %>%
    select(x0,y0,x1,y1,t0)

---

Clifford吸引子
Clifford吸引子由以下方程定义：
xn+1=sin（ayn）+ccos（axn）
yn+1=sin（bxn）+dcos（byn）

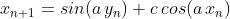
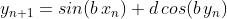
上述方程确定了从点（x0，y0）开始并根据参数a、b、c和d的粒子离散步的（X，Y）位置。
功能选择基于Antonio Sánchez Chinchón的实现。唯一的区别是拾音器是基于R的，而Sánchez Chinchón通过rcpp包在C++中定义了他的功能。

 

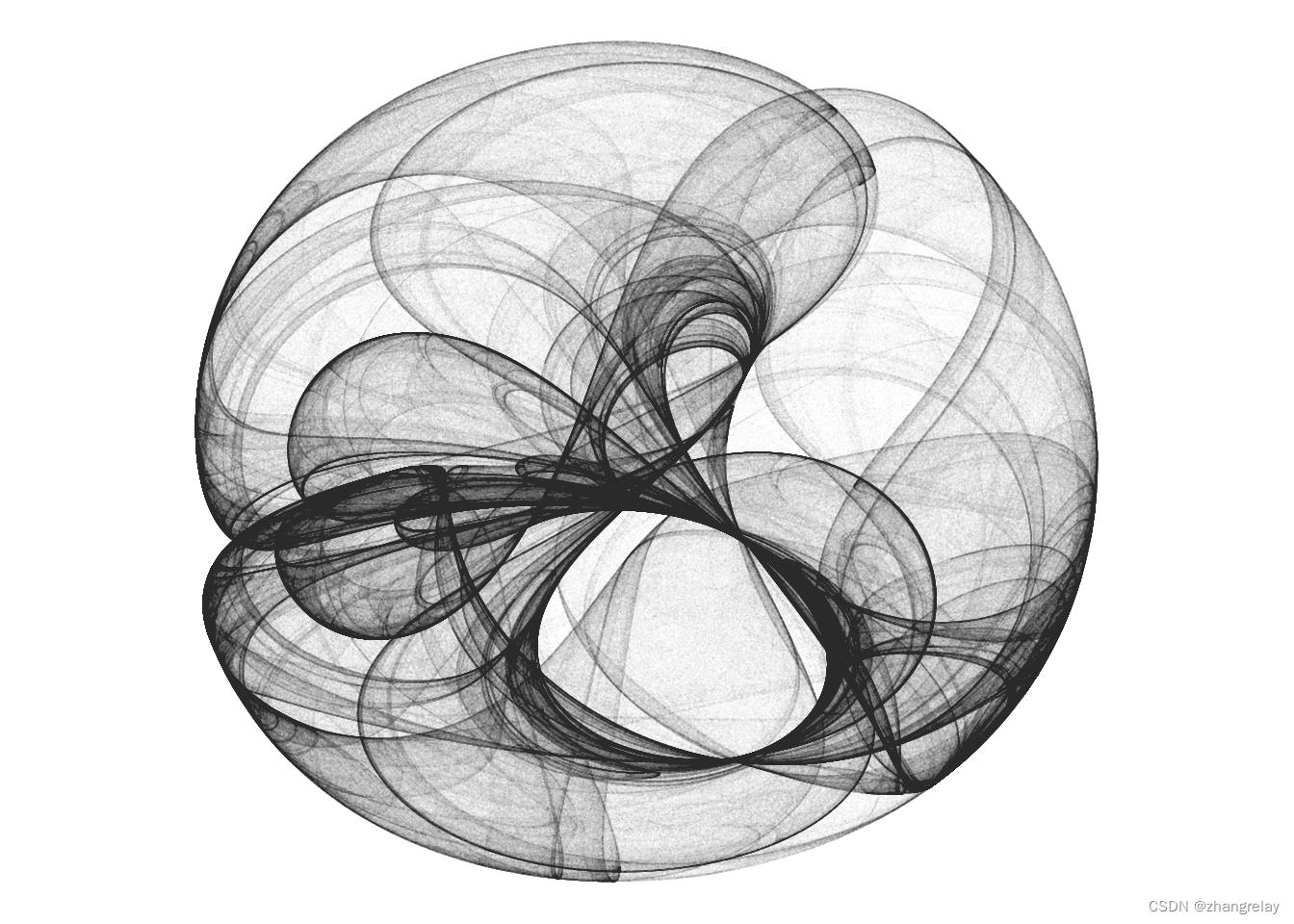
# n is set to 1M
# a, b, c and d are the parameters of the equations
pickover <- function(n=10000000,a,b,c,d)
  x <- vector("numeric", n)
  y <- vector("numeric", n)
  x[1] <- 0
  y[1] <- 0
  for(i in 2:n) 
    x[i] <- sin(a * y[i-1]) + c * cos(a * x[i-1])
    y[i] <- sin(b * x[i-1]) + d * cos(b * y[i-1])
  
  df <- data.frame(x = x, y = y)

 

---

References

Fay, Temple H. 1989. “The Butterfly Curve.” Journal Article. The American Mathematical Monthly 96 (5): 442–43.