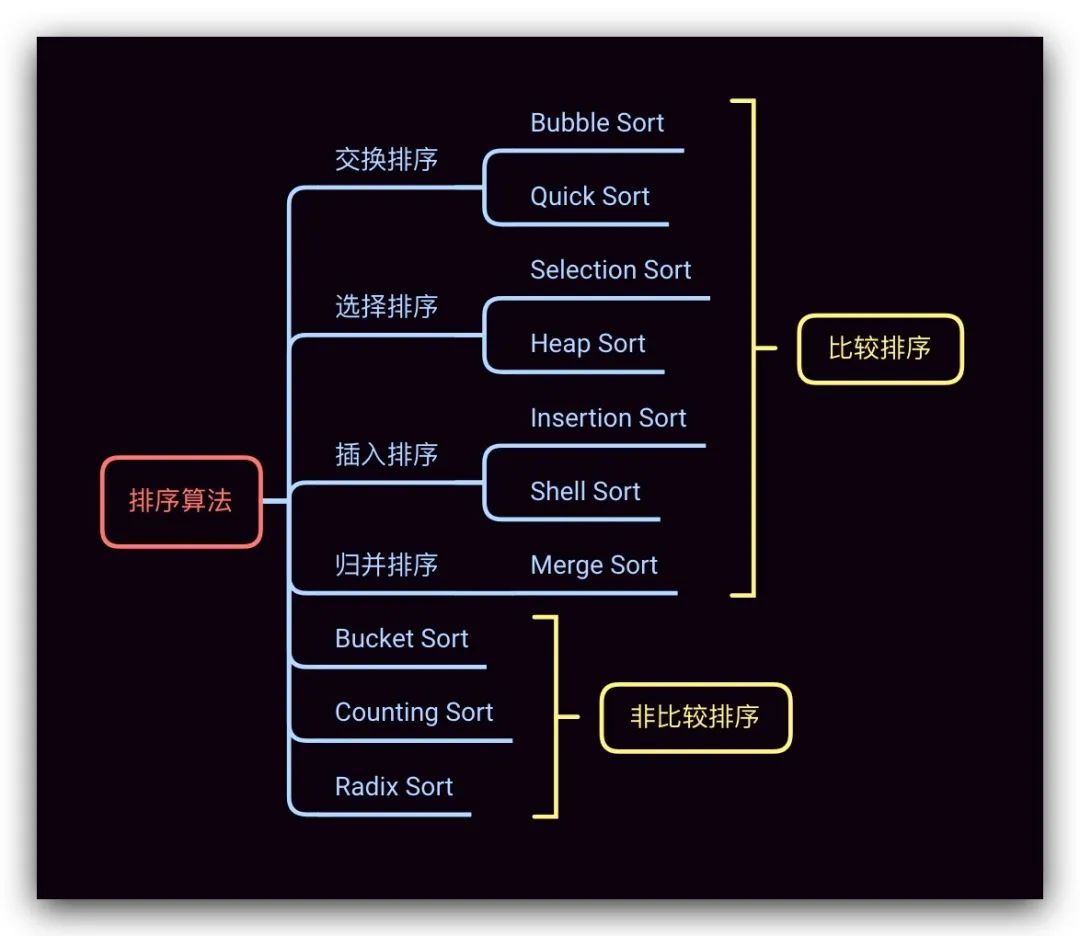
排序算法:Counting Sort 计数排序
Posted ProjectDaedalus
tags:
篇首语:本文由小常识网(cha138.com)小编为大家整理,主要介绍了排序算法:Counting Sort 计数排序相关的知识,希望对你有一定的参考价值。
基本思想
Counting Sort 计数排序,顾名思义,就是统计待排序数组元素的出现次数。其基本思想比较简单:
根据待排序元素的数值范围大小k(max-min+1),建立一个k大小的频数统计数组counts。对于counts数组而言,其索引范围 0 ~ k-1,正好可以对应到待排序元素的取值范围min~max上
统计待排序元素element的次数,并其存储到counts数组中,即counts[ elemet-min ] = countValue
待计数统计完成后遍历counts数组,根据次数值来输出原待排序元素值,此时即完成排序
这里给出计数排序在Java下的实现:
/**
* 计数排序
*/
public class CountingSort {
/**
* 升序排列 (非稳定)
*/
public static void sort1() {
// 获取测试用例
int[] array = getTestCase();
int size = array.length;
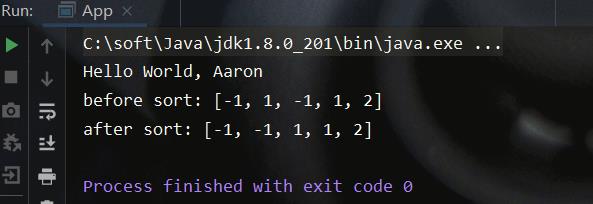
System.out.println("before sort: " + Arrays.toString(array) );
// 求取最值
int[] minMax = getMinMax(array);
int min = minMax[0];
int max = minMax[1];
// 根据数据范围创建统计数组
int k = max-min+1;
int[] counts = new int[ k ];
// 统计原数组中元素出现的次数
for(int i=0; i<size; i++) {
int dataInCountIndex = array[i] - min; // 计算原数组元素在统计数组中的索引
counts[dataInCountIndex] +=1; // 统计该值次数
}
int j = 0; // 有序的数据的插入位置
for(int i=0; i<k; i++) {
int originalData = i + min; // 还原 原排序数组的数据值
while( counts[i]>0 ) {
array[j] = originalData;
counts[i]--; // 该数值出现的次数减1
j++; // 更新有序数据的插入位置
}
}
System.out.println("after sort: " + Arrays.toString(array) );
}
/**
* 求指定数组的最值
* @param array
* @return [0]: 最小值; [1]: 最大值;
*/
private static int[] getMinMax(int[] array) {
int min = array[0];
int max = array[0];
for(int i=1; i<array.length; i++) {
if( array[i]>max ) {
max = array[i];
}
if( array[i]<min ) {
min = array[i];
}
}
int[] minMax = new int[]{min,max};
return minMax;
}
/**
* 获取测试用例
*/
private static int[] getTestCase() {
int[] caseArray = {-1,1,-1,1,2};
return caseArray;
}
}
测试结果如下:
稳定的计数排序
基本原理
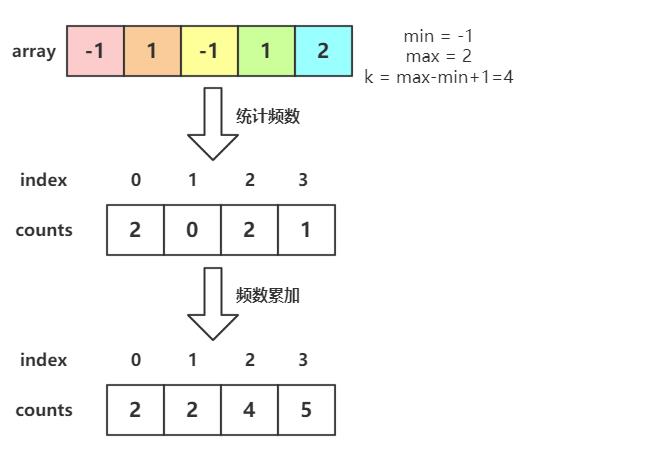
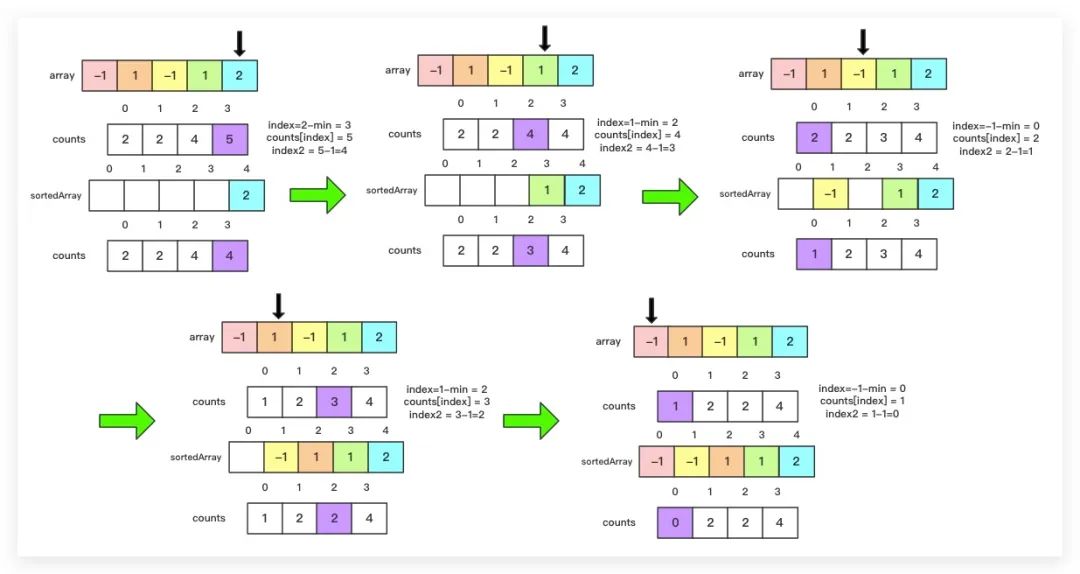
上面的计数排序算法是非稳定的,而一般我们所说的计数排序都是稳定的。那么如何保证计数排序的稳定性呢?其实很简单,只需在统计完待排序元素的频数后,对counts作累加计算(counts[i] = counts[i-1] + counts[i]),即计算统计数组中指定索引位置上的元素在排序后的位置;然后倒序遍历原数组,根据counts数组中的排序位置来将待排序元素放入合适的位置,同时将counts数组相应的值减1,以使下一个重复的排序元素可以放在前一位的位置上,以保证稳定性
算法图解
下图即是通过稳定的计数排序对 -1,1,-1,1,2 序列进行升序排列的图解过程
1. 建立counts数组
2. 倒序遍历原待排序数组,按升序排列
Java实现
这里给出计数排序在Java下的实现:
/**
* 计数排序
*/
public class CountingSort {
...
/**
* 升序排列 (稳定)
*/
public static void sort2() {
// 获取测试用例
int[] array = getTestCase();
int size = array.length;
System.out.println("before sort: " + Arrays.toString(array) );
// 求取最值
int[] minMax = getMinMax(array);
int min = minMax[0];
int max = minMax[1];
// 根据数据范围创建统计数组
int k = max-min+1;
int[] counts = new int[ k ];
// 统计原数组中元素出现的次数
for(int i=0; i<size; i++) {
int dataInCountIndex = array[i] - min; // 计算原数组元素在统计数组中的索引
counts[dataInCountIndex] +=1; // 统计该值次数
}
// 计算统计数组的累计值,即计算 统计数组中指定索引位置上的元素在排序后的位置
// 如果该索引位置上有重复元素,则为重复元素所占的最大排序位置
for(int i=1; i<k; i++) {
counts[i] = counts[i-1] + counts[i];
}
int[] sortedArray = new int[size]; // 排序结果数组
// 倒序遍历原数组,保证稳定性
for(int i=size-1; i>=0; i--) {
int dataInCountIndex = array[i] - min; // 计算原数组元素在统计数组中的索引
// 计算其排序后的位置, 因为数组索引从0开始计算,故应对排序位置减1
// 例如,排在最前面的元素,排序位置为1,则其在数组中的位置索引应为0
int sortIndex = counts[dataInCountIndex] - 1;
sortedArray[sortIndex] = array[i]; // 将原数组元素放入排序后的位置上
counts[dataInCountIndex]--; // 下一个重复的元素,应排前一个位置,以保证稳定性
}
System.out.println("after sort: " + Arrays.toString(sortedArray) );
}
...
}
测试结果如下:
特点
复杂度、稳定性
这里以稳定的计数排序进行说明:
缺陷
计数排序只能适用待排序元素为整数的场景
待排序元素的数值范围(极差)过大的情况下,计数排序会浪费大量空间,故一般不推荐使用计数排序
参考文献
算法导论 · 第3版
以上是关于排序算法:Counting Sort 计数排序的主要内容,如果未能解决你的问题,请参考以下文章