数据结构&算法-图最短路径

Posted 2021-08-02 彩色墨水

图最短路径介绍

对于网图来说，最短路径，是指两顶点之间经过的边上权值之和最少路径，并且我们称路径上的第一个顶点是源点，最后一个顶点终点。

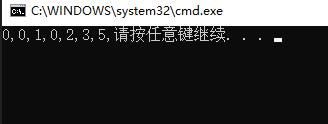
迪杰斯特拉算法运行结果

        static void Main(string[] args)
        {

            //  int[,] aaa = new int[4, 3];

            LedByMatrix ledByMatrix = new LedByMatrix(7, new int[11, 3] { { 0, 1, 3 },{ 0, 2, 7 },{ 0, 3, 5 },
                                                         { 1, 2, 2 },{ 2, 3, 3 },{ 1, 4, 6 },
                                                         { 2, 4, 3 },{ 3, 6, 8 },{ 3, 5, 2 },
                                                         { 5, 6, 2 },{ 4, 6, 2},});
            Dijkstra dijkstra = new Dijkstra();
            dijkstra.GetDijkstraPath(ledByMatrix.G);
            //Floyd floyd = new Floyd();
            //floyd.GetFloydPath(ledByMatrix.G);
        }

   /// <summary>
    /// 邻接矩阵
    /// </summary>
    class LedByMatrix
    {
        int[,] _g;
        int INFINITY = 65535;
        public int[,] G { get => _g; }
        /// <summary>
        /// 初始化邻接矩阵
        /// </summary>
        /// <param name="vertexCount">顶点数</param>
        /// <param name="arr">A-B及其权值，{1,3,7}表示V1到V3的权值是7</param>
        public LedByMatrix(int vertexCount, int[,] arr)
        {
            _g = new int[vertexCount, vertexCount];
            for (int i = 0; i < _g.GetLength(0); i++)
            {
                for (int J = 0; J < _g.GetLength(1); J++)
                {
                    _g[i, J] = INFINITY;
                }
            }
            for (int i = 0; i < arr.GetLength(0); i++)
            {
                _g[arr[i, 0], arr[i, 1]] = arr[i, 2];
                _g[arr[i, 1], arr[i, 0]] = arr[i, 2];
            }
        }

        /// <summary>
        /// 打印邻接矩阵
        /// </summary>
        public void PrintLedByMatrix()
        {

            for (int i = 0; i < _g.GetLength(0); i++)
            {
                for (int j = 0; j < _g.GetLength(1); j++)
                {
                    Console.Write(_g[i, j] + ",");

                }
                Console.WriteLine();
            }
        }
    }

   /// <summary>
    /// 迪杰斯特拉算法
    /// </summary>
    class Dijkstra
    {

        //初始化数据，到每个顶点的最短路径权值初始化
        //求V0到某个顶点的最短路径
        bool[] final;//V0到该点的最小路径已经求的
        int[] pathMatirx;//到该点的最短路径前驱点
        int[] shortPathTable;//到该点的最短路径权值
        int INFINITY = 65535;

        /// <summary>
        /// 求最短路径
        /// </summary>
        /// <param name="G"></param>
        public void GetDijkstraPath(int[,] G)
        {
            final = new bool[G.GetLength(0)];
            pathMatirx = new int[G.GetLength(0)];
            shortPathTable = new int[G.GetLength(0)];
            for (int j = 0; j < G.GetLength(0); j++)
            {
                shortPathTable[j] = INFINITY;
                final[j] = false;
                pathMatirx[j] = 0;
                shortPathTable[j] = G[0, j];
            }
            final[0] = true;
            shortPathTable[0] = 0;
            for (int i = 1; i < G.GetLength(0); i++)
            {

                int min = INFINITY;
                int k = 0;
                for (int w = 0; w < G.GetLength(0); w++)
                {
                    if (!final[w] && shortPathTable[w] < min)//还未确认的顶点中离V0最短的那个
                    {
                        min = shortPathTable[w];
                        k = w;
                    }
                }
                final[k] = true;

                for (int w = 0; w < G.GetLength(0); w++)
                {
                    if (!final[w] && min + G[k, w] < shortPathTable[w])//找凡是还没确认的顶点中，如果经过K顶点的路径比现在的路径短的话，更新
                    {
                        shortPathTable[w] = min + G[k, w];
                        pathMatirx[w] = k;//确认前驱点
                    }
                }
            }

            PrintShortestPath();
        }

        /// <summary>
        /// 打印最短路径
        /// </summary>
        public void PrintShortestPath()
        {
            for (int i = 0; i < shortPathTable.Length; i++)
            {
                Console.Write(pathMatirx[i] + ",");
                //Console.Write(shortPathTable[i] + ",");
            }
        }
    }

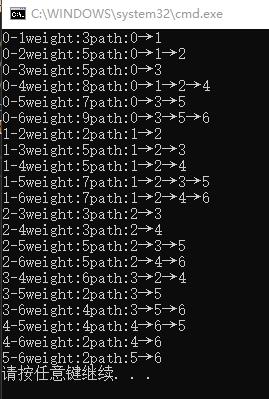
弗洛伊德运行结果

 /// <summary>
    /// 弗洛伊德算法
    /// </summary>
    class Floyd
    {
        int[,] pathmatirx;
        int[,] shortPathTable;
        int numV;
        public void GetFloydPath(int[,] G)
        {
            numV = G.GetLength(0);
            pathmatirx = new int[G.GetLength(0), G.GetLength(1)];
            shortPathTable = new int[G.GetLength(0), G.GetLength(1)];
            for (int i = 0; i < pathmatirx.GetLength(0); i++)
            {
                for (int j = 0; j < pathmatirx.GetLength(1); j++)
                {
                    pathmatirx[i, j] = j;
                    shortPathTable[i, j] = G[i, j];
                }
            }

            for (int k = 0; k < numV; k++)
            {
                for (int v = 0; v < numV; v++)
                {
                    for (int w = 0; w < numV; w++)
                    {
                        if (shortPathTable[v, w] > shortPathTable[v, k] + shortPathTable[k, w])
                        {
                            shortPathTable[v, w] = shortPathTable[v, k] + shortPathTable[k, w];
                            pathmatirx[v, w] = pathmatirx[v, k];
                        }
                    }
                }
            }
            PrintPath();
        }

        void PrintPath()
        {
            for (int v = 0; v < numV; v++)
            {
                for (int w = v + 1; w < numV; w++)
                {
                    Console.Write("{0}-{1}weight:{2}", v, w, shortPathTable[v, w]);
                    int k = pathmatirx[v, w];
                    Console.Write("path:{0}", v);
                    while (k != w)
                    {
                        Console.Write("→{0}", k);
                        k = pathmatirx[k, w];

                    }
                    Console.Write("→{0}", w);
                    Console.WriteLine();
                }
                
            }
        }
    }