Multiple Qubits and Entangled States
Posted 安徽思远
tags:
篇首语：本文由小常识网(cha138.com)小编为大家整理，主要介绍了Multiple Qubits and Entangled States相关的知识，希望对你有一定的参考价值。
Single Qubit Superposition
量子态可以通过一些unitary transformation转变为叠加态量子态。
如Hardmard Gate就可以将
∣
0
⟩
|0\\rangle
∣0⟩转变为叠加态
q
0
q_{0}
q0。
H
∣
0
⟩
=
(
1
2
1
2
1
2
−
1
2
)
(
1
0
)
=
(
1
2
1
2
)
H|0\\rangle=\\left(\\begin{array}{cc}\\frac{1}{\\sqrt{2}} & \\frac{1}{\\sqrt{2}} \\\\ \\frac{1}{\\sqrt{2}} & \\frac{-1}{\\sqrt{2}}\\end{array}\\right)\\left(\\begin{array}{l}1 \\\\ 0\\end{array}\\right)=\\left(\\begin{array}{l}\\frac{1}{\\sqrt{2}} \\\\ \\frac{1}{\\sqrt{2}}\\end{array}\\right)
H∣0⟩=(2121212−1)(10)=(2121)
∣ q 0 ⟩ = 1 2 ∣ 0 ⟩ + 1 2 ∣ 1 ⟩ \\left|q_{0}\\right\\rangle=\\frac{1}{\\sqrt{2}}|0\\rangle+\\frac{1}{\\sqrt{2}}|1\\rangle ∣q0⟩=21∣0⟩+21∣1⟩
有趣的是，量子计算机中的任何操作均是可逆的(reversible)，这也就意味着，叠加态 ∣ q 0 ⟩ |q_{0}\\rangle ∣q0⟩可以通过变化再重新变回 ∣ 0 ⟩ |0\\rangle ∣0⟩。
这里我们只需要对 q 0 q_{0} q0再进行一次Hardmard变换即可。
H ∣ q 0 ⟩ = ( 1 2 1 2 1 2 − 1 2 ) ( 1 2 1 2 ) = ( 1 0 ) H|q_{0}\\rangle=\\left(\\begin{array}{cc}\\frac{1}{\\sqrt{2}} & \\frac{1}{\\sqrt{2}} \\\\ \\frac{1}{\\sqrt{2}} & \\frac{-1}{\\sqrt{2}}\\end{array}\\right)\\left(\\begin{array}{l}\\frac{1}{\\sqrt{2}} \\\\ \\frac{1}{\\sqrt{2}} \\end{array}\\right)=\\left(\\begin{array}{l}1 \\\\ 0\\end{array}\\right) H∣q0⟩=(2121212−1)(2121)=(10)
对于叠加态，如 ∣ q 0 ⟩ |q_{0}\\rangle ∣q0⟩，我们在测量时会有50%的概率得到0，50%的概率得到1。这里的概率是由振幅决定的，振幅的平方即是概率。对于 ∣ q 0 ⟩ |q_{0}\\rangle ∣q0⟩， ∣ 0 ⟩ |0\\rangle ∣0⟩的振幅为 1 2 \\frac{1}{\\sqrt{2}} 21。
这里的测量，是在01坐标系下来看结果的，如果我们变更坐标系，得到的结果又将不同。
我们通过qiskit来进行测量一下。
from qiskit import QuantumCircuit, Aer, assemble
from math import pi
import numpy as np
from qiskit.visualization import plot_histogram, plot_bloch_multivector
qc = QuantumCircuit(1,1)
qc.h([0])
qc.measure(0,0)
qc.draw("mpl")
# use local simulator
qasm_sim = Aer.get_backend('qasm_simulator')
qobj = assemble(qc)
results = qasm_sim.run(qobj,shots = 1).result()
answer = results.get_counts()
plot_histogram(answer)
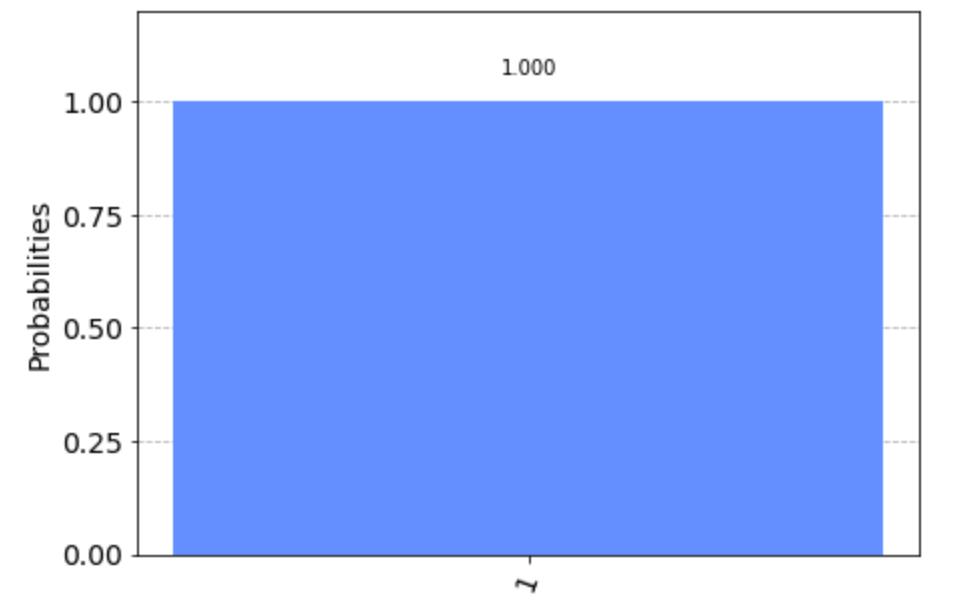
这里设置为测量一次，结果自然是一种。图中为1。
若测量1024次，结果就接近50%的均分。
Multiple Qubits Superposition
以上讨论了单个qubit的系统，对于多个qubit呢？
qc = QuantumCircuit(2,2)
# Apply H-gate and measure to each qubit:
for qubit in range(2):
qc.h(qubit)
qc.measure(qubit,qubit)
qc.draw('mpl')
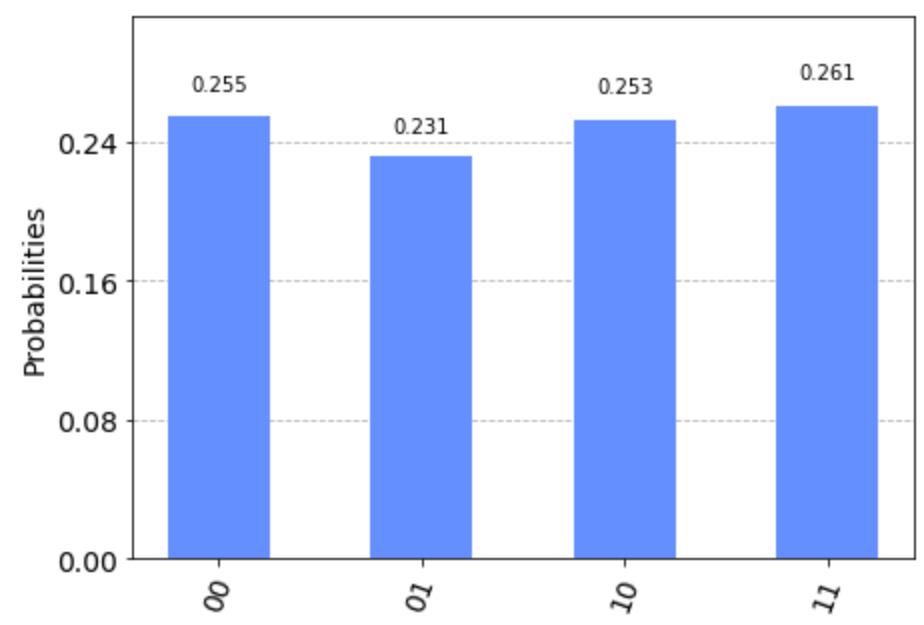
测量1024次，我们得到的结果仍然接近均分。
这里是为什么呢？我们用数学来推导。
首先对于multiple Qubits的表示方法，我们需要借助tensor product。 以上是关于Multiple Qubits and Entangled States的主要内容，如果未能解决你的问题，请参考以下文章 Multiple Qubits and Entangled States The Precision Atom Qubits Can “Talk” to Each Other $element 匹配或 $unwind 与 AND/Multiple 条件与 mongoDB 聚合
基本规则如下，如初始状态
∣
00
⟩
=
∣
0
⟩
⊗
∣
0
⟩