Codeforces990 G. GCD Counting（点分治）

Posted 2021-07-23 live4m

题意：

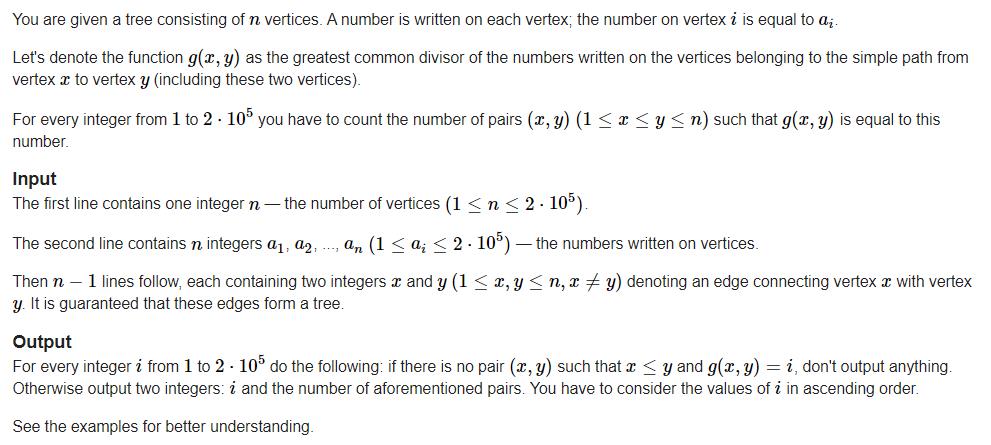
解法：

点分治.

对于点x,用map维护子树点到x的数的个数,
每次暴力计算经过x的路径即可,
由于gcd是收敛的,数不会太多.

code：

#include<bits/stdc++.h>
#define ll long long
using namespace std;
const int maxm=2e5+5;
int head[maxm],nt[maxm<<1],to[maxm<<1],tot;
int sz[maxm],son[maxm],root,size;
int mark[maxm];
ll cnt[maxm];
int a[maxm];
int n;
void add(int x,int y){
    tot++;nt[tot]=head[x];head[x]=tot;to[tot]=y;
}
void getroot(int x,int fa){
    sz[x]=1;son[x]=0;
    for(int i=head[x];i;i=nt[i]){
        int v=to[i];
        if(v==fa||mark[v])continue;
        getroot(v,x);
        sz[x]+=sz[v];
        son[x]=max(son[x],sz[v]);
    }
    son[x]=max(son[x],size-sz[x]);
    if(son[x]<son[root])root=x;
}
map<int,int>last;
map<int,int>now;
void dfs(int x,int fa,int val){
    now[val]++;
    for(int i=head[x];i;i=nt[i]){
        int v=to[i];
        if(v==fa||mark[v])continue;
        dfs(v,x,__gcd(val,a[v]));
    }
}
void solve(int x){
    cnt[a[x]]++;
    last.clear();
    last[a[x]]++;
    for(int i=head[x];i;i=nt[i]){
        int v=to[i];
        if(mark[v])continue;
        now.clear();
        dfs(v,x,__gcd(a[x],a[v]));
        for(auto i:now){
            for(auto j:last){
                cnt[__gcd(i.first,j.first)]+=1ll*i.second*j.second;
            }
        }
        for(auto i:now){
            last[i.first]+=i.second;
        }
    }
}
void divide(int x){
    mark[x]=1;
    solve(x);
    for(int i=head[x];i;i=nt[i]){
        int v=to[i];
        if(mark[v])continue;
        son[root=0]=size=sz[v];
        getroot(v,v);
        divide(root);
    }
}
void solve(){
    scanf("%d",&n);
    for(int i=1;i<=n;i++)scanf("%d",&a[i]);
    for(int i=1;i<n;i++){
        int a,b;scanf("%d%d",&a,&b);
        add(a,b);add(b,a);
    }
    son[root=0]=size=n;
    getroot(1,1);
    divide(root);
    for(int i=1;i<=2e5;i++){
        if(cnt[i]){
            printf("%d %lld\\n",i,cnt[i]);
        }
    }
}
signed main(){
    solve();
    return 0;
}

---