NC52275 图的遍历

Posted 2021-06-15 吃花椒的妙酱

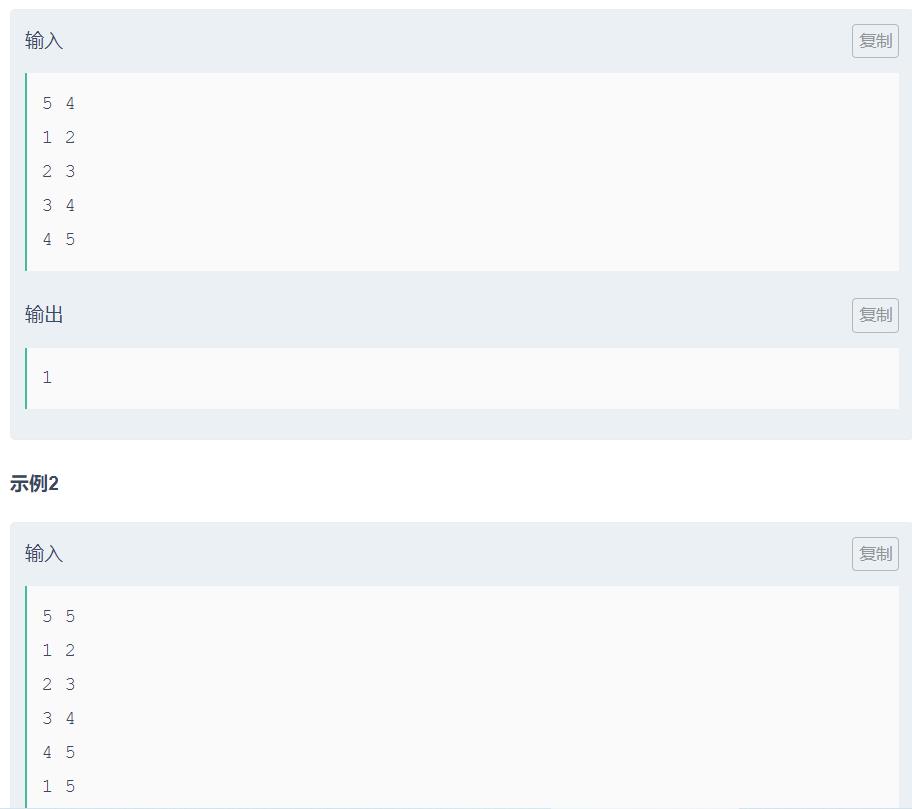
可以发现当，当出现奇环并且整张图联通时，可以跳两格遍历所有的点。
问题转换为判奇环，和连通块数目。
如何判奇环，在一个连通块中，如果相邻结点有(deep[i] - deep[j])%2==0，两个结点的深度差值为偶数，则有奇环，因为相邻距离为1，加上他们到所在的连通块起点距离为偶数，则是奇环。

#include <iostream>
#include <cstring>
#include <cmath>
#include <cstdio>
#include <cstdlib>
#include <algorithm>
#include <map>
#include <vector>
#include <queue>
#include <bitset>
using namespace std;
#define _for(i,a,b) for(int i=(a) ;i<=(b) ;i++)
#define _rep(i,a,b) for(int i=(a) ;i>=(b) ;i--)
#define mst(v,s) memset(v,s,sizeof(v))
#define pb push_back
#define IOS ios::sync_with_stdio(false)
#define int long long
#define inf 0x3f3f3f3f
#define lson p<<1,l,mid
#define rson p<<1|1,mid+1,r
#define ls p<<1
#define rs p<<1|1
typedef long long ll;
const int N=1e5+10;
int n,m;
bool boo;//是否有奇环
struct ty
{
    int t,next;
}edge[N<<1];
int tot,head[N];
void addedge(int x ,int y)
{
    edge[++tot].t=y;
    edge[tot].next=head[x];
    head[x]=tot;
}
int v[N];
void dfs(int x)
{
    for(int i=head[x] ;i!=0 ;i=edge[i].next)
    {
        int y = edge[i].t;
        if( v[y] )
        {
            if((v[y]-v[x])%2==0) boo=1;
            //到深度差为偶数则存在奇环
        }
        else
        {
            v[y]= v[x] + 1;
            dfs(y);
        }
    }
    return;
}
void solve()
{
    int cnt=0;
    _for(i,1,n)
    {
        if( !v[i] )
        {
            v[i]=1;
            cnt++;//连通块数
            dfs(i);
        }
    }
    cnt--;
    if( boo ) cout<<cnt<<endl;//有奇环的话
    else cout<<cnt+1<<endl;

}
signed main()
{
    ///!!!
//    freopen("data.txt","r",stdin);
//    !!!
    IOS;
    cin>>n>>m;
    _for(i,1,m)
    {
        int x,y;
        cin>>x>>y;
        addedge(x,y);addedge(y,x);
    }
    solve();
}