993. 二叉树的堂兄弟节点

Posted 2021-05-18 阿飞研学

题目：

在二叉树中，根节点位于深度 0 处，每个深度为 k 的节点的子节点位于深度 k+1 处。

如果二叉树的两个节点深度相同，但 父节点不同 ，则它们是一对堂兄弟节点。

我们给出了具有唯一值的二叉树的根节点 root ，以及树中两个不同节点的值 x 和 y 。

只有与值 x 和 y 对应的节点是堂兄弟节点时，才返回 true 。否则，返回 false。

示例1：

输入：root = [1,2,3,4], x = 4, y = 3输出：false

示例2：

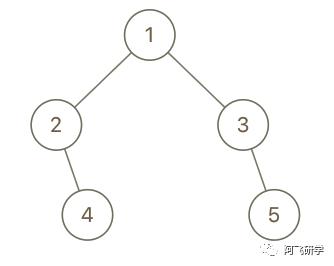
输入：root = [1,2,3,null,4,null,5], x = 5, y = 4输出：true

示例3：

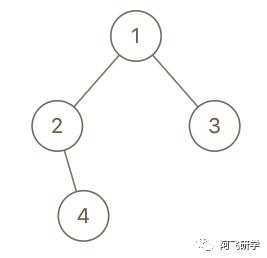
输入：root = [1,2,3,null,4], x = 2, y = 3输出：false

提示：

• 二叉树的节点数介于 2 到 100 之间。

• 每个节点的值都是唯一的、范围为 1 到 100 的整数。

思路：

遍历二叉树，有深度优先搜索和广度优先搜索两种方法，需要注意的是要记录父亲节点和x,y结点的深度Depth。最后比较父亲节点是否不一样，和深度是否一致。

代码（深度优先搜索DFS）：

/** * Definition for a binary tree node. * type TreeNode struct { * Val int * Left *TreeNode * Right *TreeNode * } */func isCousins(root *TreeNode, x int, y int) bool { var xParent, yParent *TreeNode var xDepth, yDepth int var xFound, yFound bool var dfs func(node, parent *TreeNode, depth int) dfs = func(node, parent *TreeNode, depth int){ if node == nil{ return } if node.Val == x{ xParent, xDepth, xFound = parent, depth, true }else if node.Val == y{ yParent, yDepth, yFound = parent, depth, true } if xFound && yFound{ return } dfs(node.Left, node, depth+1) dfs(node.Right, node, depth+1) } dfs(root, nil, 0) return xParent != yParent && xDepth == yDepth}

结果（DFS）：

代码（广度优先搜素）：

func isCousins(root *TreeNode, x, y int) bool { var xParent, yParent *TreeNode var xDepth, yDepth int var xFound, yFound bool
 // 用来判断是否遍历到 x 或 y 的辅助函数 update := func(node, parent *TreeNode, depth int) { if node.Val == x { xParent, xDepth, xFound = parent, depth, true } else if node.Val == y { yParent, yDepth, yFound = parent, depth, true } }
 type pair struct { node *TreeNode depth int } q := []pair{{root, 0}} update(root, nil, 0) for len(q) > 0 && (!xFound || !yFound) { node, depth := q[0].node, q[0].depth q = q[1:] if node.Left != nil { q = append(q, pair{node.Left, depth + 1}) update(node.Left, node, depth+1) } if node.Right != nil { q = append(q, pair{node.Right, depth + 1}) update(node.Right, node, depth+1) } }
 return xDepth == yDepth && xParent != yParent}

结果：

分析：

两个方法的时间复杂度和空间复杂度都是O(n)