(等价无穷小)泰勒公式——必须背住!!
Posted 大闸謝Gemini
tags:
篇首语:本文由小常识网(cha138.com)小编为大家整理,主要介绍了(等价无穷小)泰勒公式——必须背住!!相关的知识,希望对你有一定的参考价值。
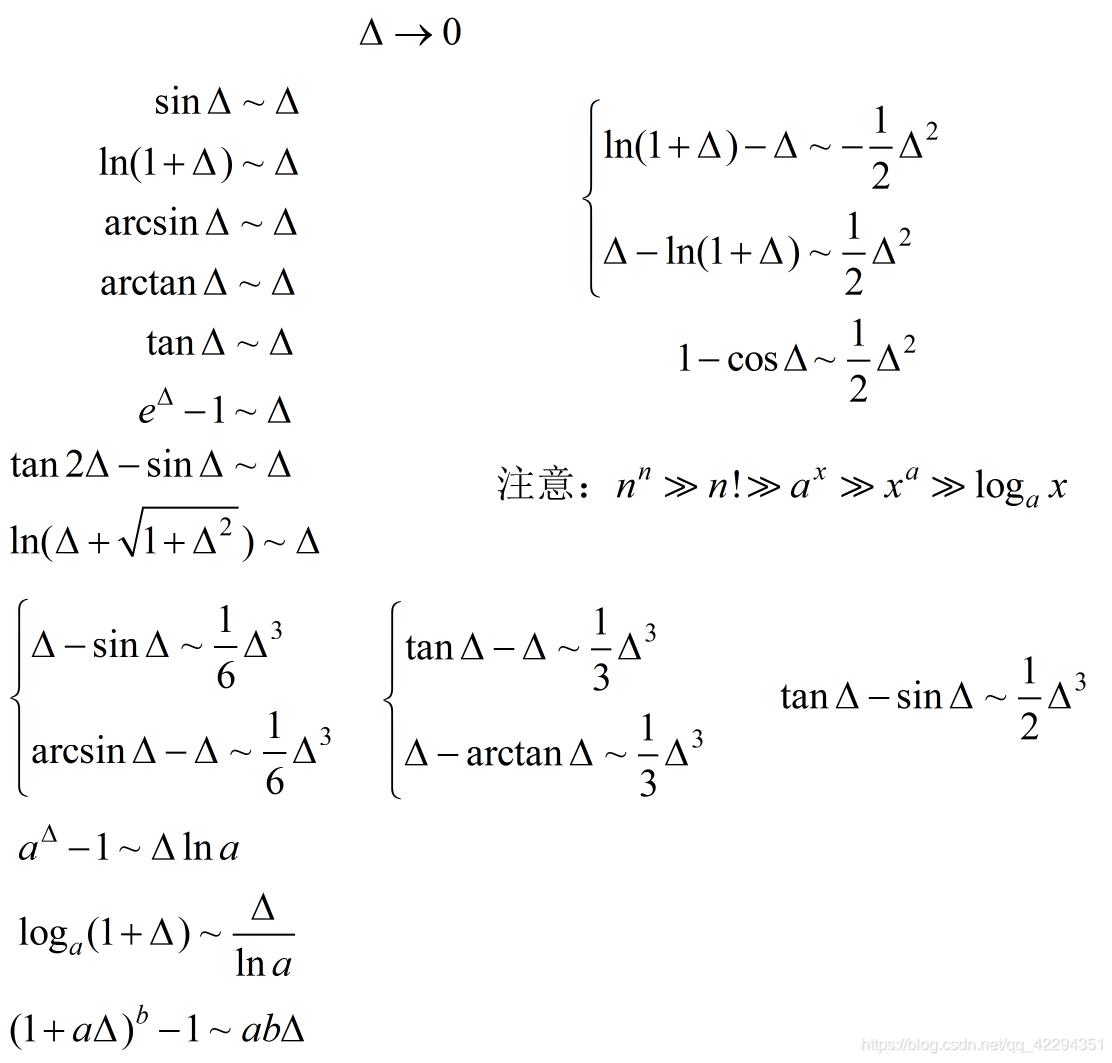
泰勒公式的麦克劳林展开式与等价无穷小
f ( x ) = f ( 0 ) + f ′ ( 0 ) x + f ′ ′ ( 0 ) 2 x 2 + f ′ ′ ′ ( 0 ) 3 ! x 3 + . . . + f ( n ) ( 0 ) n ! x n + o ( x n ) f(x)=f(0)+f'(0)x+\\fracf''(0)2x^2+\\fracf'''(0)3!x^3+...+\\fracf^(n)(0)n!x^n+o(x^n) f(x)=f(0)+f′(0)x+2f′′(0)x2+3!f′′′(0)x3+...+n!f(n)(0)xn+o(xn)
x → 0 x→0 x→0
s
i
n
x
=
x
−
1
6
x
3
+
o
(
x
3
)
sinx=x-\\frac16x^3+o(x^3)
sinx=x−61x3+o(x3)
s
i
n
x
sinx
sinx ~
x
x
x
s
i
n
x
−
x
sinx-x
sinx−x ~
−
1
6
x
3
-\\frac16x^3
−61x3
x
−
s
i
n
x
x-sinx
x−sinx ~
1
6
x
3
\\frac16x^3
61x3
a
r
c
s
i
n
x
=
x
+
1
6
x
3
+
o
(
x
3
)
arcsinx=x+\\frac16x^3+o(x^3)
arcsinx=x+61x3+o(x3)
a
r
c
s
i
n
x
arcsinx
arcsinx ~
x
x
x
t
a
n
x
=
x
+
1
3
x
3
+
o
(
x
3
)
tanx=x+\\frac13x^3+o(x^3)
tanx=x+31x3+o(x3)
t
a
n
x
−
x
tanx-x
tanx−x ~
1
3
x
3
\\frac13x^3
31x3
x
−
t
a
n
x
x-tanx
x−tanx ~
−
1
3
x
3
-\\frac13x^3
−31x3
a
r
c
t
a
n
x
=
x
−
1
3
x
3
+
o
(
x
3
)
arctanx=x-\\frac13x^3+o(x^3)
arctanx=x−31x3+o(x3)
a
r
c
t
a
n
x
arctanx
arctanx ~
x
x
x
x
−
a
r
c
t
a
n
x
x-arctanx
x−arctanx ~
1
3
x
3
\\frac13x^3
31x3
c
o
s
x
=
1
−
1
2
x
2
+
1
4
!
x
4
+
o
(
x
4
)
cosx=1-\\frac12x^2+\\frac14!x^4+o(x^4)
cosx=1−21x2+4!1x4+o(x4)
1
−
c
o
s
x
1-cosx
1−cosx ~
1
2
x
2
\\frac12x^2
21x2
l
n
(
1
+
x
)
=
x
−
1
2
x
2
+
o
(
x
2
)
ln(1+x)=x-\\frac12x^2+o(x^2)
ln(1+x)=x−21x2+o(x2)
l
n
(
1
+
x
)
ln(1+x)
ln(1+x) ~
x
x
x
l
n
(
1
+
x
)
−
x
ln(1+x)-x
ln(1+x)−x ~
−
1
2
x
2
-\\frac12x^2
−21x2
x
−
l
n
(
1
+
x
)
x-ln(1+x)
x−ln(1+x) ~
1
2
x
2
\\frac12x^2
21