[JSOI2016]灯塔/[POI2011]Lightning Conductor

Posted 2020-12-10 crashed

题目

点这里看题目。

分析

直接变换式子：

[egin{aligned} h_jle h_i+p_i-sqrt{|i-j|} & Rightarrow p_ile h_j+sqrt{|i-j|}-h_i & Rightarrow p_i=lceilmax{h_j+sqrt{|i-j|}-h_i} ceil & Rightarrow p_i=lceilmax{h_j+sqrt{|i-j|}} ceil-h_i end{aligned} ]

显然，(|i-j|)可以拆分成(i<j)和(i>j)两个部分，两个部分是相似的，因此我们可以只考虑(i<j)的情况。

考虑到(sqrt{n})的增长速度随(n)的增大而减小，因此会存在“后来的决策点超越前面的决策点”的情况，但绝不存在“前面的决策点超越后来的决策点”的情况。

设(f_i(n)=sqrt{n-i}+h_i)，那么我们就正在求解(max_{jle i}{f_j(i)})。

下图展示了样例中(f)函数的情况：

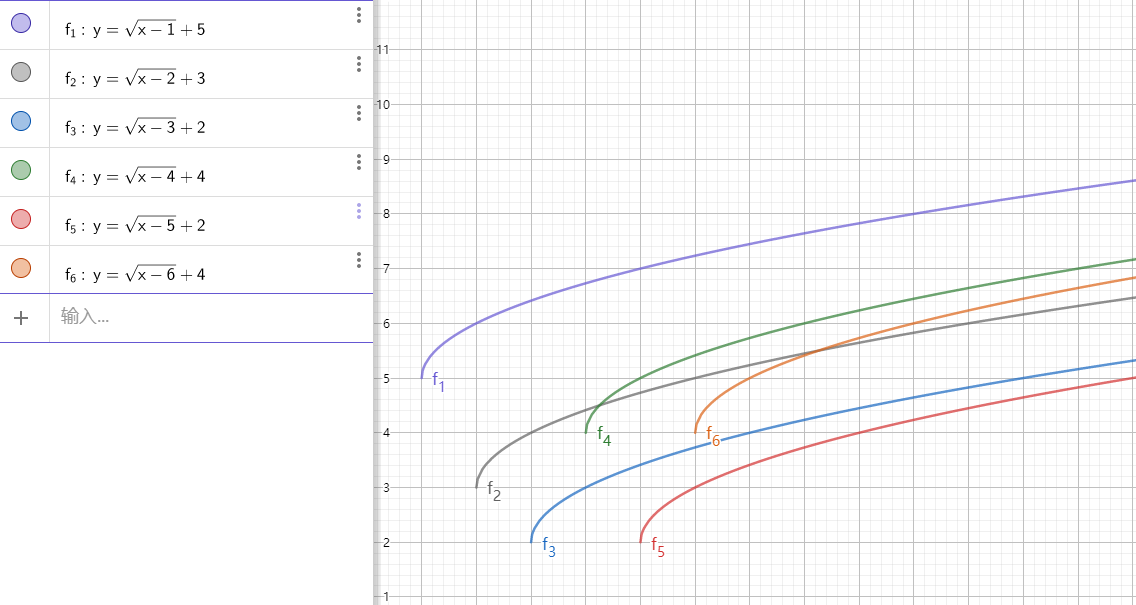
可以发现，两个(f)函数最多有一个交点。那么，对于两个函数，我们就可以二分出它们交点的(x)坐标（没有交点的，我们视为交点在无限远处）。那么，当扫描的下标(i)越过了交点之后，先来的决策点就会被踢出去，后来的决策点就比它更优。

样例中，(f_4)和(f_2)的交点的(x)坐标为(frac {17} 4)。这意味着，当(i<frac {17}4)时，(f_2)更优；否则(f_4)更优。

根据上述性质，每个决策点最多会被弹出一次。因此我们可以想到用队列维护决策点集合。对于每个决策点(k)，我们处理出它什么时候会被它后面一个决策点弹出去，记为(d_k)。在新扫描到一个位置的时候，我们先将这个位置从队尾加入到决策点集合，并把那些比它劣的决策点全部弹掉。然后再查询当前的答案，先把队头的变劣的决策点弹掉（惰性删除），然后再计算。

时间复杂度(O(nlog_2n))。

代码

#include <cmath>
#include <cstdio>
#include <algorithm>

const int MAXN = 5e5 + 5;

template<typename _T>
void read( _T &x )
{
	x = 0;char s = getchar();int f = 1;
	while( s > ‘9‘ || s < ‘0‘ ){if( s == ‘-‘ ) f = -1; s = getchar();}
	while( s >= ‘0‘ && s <= ‘9‘ ){x = ( x << 3 ) + ( x << 1 ) + ( s - ‘0‘ ), s = getchar();}
	x *= f;
}

template<typename _T>
void write( _T x )
{
	if( x < 0 ){ putchar( ‘-‘ ); x = ( ~ x ) + 1; }
	if( 9 < x ){ write( x / 10 ); }
	putchar( x % 10 + ‘0‘ );
}

template<typename _T>
_T MAX( const _T a, const _T b )
{
	return a > b ? a : b;
}

template<typename _T>
_T ABS( const _T a, const _T b )
{
	return a > b ? a : b;
}

int q[MAXN], dl[MAXN];
int H[MAXN], p1[MAXN], p2[MAXN];
int N;

double calc( const int j, const int i )	//j < i 
{
	return H[j] + sqrt( i - j );
}

int lb( const int a, const int b )	//a < b
{
	int l = b, r = N, mid, ret = r + 1;
	while( l <= r )
	{
		mid = l + r >> 1;
		if( calc( a, mid ) > calc( b, mid ) ) l = mid + 1;
		else ret = mid, r = mid - 1;
	}
	return ret;
}

void calc( int *P )
{
	int h = 1, t = 0;
	for( int i = 1 ; i <= N ; i ++ )
	{
		while( h < t && calc( q[t], dl[t - 1] ) < calc( i, dl[t - 1] ) ) t --;
		dl[t] = lb( q[t], i ); q[++ t] = i;
		while( h < t && i >= dl[h] ) h ++;
		P[i] = MAX( P[i], ( int ) ceil( calc( q[h], i ) ) );
	}
}

int main()
{
	read( N );
	for( int i = 1 ; i <= N ; i ++ ) read( H[i] );
	calc( p1 ); 
	std :: reverse( H + 1, H + 1 + N ); 
	calc( p2 );
	for( int i = 1 ; i <= N ; i ++ ) 
		write( MAX( MAX( p1[i], p2[N - i + 1] ) - H[N - i + 1], 0 ) ), putchar( ‘
‘ );
	return 0;
}