CodeForces #1 C. Ancient Berland Circus(两种解法...

Posted 2023-02-02 ling_xiao007

CodeForces #1 C. Ancient Berland Circus

【题目大意】

给出一个正N多边形的三个点，问其最小可能面积是多少。

【题解一】

首先给出CodeForce官方题解。在这，我也学习了。因为接下来的题解二代码我实在没有通过，虽然思路很清晰，但个别数据的gcd会计算崩溃。如果有人能帮我解决一下不胜感激。

点可以正N多边形的顶点,当且仅当每一对点的极坐标角度之差(从多边形中心)是2的倍数*π/ N。所有的点都应该在圆中心多边形。我们可以找到多边形的中心/圆心(但我们要避免这样一个问题,即作为一个和弦(比如说,(x1,y1)-(x2,y2))在两次从中心更大的角度,而不是从其他角度的cricle(x3,y3)]。有很多方法可以定位圆心,我使用的方法是建立中点垂直段(x1,y1)-(x2,y2)和(x2,y2)-(x3,y3)形成y = kx + b和发现他们的十字路口。公式y =kx+ b的缺点是，它不能使用如果线平行于y,可能的解决方案是所有点随机旋转角(使用公式x”= x * cos(a)-y * sin(a),y = y * cos(a)+ x * sin()),直到没有水平线。

　　中心的坐标是已知后,我们使用的量化函数atan2,它返回在右象限角:a[i]=atan2(y[i]-ycenter, x[i]-xcenter)

　　正多边形的面积随N的增加而增加,所以有可能只是在N遍历所有可能的值按升序,退出循环首先发现N是满足条件的。

　　如何满足条件？使用sin(x)是使它容易:sin(x)= 0,当x是2*π的倍数。因此,分属于常规正N多边形。sin( N * (a[i]-a[j]) /2 )=0。因为有限精度算法,fabs( sin( N *(a[i]-a[j]) /2 ) ) < eps。

 

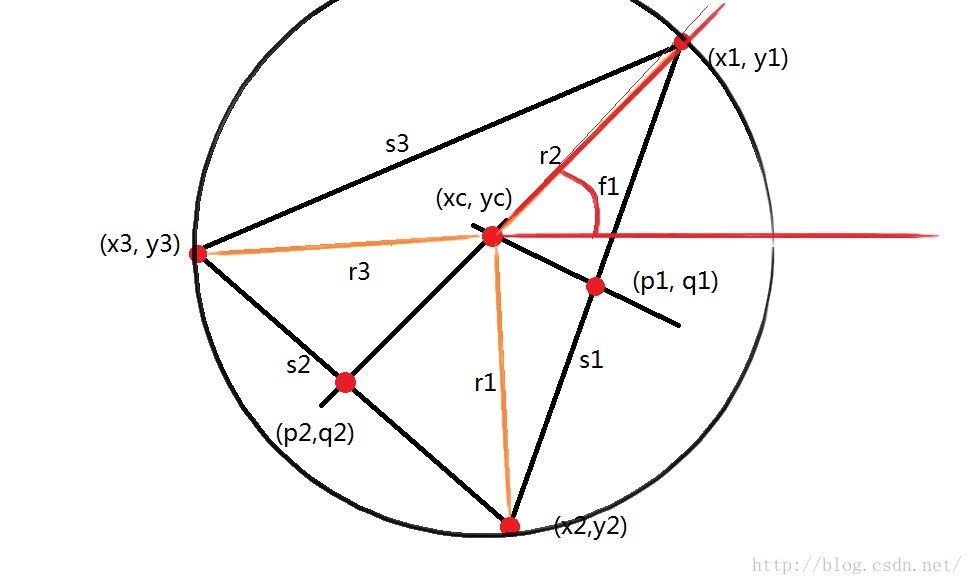
【题解一代码】

#ifdef O_O
#include "a.h"
#else
#include <cstdio>
#include <vector>
#include <list>
#include <map>
#include <set>
#include <deque>
#include <stack>
#include <bitset>
#include <algorithm>
#include <functional>
#include <numeric>
#include <utility>
#include <sstream>
#include <iostream>
#include <iomanip>
#include <cstring>
#include <cstdlib>
#include <cmath>
#include <ctime>
#endif


#define PB push_back
#define MP make_pair
typedef __int64 LL;
#define ALL(x)x.begin(),x.end()
#define FOR(a,b)for(int a=0;a<b;a++)
#define FORR(a,b,c)for(int a=b;a<=c;a++)
#define FOR1(a,b)for(int a=1;a<=b;a++)
#define CLR(a,b)memset(a,b,sizeof(a))
#define CPY(a,b)memcpy(a,b,sizeof(a))
#define tr(i,c)for(typeof((c).begin())i=(c).begin();i!=(c).end();i++)
#define M_PI acos(-1.0)
using namespace std;

LL n,m,a;

void rot(double &a,double &b,double cs,double sn) 
	double
	t = a*cs - b*sn;
	b = b*cs + a*sn;
	a = t;


int main()

	double x1,x2,x3,y1,y2,y3;
	cin >> x1 >> y1 >> x2 >> y2 >> x3 >> y3;

	double ma,mb,p1,p2;


	do <span style="white-space:pre">	</span>//旋转使之不水平
		double f = rand()%311/100.0;
		double c = cos(f), s= sin(f);

		rot(x1,y1,c,s);
		rot(x2,y2,c,s);
		rot(x3,y3,c,s);

		if(y1==y2 || y2==y3) continue;

		ma = -(x2-x1)/(y2-y1);
		mb = -(x3-x2)/(y3-y2);
		if(ma==mb) continue;
		goto ok;

	 while(1);
	ok:

	p1 = (x1+x2)/2;
	p2 = (x3+x2)/2;
	double q1 = (y1+y2)/2, q2 = (y3+y2)/2;
	
	double xc = (-q1+q2 + p1*ma - p2*mb)/(ma-mb);
	double yc = ma*(xc-p1) + q1;


	double
	s1 = hypot(x1-x2,y1-y2),
	s2 = hypot(x2-x3,y2-y3),
	s3 = hypot(x3-x1,y3-y1);//边长


	double
	r1 = hypot(x1-xc,y1-yc),
	r2 = hypot(x2-xc,y2-yc),
	r3 = hypot(x3-xc,y3-yc);//半径

	double
	f1 = atan2(x1-xc,y1-yc),
	f2 = atan2(x2-xc,y2-yc),
	f3 = atan2(x3-xc,y3-yc);//角

	double q = 180/M_PI;

	FORR(vv,3,100) 

		if(abs(sin( (f1-f3)*vv/2.0 ))>1e-4) continue;
		if(abs(sin( (f1-f2)*vv/2.0 ))>1e-4) continue;
		if(abs(sin( (f2-f3)*vv/2.0 ))>1e-4) continue;


		printf("%.8lf\\n",vv * r1*r1 * 0.5 * sin(2*M_PI / vv));
		return 0;	

	
	return 1;

	

【题解二】

网上比较清晰地思路

1.根据三点求3边a,b,c

2. 先求外接圆半径（一定存在）？

海伦公式 + 正弦定理   得

R = a * b * c / (4 * S)     S = sqrt(q * (q - a) * (q - b) * (q -c));  q = (a + b + c) / 2;

3. 求3个角？

余弦定理。三角求最大公约数就是  正多边形 每一份   最小的角度。

4.  求面积？

正弦定理。 S = R * R * sin(  angle ) / 2  * N （N = 2 * pi / angle);

【题解二代码】

#include <stdio.h>
#include <math.h>
#define feq(a, b) (fabs((a) - (b)) < 1e-2)
#define pi acos(-1.0)

double GetR(const double d[])

	double q = (d[0] + d[1] + d[2]) / 2;
	double S = sqrt (q * (q - d[0]) * (q - d[1]) * (q - d[2]));
	return d[0] * d[1] * d[2] / (4 * S);


double fgcd(double a, double b)  
     if (feq(a, 0)) return b;  
     if (feq(b, 0)) return a;  
     return fgcd(b, fmod(a, b));  
  


int main()

	double x[3], y[3], d[3];
	for (int i = 0; i < 3; i++)
		scanf("%lf%lf", &x[i], &y[i]);
	for (int i = 0; i < 3; i++)
		d[i] = sqrt(pow((x[i] - x[(i + 1) % 3]), 2) + pow((y[i] - y[(i + 1) % 3]), 2));
	double R = GetR(d);
	double angle[4];
	for (int i = 0; i < 2; i++)
		angle[i] = acos(1 - d[i] * d[i] / (2 * R * R));
	angle[2] = 2 * pi - angle[0] - angle[1]; 
	angle[3] = fgcd(angle[0], fgcd(angle[1], angle[2]));
	printf("%.8lf\\n",  R * R * sin(angle[3]) / 2 * (2 * pi / angle[3]) );

Notice：

CF测试给出下一组数据无法通过测试。具体我也不清楚怎么回事....

31.312532 151.532355
182.646053 56.534075
15.953947 127.065925