常见的七种排序算法(Java实现)
Posted 编程小栈
tags:
篇首语:本文由小常识网(cha138.com)小编为大家整理,主要介绍了常见的七种排序算法(Java实现)相关的知识,希望对你有一定的参考价值。
文章目录
一、算法的一些概念
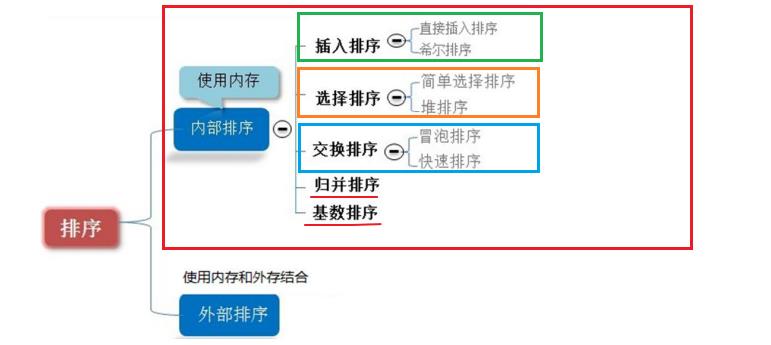
1.排序算法分类
排序也称排序算法(Sort Algorithm),排序是将一组数据,依指定的顺序进行排列的过程。
其可以分为两大类:
-
内部排序: 指将需要处理的所有数据都加载到内部存储器(内存)中进行排序。
-
外部排序法: 数据量过大,无法全部加载到内存中,需要借助外部存储(文件等)进行排序。
2.算法的时间复杂度
2.2.1度量一个程序(算法)执行时间的两种方法
- 运行后统计的方法 这种方法可行, 但是有两个问题:
一是要想对设计的算法的运行性能进行评测,需要实际运行该程序;
二是所 得时间的统计量依赖于计算机的硬件、软件等环境因素, 这种方式,要在同一台计算机的相同状态下运行,才能比较那个算法速度更快。
- 运行前估算的方法 通过分析某个算法的时间复杂度来判断哪个算法更优.
2.2.2时间频度
一个算法中的语句执行次数称为语句频度或时间频度。记为 T(n)。
一个算法花费的时间与算法中语句的执行次数成正比例,哪个算法中语句执行次数多,它花费时间就多。
2.3.3时间复杂度
- 一般情况下,算法中的基本操作语句的重复执行次数是问题规模 n 的某个函数,用 T(n)表示。**若有某个辅助函数 f(n),使得当 n 趋近于无穷大时,T(n) / f(n) 的极限值为不等于零的常数,**则称 f(n)是 T(n)的同数量级函数。 记作 T(n)=O( f(n) ),称O( f(n) ) 为算法的渐进时间复杂度,简称时间复杂度。
- T(n) 不同,但时间复杂度可能相同。 如:T(n)=n²+7n+6 与 T(n)=3n²+2n+2 它们的 T(n) 不同,但时间复杂度相同,都为 O(n²)。
- 计算时间复杂度的方法:
- 用常数 1 代替运行时间中的所有加法常数 : T(n)=n²+7n+6 => T(n)=n²+7n+1
- 修改后的运行次数函数中,只保留最高阶项 :T(n)=n²+7n+1 => T(n) = n²
- 去除最高阶项的系数 :T(n) = n² => T(n)= n² =>On²)
2.3.4常见的时间复杂度
- 常数阶 O(1)
- 对数阶 O(log2n)
- 线性阶 O(n)
- 线性对数阶 O(nlog2n)
- 平方阶 O(n^2)
- 立方阶 O(n^3)
- k 次方阶 O(n^k)
- 指数阶 O(2^n)
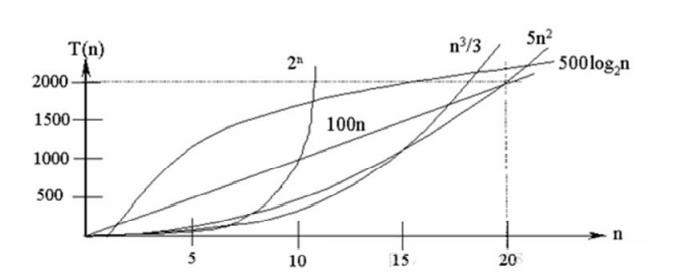
说明:
- 常见的算法时间复杂度由小到大依次为:
Ο(1)<Ο(log2n)<Ο(n)<Ο(nlog2n)<O(n^2) <O(n^3) < O(n^k) < O(2^n) ,
随着问题规模 n 的不断增大,上述时间复杂度不断增大,算法的执行效率越低
- 从图中可见,我们应该尽可能避免使用指数阶的算法
2.3.5平均时间复杂度和最坏时间复杂度
-
平均时间复杂度是指所有可能的输入实例均以等概率出现的情况下,该算法的运行时间。
-
最坏情况下的时间复杂度称最坏时间复杂度。一**般讨论的时间复杂度均是最坏情况下的时间复杂度。**这样做的 原因是:最坏情况下的时间复杂度是算法在任何输入实例上运行时间的界限,这就保证了算法的运行时间不会 比最坏情况更长。
-
平均时间复杂度和最坏时间复杂度是否一致,和算法有关(如图:)
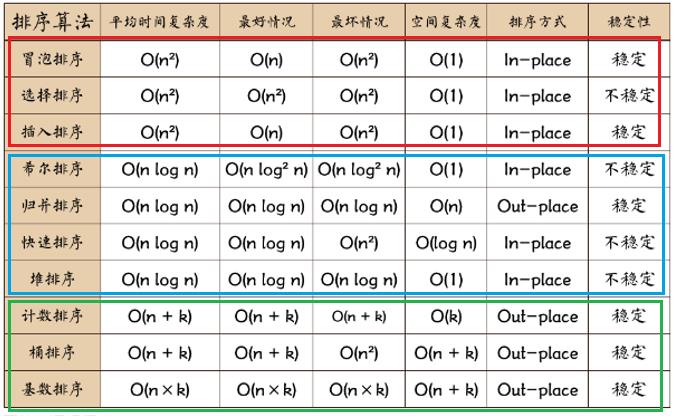
相关术语:
-
稳定:如果 a 原本在 b 前面,而 a=b,排序之后 a 仍然在 b 的前面;
-
不稳定:如果 a 原本在 b 的前面,而 a=b,排序之后 a 可能会出现在 b 的后面;
-
内排序:所有排序操作都在内存中完成;
-
外排序:由于数据太大,因此把数据放在磁盘中,而排序通过磁盘和内存的数据传输才能进行;
-
时间复杂度: 一个算法执行所耗费的时间。
-
空间复杂度:运行完一个程序所需内存的大小。
-
n: 数据规模
-
k: “桶”的个数
-
In-place: 不占用额外内存 10) Out-place: 占用额外内存
3.算法的空间复杂度
-
类似于时间复杂度的讨论,一个算法的空间复杂度(Space Complexity)定义为该算法所耗费的存储空间,它也是 问题规模 n 的函数。
-
空间复杂度(Space Complexity)是**对一个算法在运行过程中临时占用存储空间大小的量度。**有的算法需要占用的 临时工作单元数与解决问题的规模 n 有关,它随着 n 的增大而增大,当 n 较大时,将占用较多的存储单元,例如快速排序和归并排序算法, 基数排序就属于这种情况
-
在做算法分析时,主要讨论的是时间复杂度。从用户使用体验上看,更看重的程序执行的速度。一些缓存产品 (redis, memcache)和算法(基数排序)本质就是用空间换时间。
二、八种排序算法[Java代码]
1.冒泡排序
package com.ahcfl._06sort;
import java.time.LocalDateTime;
import java.util.Arrays;
/**
* @Author: ahcfl
* @Description: 冒泡排序 - 渐进时间复杂度(时间复杂度)O(n^2)
* 通过对待排序序列从前向后(从下标较小的元素开始),
* 依次比较相邻元素的值,若发现逆序则交换,使值较大的元素逐渐从前移向后部。
* 就象水底下的气泡一样逐渐向上冒。
*
* 优化:
* 因为排序的过程中,各元素不断接近自己的位置,如果一趟比较下来没有进行过交换,就说明序列有序,
* 因此要在排序过程中设置一个标志 flag 判断元素是否进行过交换。从而减少不必要的比较。
*/
public class BubbleSort
public static void main(String[] args)
//int[] array = 2, -3, 1, 8, 10, -1; // 执行5次
//-3, 1, 2, 8, -1, 10
// -3 1 2 -1 8 10
// -3 1 -1 2 8 10
// -3 -1 1 2 8 10
// -3 -1 1 2 8 10
/*加flag 标志位优化 一次循环比较不产生交换 说明已经排序完毕
* 没必要再执行不必要的比较
* */
//int[] array = 2, -3, 1, 8, 10, -1; // 执行4次
//int[] array = 1, 2, 3, 4, 5, 6; // 执行1次
/*测试8w条数据排序时间*/
int[] array = new int[80000];
for (int i = 0; i < 80000; i++)
array[i] = (int) (Math.random() * 80000);
System.out.println("排序前:" + LocalDateTime.now().toString());
bubbleSort(array); // 20s左右
System.out.print("排序后:" + LocalDateTime.now().toString());
private static void bubbleSort(int[] array)
int count = 0;
int temp = 0; // 中间变量
boolean flag = false; // 交换标志位
for (int j = 0; j < array.length - 1; j++)
for (int i = 0; i < array.length - 1 - j; i++)
if (array[i] > array[i + 1])
flag = true; // 产生了交换
temp = array[i];
array[i] = array[i + 1];
array[i + 1] = temp;
count++;
//System.out.println(j + "--> array = " + Arrays.toString(array));
if (!flag) // flag不变 退出
break;
else
flag = false; // 每次循环
System.out.println("count = " + count);
2.选择排序
package com.ahcfl._06sort;
import java.time.LocalDateTime;
import java.util.Arrays;
/**
* @Author: ahcfl
* @Description: 选择排序 (时间复杂度)O(n^2)
* 从排序的数据中,按指定的规则选出(选最小值或最大值)某一元素,再依规定交换位置
* 第一次从 arr[0]~arr[n-1]中选取最小值,与 arr[0]交换,
* 第二次从 arr[1]~arr[n-1]中选取最小值,与 arr[1]交换,
* 第三次从 arr[2]~arr[n-1]中选取最小值,与 arr[2]交换,…,
* 第 i 次从 arr[i-1]~arr[n-1]中选取最小值,与 arr[i-1]交换,…,
* 第 n-1 次从 arr[n-2]~arr[n-1]中选取最小值,与 arr[n-2]交换,
* 总共通过 n-1 次,得到一个按排序码从小到大排列的有序序列
*/
public class SelectSort
public static void main(String[] args)
//int[] array = 2, -3, 1, 8, 10, -5;
/*int minIndex = 0; // 初始最小索引
int minVal = array[0]; // 假定最小值
for (int j = 1; j < array.length - 1; j++)
if (minVal > array[j])
minVal = array[j]; // 发现比假定值小的交换
minIndex = j; // 这里的j 表示最小值的索引
array[minIndex] = array[0]; // 0索引位置值与最小值交换
array[0] = minVal; // 把一次循环比较后的最小值放到0索引位
System.out.println("array = " + Arrays.toString(array));*/
// 测试8w条数据排序时间
int[] array = new int[80000];
for (int i = 0; i < 80000; i++)
array[i] = (int) (Math.random() * 80000);
System.out.println("排序前:" + LocalDateTime.now().toString());
selectSort(array); // 3s
System.out.println("排序后:" + LocalDateTime.now().toString());
private static void selectSort(int[] array)
for (int i = 0; i < array.length; i++)
int minIndex = i; // 初始最小索引
int minVal = array[i]; // 假定最小值
for (int j = 1 + i; j < array.length; j++)
if (minVal > array[j])
minVal = array[j]; // 发现比假定值小的交换
minIndex = j; // 这里的j 表示最小值的索引
if (minIndex != i)
array[minIndex] = array[i]; // 0索引位置值与最小值交换
array[i] = minVal; // 把一次循环比较后的最小值放到0索引位
//System.out.println(i + "--> array = " + Arrays.toString(array));
3.插入排序
package com.ahcfl._06sort;
import java.time.LocalDateTime;
import java.util.Arrays;
/**
* @Author: ahcfl
* @Date: 2021年08月14日 14:16
* @Description: 插入排序 时间复杂度 O(n^2)
* 把 n 个待排序的元素 【看成为一个有序表和一个无序表】,
* 开始时有序表中只包含一个元素,无序表中包含有 n-1 个元素,
* 排序过程中每次从无序表中取出第一个元素,【把它的排序码依次与有序表元素的排序码进行比较】,
* 将它插入到有序表中的适当位置,使之成为新的有序表。
*/
public class InsertSort
public static void main(String[] args)
//int[] array = 2, -3, 8, 1, 10, -5; // 升序排列
/*// 插入第一个数
int insertVal = array[1]; // 存放待插入值
int insertIndex = 1 - 1; // 存放最终插入值前一个值索引
// 索引>=0退出 防止索引越界
// insertVal < array[insertIndex] 待插入的数还没倒找插入位置
while (insertIndex >= 0 && insertVal < array[insertIndex])
array[insertIndex + 1] = array[insertIndex];
insertIndex--;
array[insertIndex+1] = insertVal;
System.out.println("array = " + Arrays.toString(array));*/
// 测试8w条数据排序时间
int[] array = new int[80000];
for (int i = 0; i < 80000; i++)
array[i] = (int) (Math.random() * 80000);
System.out.println("排序前:" + LocalDateTime.now().toString());
insertSort(array); // 1s
System.out.println("排序后:" + LocalDateTime.now().toString());
private static void insertSort(int[] array)
int insertVal = 0; // 存放待插入值
int insertIndex = 0; // 存放最终插入值前一个值索引
for (int i = 1; i < array.length; i++)
insertVal = array[i];
insertIndex = i - 1;
// 索引>=0退出 防止索引越界
// insertVal < array[insertIndex] 待插入的数还没倒找插入位置 进入循环
while (insertIndex >= 0 && insertVal < array[insertIndex])
array[insertIndex + 1] = array[insertIndex]; // 比待插入值大的前移
insertIndex--; // 再找前一个值 进行 判断&&比较 直到索引为0退出
if (insertIndex + 1 != i)
array[insertIndex + 1] = insertVal;
//System.out.println(i + "--> array = " + Arrays.toString(array));
4.希尔排序
package com.ahcfl._06sort;
import java.time.LocalDateTime;
import java.util.Arrays;
/**
* @Author: ahcfl
* @Date: 2021年08月14日 15:35
* @Description: 希尔排序 时间复杂度O(nlogn) 分组并进行插入排序 缩小增量排序(缩小分组的增量)
* 希尔排序是希尔(Donald Shell)于 1959 年提出的一种排序算法。
* 希尔排序也是一种插入排序,它是简单插入排序经过改进之后的一个更高效的版本,也称为缩小增量排序。
*
* 希尔排序是把记录按下标的一定增量分组,对每组使用直接插入排序算法排序;随着增量逐渐减少,每组包含
* 的关键词越来越多,当增量减至 1 时,整个文件恰被分成一组,算法便终止。
*/
public class ShellSort
public static void main(String[] args)
//int[] array = 8, 9, 2, 7, 3, 4, 1, 5, 6, 0;
//shellMind(array);
// 测试8w条数据排序时间
int[] array = new int[80000];
for (int i = 0; i < 80000; i++)
array[i] = (int) (Math.random() * 80000);
System.out.println("排序前:" + LocalDateTime.now().toString());
//swapShellSort(array); // 插入时采用交换法 6s
gressionShellSort(array); // 插入时采用移位法 40ms
System.out.println("排序后:" + LocalDateTime.now().toString());
private static void swapShellSort(int[] array)
int temp = 0;
for (int gap = array.length / 2; gap > 0; gap /= 2)
for (int i = gap; i < array.length; i++)
// 遍历各组中所有的元素 gap组, 每组元素的步长gap
for (int j = i - gap; j >= 0; j -= gap)
// 如果当前元素 > 加上步长元素 交换(大的值向后移动)
if (array[j] > array[j + gap])
temp = array[j];
array[j] = array[j + gap];
array[j + gap] = temp;
//System.out.println(gap + "--> array = " + Arrays.toString(array));
private static void gressionShellSort(int[] array)
for (int gap = array.length / 2; gap > 0; gap /= 2)
for (int i = gap; i < array.length; i++)
// 遍历各组中所有的元素 gap组, 每组元素的步长gap
int j = i;
int temp = array[i]; //待插入的值
if (array[j] < array[j - gap]) // 待插入值如果小于前面步长值 进行插入,反之,进行下一个
while (j - gap >= 0 && temp < array[j - gap]) // 循环确定最终插入位置
array[j] = array[j - gap];
j -= gap;
// 当索引小于0退出, 或者temp大于前面的值退出
if (j != i)
array[j] = temp; // 插入数值
//System.out.println(gap + "--> array = " + Arrays.toString(array));
private static void shellMind(int[] array)
// 交换
int temp = 0;
for (int i = 5; i < array.length; i++)
for (int j = i - 5; j >= 0; j -= 5)
if (array[j] > array[j + 5])
temp = array[j];
array[j] = array[j + 5];
array[j + 5] = temp;
System.out.println("array = " + Arrays.toString(array));
for (int i = 2; i < array.length; i++)
for (int j = i - 2; j >= 0; j -= 2)
if (array[j] > array[j + 2])
temp = array[j];
array[j] = array[j + 2];
array[j + 2] = temp;
System.out.println("array = " + Arrays.toString(array));
for (int i = 1; i < array.length; i++)
for (int j = i - 1; j >= 0; j -= 1)
if (array[j] > array[j + 1])
temp = array[j];
array[j] = array[j + 1];
array[j + 1] = temp;
System.out.println("array = " + Arrays.toString(array以上是关于常见的七种排序算法(Java实现)的主要内容,如果未能解决你的问题,请参考以下文章