Java集合与数据结构 排序
Posted 头发都哪去了
tags:
篇首语:本文由小常识网(cha138.com)小编为大家整理,主要介绍了Java集合与数据结构 排序相关的知识,希望对你有一定的参考价值。
Java集合与数据结构 排序
概念
排序,就是使一串记录,按照其中的某个或某些关键字的大小,递增或递减的排列起来的操作。
平时的上下文中,如果提到排序,通常指的是排升序(非降序)。
通常意义上的排序,都是指的原地排序(in place sort)。
稳定性: 两个相等的数据,如果经过排序后,排序算法能保证其相对位置不发生变化,则我们称该算法是具备稳定性的排序算法。

插入排序
直接插入排序
整个区间被分为
- 有序区间
- 无序区间
每次选择无序区间的第一个元素,在有序区间内选择合适的位置插入
代码实现
逻辑代码:
public class InsertSort
public static void insertSort(int[] array)
for (int i = 1; i < array.length; i++)
int temp = array[i];
int j = i-1;
for (; j >= 0; j--)
if (array[j] > temp)
array[j+1] = array[j];
else
break;
array[j+1] = temp;
调试代码:
public class TestDemo
public static void main(String[] args)
int[] array = 10,3,2,7,19,78,65,127;
System.out.println("排序前:" + Arrays.toString(array));
InsertSort.insertSort(array);
System.out.println("排序后:" + Arrays.toString(array));
该代码的执行结果为:
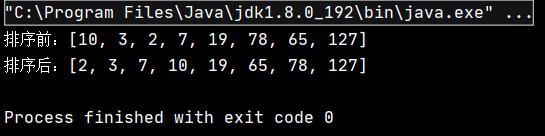
可见,实现了对原数组的升序排序。
性能分析
时间复杂度:
最好情况:O(n)【数据有序】
平均情况:O(n2)
最坏情况:O(n2)【数据逆序】
空间复杂度:O(1)
稳定性:稳定
对于直接插入排序:越有序越快。另外,直接插入排序会用在一些排序的优化上。
希尔排序
希尔排序法又称缩小增量法。希尔排序法的基本思想是:先选定一个整数,把待排序文件中所有记录分成个组,所有距离为的记录分在同一组内,并对每一组内的记录进行排序。然后,取,重复上述分组和排序的工作。当到达=1时, 所有记录在统一组内排好序。
- 希尔排序是对直接插入排序的优化。
- 当gap > 1时都是预排序,目的是让数组更接近于有序。当gap == 1时,数组已经接近有序的了,这样就会很快。这样整体而言,可以达到优化的效果。我们实现后可以进行性能测试的对比。
代码实现
逻辑代码:
public class ShellSort
public static void shell(int[] array,int gap)
for (int i = gap; i < array.length; i = i + gap)
int temp = array[i];
int j = i-gap;
for (; j >= 0; j = j-gap)
if (array[j] > temp)
array[j+gap] = array[j];
else
break;
array[j+gap] = temp;
public static void shellSort(int[] array)
int[] drr = 5,3,1;//增量数组-->没有明确的规定,但保证为素数的增量序列
for (int i = 0; i < drr.length; i++)
shell(array,drr[i]);
测试代码:
public class TestDemo
public static void main(String[] args)
int[] array = 10,3,2,7,19,78,65,127;
System.out.println("排序前:" + Arrays.toString(array));
ShellSort.shellSort(array);
System.out.println("排序后:" + Arrays.toString(array));
该代码的执行结果为:
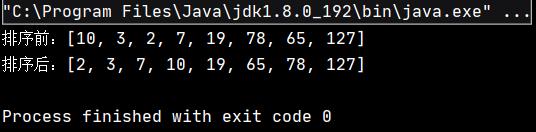
可见,实现了对原数组的升序排序。
性能分析
时间复杂度:
最好情况:O(n)【数据有序】
平均情况:O(n1.3)
最坏情况: O(n2) 【比较难构造】
空间复杂度:O(1)
稳定性:不稳定
选择排序
直接选择排序
每一次从无序区间选出最大(或最小)的一个元素,存放在无序区间的最后(或最前),直到全部待排序的数据元素排完 。
代码实现
逻辑代码:
public class SelectSort
public static void selectSort(int[] array)
for (int i = 0; i < array.length-1; i++)
for (int j = i+1; j < array.length; j++)
if (array[i] > array[j])
int temp = array[j];
array[j] = array[i];
array[i] = temp;
测试代码:
public class TestDemo
public static void main(String[] args)
int[] array = 10,3,2,7,19,78,65,127;
System.out.println("排序前:" + Arrays.toString(array));
SelectSort.selectSort(array);
System.out.println("排序后:" + Arrays.toString(array));
该代码的执行结果为:
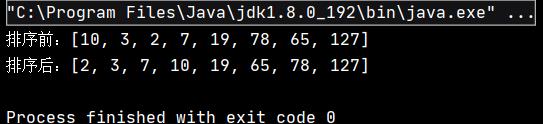
可见,实现了对原数组的升序排序。
性能分析
时间复杂度 : 不管是最好情况还是最坏情况都是O(n2) 【数据不敏感】
空间复杂度: O(1)
稳定性:不稳定
堆排序
基本原理也是选择排序,只是不在使用遍历的方式查找无序区间的最大的数,而是通过堆来选择无序区间的最大的数。
注意:排升序要建大堆;排降序要建小堆。
代码实现
逻辑代码:
public class HeapSort
public static void heapSort(int[] array)
PriorityQueue<Integer> priorityQueue = new PriorityQueue<>(new Comparator<Integer>()
@Override
public int compare(Integer o1, Integer o2)
return o1-o2;
);
for (int i = 0; i < array.length; i++)
priorityQueue.add(array[i]);
for (int i = 0; i < array.length; i++)
array[i] = priorityQueue.poll();
测试代码:
public class TestDemo
public static void main(String[] args)
int[] array = 10,3,2,7,19,78,65,127;
System.out.println("排序前:" + Arrays.toString(array));
HeapSort.heapSort(array);
System.out.println("排序后:" + Arrays.toString(array));
该代码的执行结果为:

可见,实现了对原数组的升序排序。
性能分析
时间复杂度:不管是最好的情况还是最坏的情况都是O(n * log(n)) 。
空间复杂度:O(1)。
稳定性:不稳定
交换排序
冒泡排序
在无序区间,通过相邻数的比较,将最大的数冒泡到无序区间的最后,持续这个过程,直到数组整体有序。
代码实现
逻辑代码:
public class BubbleBort
public static void bubbleBort(int[] array)
for (int i = 0; i < array.length-1; i++)
for (int j = 0; j < array.length-i-1; j++)
if (array[j] > array[j+1])
int temp = array[j];
array[j] = array[j+1];
array[j+1] = temp;
测试代码:
public class TestDemo
public static void main(String[] args)
int[] array = 10,3,2,7,19,78,65,127;
System.out.println("排序前:" + Arrays.toString(array));
BubbleBort.bubbleBort(array);
System.out.println("排序后:" + Arrays.toString(array));
该代码的执行结果为:

可见,实现了对原数组的升序排序。
性能分析
时间复杂度:
最好情况:O(n)【数据有序】
平均情况:O(n2)
最坏情况: O(n2) 【数据逆序】
空间复杂度:O(1)。
稳定性:稳定
快速排序
- 从待排序区间选择一个数,作为基准值(pivot);
- Partition: 遍历整个待排序区间,将比基准值小的(可以包含相等的)放到基准值的左边,将比基准值大的(可以包含相等的)放到基准值的右边;
- 采用分治思想,对左右两个小区间按照同样的方式处理,直到小区间的长度 = 1,代表已经有序,或者小区间的长度 = 0,代表没有数据。
代码实现
逻辑代码:
public class QuickSort
public static void quick(int[] array,int low,int high)
if (low < high)
int piv = piovt(array,low,high);//找基准
quick(array,low,piv-1);
quick(array,piv+1,high);
private static int piovt(int[] array,int start,int end)
int temp = array[start];
while (start < end)
while (start < end && array[end] >= temp)
end--;
array[start] = array[end];
while (start < end && array[start] < temp)
start++;
array[end] = array[start];
array[start] = temp;
return start;
public static void quickSort(int[] array)
quick(array,0,array.length-1);
测试代码:
public class TestDemo
public static void main(String[] args)
int[] array = 10,3,2,7,19,78,65,127;
System.out.println("排序前:" + Arrays.toString(array));
QuickSort.quickSort(array);
System.out.println("排序后:" + Arrays.toString(array));
该代码的执行结果为:

可见,实现了对原数组的升序排序。
性能分析
时间复杂度:
最好情况:O(n * log(n))
平均情况:O(n * log(n))
最坏情况: O(n2)
空间复杂度:
最好情况:O(log(n))
平均情况:O(log(n))
最坏情况:O(n)
稳定性:不稳定
非递归实现快速排序
代码实现
逻辑代码:
/**
* 非递归实现快速排序
*/
public class QuickSortNor
public static void quickSortNor(int[] array)
int low = 0;
int high = array.length - 1;
int piv = piovt(array, low, high);
Stack<Integer> stack = new Stack<>();
if (piv > low + 1)
stack.push(low);
stack.push(piv - 1);
if (piv < high - 1)
stack.push(piv + 1);
stack.push(high);
while (!stack.isEmpty())
high = stack.pop();
low = stack.pop();
piv = piovt(array, low, high);
if (piv > low + 1)
stack.push(low);
stack.push(piv - 1);
if (piv < high - 1)
stack.push(piv + 1);
stack.push(high);
private static int piovt(int[] array, int start, int end)
int temp = array[start];
while (start < end)
while (start < end && array[end] >= temp)
end--;
array[start] = array[end];
while (start < end && array[start] < temp)
start++;
array[end] = array[start];
array[start] = temp;
return start;
测试代码:
public class TestDemo
public static void main(String以上是关于Java集合与数据结构 排序的主要内容,如果未能解决你的问题,请参考以下文章
