Hyrax: Doubly-efficient zkSNARKs without trusted setup学习笔记

Posted 2023-01-16 mutourend

1. 引言

Wahby等人2018年论文《Doubly-efficient zkSNARKs without trusted setup》。
代码实现参见：
https://github.com/hyraxZK
视频解说参见：
https://www.youtube.com/watch?v=ScY9Z5tZZKU
https://www.youtube.com/watch?v=yq2AfLlMww0

论文要点：

• 基于standard cryptographic assumption，无需trusted setup，对Prover和Verifier均具有low communication complexity和low concrete cost的zkSNARKs for NP。
• Communication为$\Theta (d\cdot \log G+\sqrt{n_w})$，其中$d,G$分别为verifying circuit的depth和width，$w_n$为witness size。
• 当用于batched statements或者data-parallel statements时，Prover的runtime为linear in the verifying circuit size，Verifier的runtime为sub-linear in the verifying circuit size。两者均具有good constants。
• 通过使用a new commitment scheme for multilinear polynomials，witness-related communication可reduced，但verifier time会增加。
• 需要在setup、complexity assumptions、proof size和computational cost之间进行取舍平衡。
• 基于discrete log assumption，采用Fiat-Shamir heuristic 实现了zkSNARK in the random oracle model，本文称之为Hyrax。
• 将Hyrax与5种系统（BCCGP-sqrt, Bulletproofs, Ligero, ZKB++和libSTARK）进行了对比。对于modest problem sizes，Hyrax具有smaller proofs，most computationally costly baseline，prover和verifier速度快于5种系统中的3种。

其中5种方案分别为：

• BCCGP-sqrt：来源于Bootle等人2016年论文《Efficient zero-knowledge arguments for arithmetic circuits in the discrete log setting》。（在Groth [57] 和 Bayer and Groth [6] 的基础上，基于hardness of discrete logarithm，提供了2种ZK argument for Arithmetic Circuit $C$’s satisfiability。第一种proof size为$O(\sqrt{M})$，具有quasi-linear prover and verifier runtime for an AC with $M$ multiplications；第二种proof size为$O(\log M)$ at the cost of concretely longer prover and verifier runtimes。）
• Bulletproofs：来源于Bünz等人2018年论文《Bulletproofs: Efficient range proofs for confidential transactions》。（在BCCGP-sqrt的基础上进行改进，reduce proof size and runtimes in the log scheme $\approx 3\times$）
• Ligero：来源于Ames等人2017年论文《Ligero: Lightweight sublinear arguments without a trusted setup》。（在ZKB++的基础上，使用了更成熟的secure computation protocol，可prove an Arithmetic Circuit $C$’s satisfiability with proof size $O(\sqrt{|C|})$，prover和verifier work为quasi-linear in $|C|$。）
• ZKB++：来源于Chase等人2017年论文《Post-quantum zero-knowledge and signatures from symmetric-key primitives》。（将a secure multi-party computation protocol into a ZK argument，为a ZK argument system for Boolean circuits with no trusted setup from collision-resistant hashes。concretely inexpensive for small circuits，但是costs scale linearly with circuit size。）
• libSTARK：来源于Ben-Sasson等人2018年论文《Scalable, transparent, and post-quantum secure computational integrity》。（zkSTARKs不需要trusted setup，no public-key cryptography，但是其soundness 基于non-standard conjecture related to Reed-Solomon codes。Both proof size and verifier runtime are logarithmic in circuit size (hundreds of kilobytes and tens of milliseconds, respectively, in practice), and prover runtime is quasi-linear。）

1.1 zero-knowledge proof

A zero-knowledge proof用于convince a verifier of a statement while revealing nothing but its own validity。

• zero-knowledge proof概念由Goldwasser等人在1989年论文《The knowledge complexity of interactive proof systems》中首次提出。

• Ben-Or等人1990年论文《Everything provable is provable in zero-knowledge》中指出：
  any problem solvable by an interactive proof (IP) is also solvable by a computational zero-knowledge proof or pefect zero-knowledge argument。
  也就是说，given an interactive proof for any NP-complete problem, one can construct zero-knowledge proofs or arguments for any NP statement。

1.2 本文算法性能表现

本文主要关注的点有：

• proof应为succinct，sub-linear in the size of the statement and the witness to the statement’s validity；
• verifier应run in time linear in input plus proof size；
• prover，given a witness to the statement’s validity，应run in time linear in the cost of the NP verification procedure；
• 整个scheme应既不需要trusted setup，也不需要common reference string；
• soundness and zero-knowledge应为statistical或者基于standard cryptographic assumptions。实际上，security in the random oracle model就足够。

本文主要做了以下两方面的改进：

• 1）在verification procedure中整合了multi-commitment scheme和Schnorr-style proof。
• 2）设计了一种新的witness commitment scheme，可产生a succinct argument and asymptotically reducing the verifier’s cost associated with the witness。

具体的性能表现为：

1.3 Polynomial commitment scheme

• Polynomial commitment scheme 首次由Kate等人2010年论文《Constant-size commitments to polynomials and their applications》中提出，并基于pairing assumption 构建了单变量polynomial commitment。
• Papamanthou等人2013年论文《Signatures of correct computation》、Zhang等人2017年论文《vSQL: veriifying arbitrary SQL queries over dynamic outsourced databases》、Zhang等人2017年论文《A zero-knowledge version of vSQL》、Zhang等人2018年论文《vRAM: Faster verifiable RAM with program-independent preprocessing》等论文中，将其扩展为多变量polynomial commitment。
• Libert等人2016年论文《Functional Commitment Schemes: From Polynomial Commitments to Pairing-Based Accumulators》中构建了Functional Commitment (FC) for a linear functions based on constant-size assumptions in composite order groups endowed with a bilinear map。设置其challenge为$\vec{x}=(1,x,\cdots ,x^{n-1})$即可实现polynomial commitment。
• Fujisaki等人1997年论文《Statistical zero knowledge protocols to prove modular polynomial relations》中 give a construction for polynomial evaluation based on the RSA problem that can be immediately adapted to polynomial commitment。
• Bootle等人2017年论文《Linear-time zero-knowledge proofs for arithmetic circuit satisfiability》 和 Bootle等人2018年论文《Efficient batch zero knowledge arguments for low degree polynomials》中基于discrete log assumption构建了单变量polynomial commitment。本文主要在此基础上，将其扩展为了multilinear polynomials。同时，Bootle等人2018年论文《Efficient batch zero knowledge arguments for low degree polynomials》中还 give a framework for expressing simple relations between commitments and field elements。

1.4 一些定义

• Arithmetic circuit (AC) $C$：
  由加法门和乘法门组成，每个门最多由2个输入fan-in，所有计算基于finite field $\mathbb{F}$。$C$为分层设计，具有depth $d$，input $\vec{x}$ with length $|x|$。
  目的是evaluate $C$ on input $\vec{x}$。在interactive proof or argument中，prover发送$y$，声称$y=C(\vec{x})$并提供相应的证明。
  本文的目的是为这种arithmetic circuit satisfiability problem提供efficient protocol。
  Let $C(\cdot ,\cdot )$为layered arithmetic circuit of fan-in two。已知输入$\vec{x}$和输出$y$，目的是确认是否存在 witness $\vec{w}$，使得 $C(\vec{x},\vec{w})=y$ 成立。相应的witness relation可表示为：$R_{(}\vec{x},y)=\vec{w}:C(\vec{x},\vec{w})=y$。

• Interactive arguments and proofs：
  

• Zero-knowledge (ZK)：
  

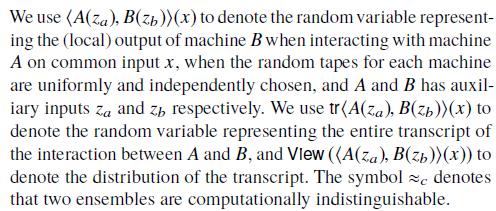
• Witness-extended emulation：
  
  

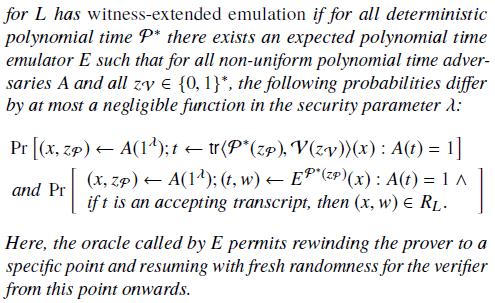
• Generalized special soundness：
  

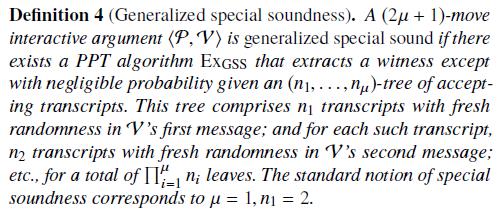
• Collection of non-interactive commitment：
  

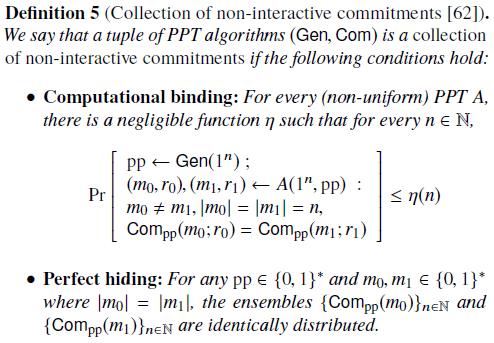
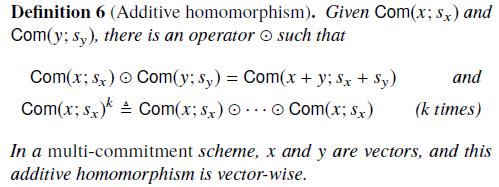
• Additive homomorphism加法同态属性：
  

2. Arithmetic circuit evaluation problem

主要的研究有：（Arithmetic circuit $C$ with depth $d$, input $x$, output $y$。）

• 【54】Goldwasser等人2015年论文《Delegating computation: Interactive proofs for muggles》中主要针对boolean circuit with depth $d$ and input length $n$。其Verifier runs in time $n\cdot poly(d,\log (n))$ and space $O(\log (n))$，communication complexity为$poly(d,\log (n))$，Prover runs in time $poly(n)$。
• 【37】【107】Cormode等人2012年论文《Practical verified computation with streaming interactive proofs》、Vu等人2013年论文《A hybrid architecture for interactive verifiable computation》中在【54】的基础上进行了改进，giving $O(|C|\log |C|)$ prover and $O(|x|+|y|+d\log |C|)$ verifier runtimes, for AC $C$ with depth $d$, input $x$, and output $y$。
• 【102】Thaler 2013年论文《Time-optimal interactive proofs for circuit evaluation》，针对$C$ 为data parallel，即包含$N$个相同的sub-computations run on different inputs，可称其为sub-AC of $C$（sub-AC的width为 G

  以上是关于Hyrax: Doubly-efficient zkSNARKs without trusted setup学习笔记的主要内容，如果未能解决你的问题，请参考以下文章

  sum-check protocol合集

  sum-check protocol合集

  SNARK原理示例

  三个正数 x、y、z 的组合，使得 x + y、x - y、y + z、y - z、x + z 和 x - z 是完全平方

  R计算Z分数（Z-Scores）

  z = z*z + c的分型图如何画