经典问题LCS（最大公共子串问题）C代码实现

Posted 2022-08-12 createchance

LCS（Longest Commons Sequence）问题，也就是最长公共子串问题，是一个经典的算法问题。该问题在很多地方都会遇到。关于这个问题的解决方案，目前最佳的实现就是使用动态规划的方式进行实现，关于使用动态规划设计这个问题算法的过程，这里就不再描述了，网上有很多的描述，整理如下：
wiki百科中的描述：https://en.wikipedia.org/wiki/Longest_common_subsequence_problem
UCI的《算法导论》课程线上教案：https://www.ics.uci.edu/~eppstein/161/960229.html
国立清华大学（台湾）线上教案：http://www.csie.ntnu.edu.tw/~u91029/LongestCommonSubsequence.html
等等，线上的资料太多了，我就不再重复造车轮了，建议大家吧前面几个链接中的资料好好看一下。下面是北京大学曲婉玲教授等编写的《算法设计与分析》第二版中的LCS描述截图：




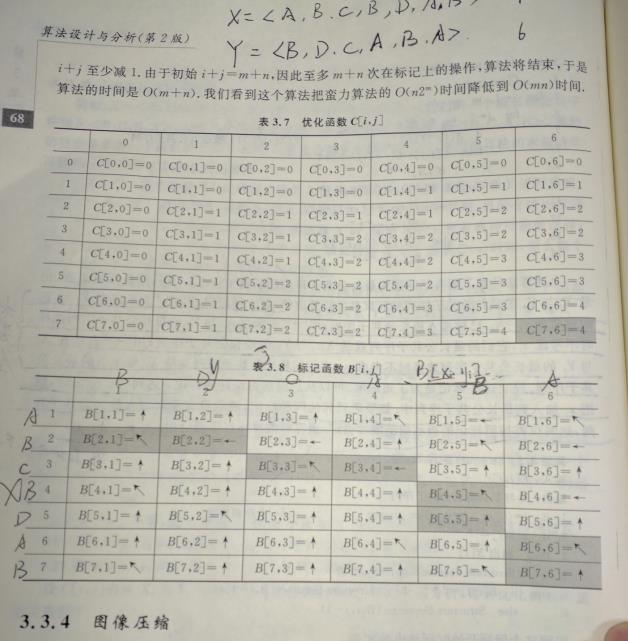
下面我给出一个使用C语言实现的LCS，使用上图中的伪代码方法：

/*************************************************************************
    > File Name: lcs.c
    > Author: Baniel Gao
    > Mail: [e-mail] 
    > Created Time: Tue 29 Nov 2016 01:53:13 PM CST
 ************************************************************************/

#include <stdio.h>
#include <string.h>

// 追踪标记
// 这里的实现不是很好，但是C语言二维数据指针操作真是的很恶心，所以出此下策
char tracker[100][100];

void print_lcs_by_tracker(char* str1, int m, int n)

    if (m == 0 || n == 0) 
        return;
    

    if (tracker[m - 1][n - 1] == '\\\\') 
        print_lcs_by_tracker(str1, m - 1, n - 1);
        printf("%c", str1[m - 1]);
     else if(tracker[m - 1][n - 1] == '|') 
        print_lcs_by_tracker(str1, m - 1, n);
     else 
        print_lcs_by_tracker(str1, m, n - 1);
    


void lcs(char* str1, char* str2, int m, int n)

    int len = 0;
    int len_arr[m + 1][n + 1];

    for (int i = 0; i <= m; i++)
    
        len_arr[i][0] = 0;
    

    for (int i = 0; i <= n; i++)
    
        len_arr[0][i] = 0;
    

    for (int i = 0; i < m; i++)
    
        for (int j = 0; j < n; j++)
        
            if (str1[i] == str2[j]) 
                len_arr[i + 1][j + 1] = len_arr[i][j] + 1;
                tracker[i][j] = '\\\\';
             else if (len_arr[i][j + 1] >= len_arr[i + 1][j]) 
                len_arr[i + 1][j + 1] = len_arr[i][j + 1];
                tracker[i][j] = '|';
             else 
                len_arr[i + 1][j + 1] = len_arr[i + 1][j];
                tracker[i][j] = '-';
            
        
    

    printf("Length array is: \\n");
    for (int i = 0; i <= n; i++) 
        printf("\\t%d", i);
    
    printf("\\n");
    for (int i = 0; i <= n; i++) 
        printf("\\t___", i);
    
    printf("\\n");
    for (int i = 0; i <= m; i++) 
        printf("%d\\t|", i);
        for (int j = 0; j <= n; j++) 
            printf("%d\\t", len_arr[i][j]);
        
        printf("\\n");
    

    printf("Tracker is: \\n");
    for (int i = 1; i <= n; i++) 
        printf("\\t%d", i);
    
    printf("\\n");
    for (int i = 1; i <= n; i++) 
        printf("\\t___", i);
    
    printf("\\n");
    for (int i = 0; i < m; i++) 
        printf("%d\\t|", i + 1);
        for (int j = 0; j < n; j++) 
            printf("%c\\t", tracker[i][j]);
        
        printf("\\n");
    

    printf("Len of LCS is: %d. \\n", len_arr[m][n]);
    printf("LCS is: ");
    print_lcs_by_tracker(str1, m, n);
    printf("\\n");


int main(int argc, char *argv[])

    int lcs_len = 0;

    if (argc != 3)
    
        printf("Usage: %s <string1> <string2> \\n", argv[0]);
        return -1;
    
    char* x = argv[1];
    char* y = argv[2];

    int m = strlen(x);
    int n = strlen(y);

    lcs(x, y, m, n);

    return 0;

运行截图：